In some cases, the geometric figure representing a certain situation does not have any special characteristics. However, dividing up this figure reveals simpler geometric figures “inside” that do have useful, particular properties. It then becomes easier to find the perimeter or area.
A decomposable polygon is a polygon that can be divided up into several simpler polygons.
To calculate the perimeter and area of a decomposable figure, use straight lines to divide the initial figure into simpler polygons. Depending on the context, just add up the parts necessary to obtain the answer.
The initial polygon:

can be divided in several ways:
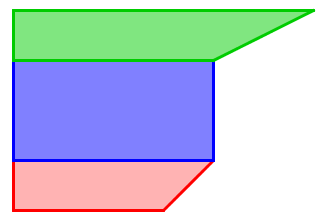
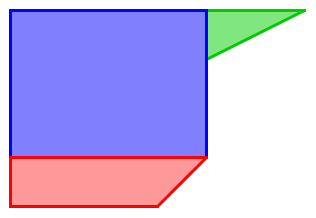
Usually, it is important to consider the given side measurements to properly decompose the initial polygon. As well, it is easier to decompose a polygon using only straight lines, starting from a single vertex in the initial polygon.
For other situations, we use recognizable geometric figures (square, triangle, trapezoid, etc.) that can be subtracted from a larger shape.

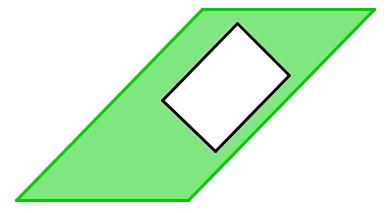
By keeping these two methods in mind, it is easier to calculate the area and perimeter of such figures. Often, recognizing the figures used in each of the drawings makes it easier to determine the missing measurements.