This concept sheet explains the procedures to determine the volume measurement of a substance.
Volume represents the space occupied by the matter contained in an object or a substance. To obtain an adequate reading of the volume, the meniscus must be taken into account. However, depending on the state of the matter, the volume will be measured using different techniques.
In order to take an accurate reading of the volume in a container, some important rules must be observed.
- The container should be placed on a flat surface. It is, therefore, important to place the container on a table rather than holding it in your hands to take the reading.
- The shape of a liquid in a container, the meniscus, must be taken into account.
- Look down to the same level as the meniscus of the liquid inside the container. If your eyes are placed higher or lower than the meniscus, the volume reading will be incorrect.
- If the meniscus is concave, the reading should be taken at the bottom of the meniscus. If the meniscus is convex, the reading should be taken at the top of the meniscus.
- To find the correct volume, identify the scale of the graduated cylinder used. To do this, first count the number of graduations (number of spaces) between two divisions of the graduated cylinder. Then, find the difference in volume between these two divisions. Finally, divide this volume by the number of graduations.
A graduated cylinder of |10 \: \text{mL}| has ten graduations between |8 \: \text{mL}| and |9 \: \text{mL}|.
The difference between the two divisions is |1 \: \text{mL}| |(9 \: \text{mL}-8 \: \text{mL} = 1 \: \text{mL})|.
The scale of this graduated cylinder is therefore |\displaystyle \frac {1 \: \text{mL}}{10 \: \text{graduations}}=0.1 \: \text{mL} |.
- The final step simply consists in reading the volume.
The volume of water in this graduated cylinder is 8.4 mL.
The scale calculation must be carried out each time there is a change of measuring instrument, since the scales are not the same for each instrument. Therefore, a 100 mL graduated cylinder will not have the same scale as the 10 mL graduated cylinder used in the example above.
The volume of regular solids can be determined based on mathematical formulas. The following concept sheet helps to find the appropriate formula according to the shape of the object for which the volume is sought.
Area and Volume of Solids
The graduated cylinder is used to measure the volume of smaller objects.
- Solid to be measured
- Graduated cylinder
- Water
- Lab coat
- Safety glasses
1. Pour water into the graduated cylinder. Record the volume of water.
To ensure that the volume measurement is carried out properly, it is recommended that a volume of water at least equal to the height of the solid to be measured is added so that the solid is completely immersed in the water.
In addition, if a water-soluble solid is measured, it is better to use oil or alcohol.
2. Gently drop the solid into the graduated cylinder.
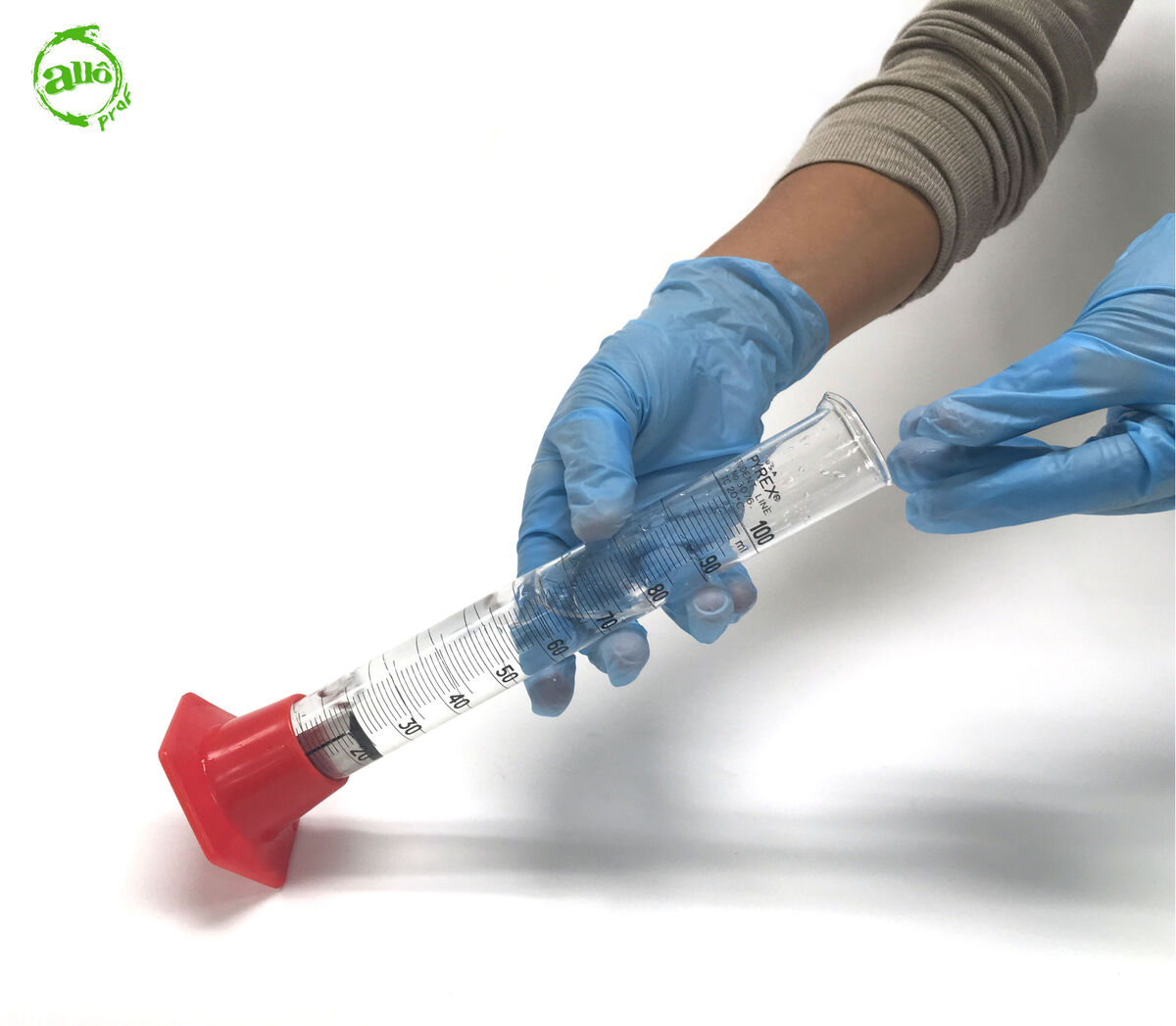
When using a glass graduated cylinder, great care must be taken when placing the solid in the graduated cylinder, as the cylinder may break. It is therefore preferable to slightly tilt the graduated cylinder, place the solid in it, and then gently put the graduated cylinder back to its vertical position.
Alternatively, an object that can absorb the shock, such as a rubber stopper, can be placed at the bottom of the graduated cylinder to prevent the cylinder from breaking.
Care should also be taken to ensure that the solid will not jam in the cylinder opening, thus the opening should be wide enough to accommodate the solid.
3. Record the volume of water with the solid.
4. Calculate the volume of the solid to be measured.
5. Clean and store the equipment.
If possible, it is recommended to repeat the experiment a second time in order to validate the experimental results obtained the first time.
To determine the volume of a solid, the total volume of the solid and water (step 3) must be subtracted from the volume of water initially placed in the graduated cylinder (step 1). This water displacement technique is used to obtain the volume of a solid object.
|{V}_ {{solid}}={V}_ {{water + solid}}-{V}_ {{water}}|
where
|{V}_ {{solid}}| represents the volume of the solid |(\text {mL})|
|{V}_ {{water + solid}}| represents the volume of water and solid |(\text {mL})|
|{V}_ {{water}}| represents the volume of water |(\text {mL})|
It is important to display the experimental results in a table.
Volume of the Solid
| | Volume of the Solid |
| |{V}_ {{water}}| | |\text {mL}| |
| |{V}_ {{water + solid}}| | |\text {mL}| |
| |{V}_ {{solid}}| | |\text {mL}| |
The overflow vessel is used to measure the volume of larger objects, including objects that cannot enter and exit the mouth of a graduated cylinder.
- Solid to be measured
- Overflow vessel
- Water
- Lab coat
- Safety glasses
1. Pour water in the overflow vessel.
When filling the overflow container, water will come out through the spout. It is therefore necessary to make sure that this is done above a sink, or to collect the water to prevent someone from slipping on this water.
2. Place a graduated cylinder under the spout of the overflow vessel.
It is important to ensure that the graduated cylinder is perfectly dry, as the presence of water in the graduated cylinder will impact the measured volume.
3. Gently place the solid in the overflow vessel. At the same time, collect the water flowing through the spout into the graduated cylinder.
It is sometimes difficult to estimate exactly the flow of water that will come out of the spout. It is recommended to proceed smoothly to accurately anticipate the speed with which the water will flow out of the overflow vessel.
It is also advisable to avoid putting fingers in the water, as the water displaced by fingers will be added to the volume of the solid, and will impact the volume measurement.
4. Measure the volume of water in the graduated cylinder.
5. Clean and store the equipment.
If possible, it is recommended to repeat the experiment a second time in order to validate the experimental results obtained the first time.
No further calculations are required as the volume of water collected in the graduated cylinder corresponds to the volume of the solid.
The results must still be displayed in a table. Here is an example:
Volume of the Solid
| | Volume of the solid |
| |{V}_ {{solid}}| | |\text {mL}| |