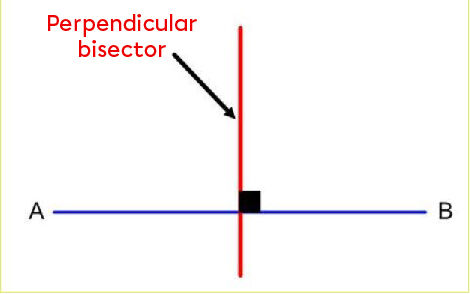
A perpendicular bisector is a line perpendicular to a line segment which passes through the midpoint of the same segment.
There are two ways to draw the perpendicular bisector of a segment:
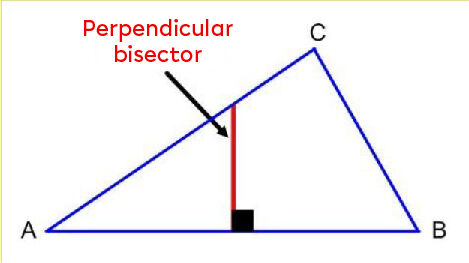
It is possible to draw the perpendicular bisector of a line segment whether this segment is inscribed in a plane figure (on the left) or not (on the right).
To draw the perpendicular bisector of a segment using a compass and ruler, follow these steps:
-
Open the compass so that the opening is larger than one half of the line segment on which the perpendicular bisector will be drawn. Keep the compass open to the same width for all the steps of the construction.
-
Place the needle of the compass on one end of the line segment and draw a circle.
-
Repeat step 2 from the other end of the line segment.
-
Using a ruler, draw a line connecting the two points where the circles intersect. This line is the perpendicular bisector of the line segment.
1. Open the compass so that the opening is larger than one half of the line segment on which the perpendicular bisector will be drawn. Keep the compass open the same width for all the steps of the construction.
2. Place the needle of the compass on one end of the line segment and draw a circle.
3. Repeat step 2 from the other end of the line segment.
4. Using a ruler, draw a line connecting the two points where the circles intersect. This line is the perpendicular bisector of the line segment.
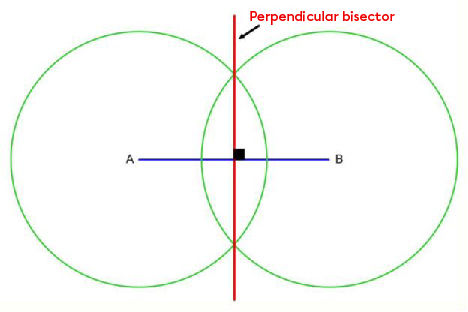
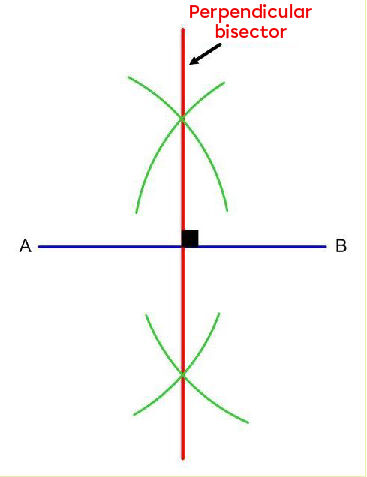
Rather than drawing circles, it’s also possible to simply draw arcs above and below the line segment to construct the perpendicular bisector.
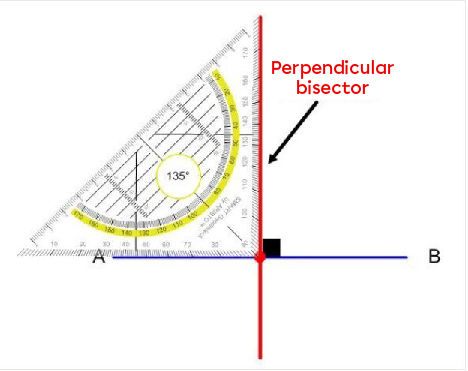
To draw a perpendicular bisector of a segment using a ruler and a set square, follow these steps:
-
Using a ruler, measure the length of the line segment that will be divided into two equal parts.
-
Divide the value of the measurement in half and mark it on the line segment.
-
Align one side forming the set square’s right angle with the line segment to be bisected, and align the other side of the set square with the mark made in step 2.
-
Draw a line perpendicular to the line segment that passes through the point drawn in step 2. This line is the perpendicular bisector of the segment.
1. Using a ruler, measure the length of the line segment that will be divided into two equal parts.
2. Divide the value of the measurement in half and mark it on the line segment.
3. Align one side forming the set square’s right angle with the line segment to be bisected, and align the other side of the set square with the mark made in step 2.
4. Draw a line perpendicular to the line segment that passes through the point drawn in step 2. This line is the perpendicular bisector of the segment.