In certain problems, the main interest is a set of certain numbers.
A set is a group of elements (shapes, numbers, words, etc.) that share a common characteristic.
This concept sheet will exclusively focus on analyzing number sets. Here are some different ways of representing these sets. These are particularly used in representing the solution set to a single-variable inequality.
A set is expressed in tabular form when it is defined by the explicit list of its elements. Thus, all the elements that are part of the set are enumerated between curly brackets.
Consider the set of odd natural numbers between |2| and |10.|
In tabular form, the set is denoted the following way. ||\left\{3,5,7,9\right\}||
Sometimes it is too long or impossible to write all the elements of a set. In these cases, an ellipsis is used.
-
The set of natural numbers greater than or equal to |5.| ||\left\{5,6,7,8,9,10,11,\color{blue}{\ldots}\right\}||
-
The set of negative integers. ||\left\{\color{blue}{\ldots}, \text{-}6,\text{-}5,\text{-}4,\text{-}3,\text{-}2,\text{-}1,0\right\}||
-
The set of multiples of |4| between |3| et |101.| ||\left\{4,8,12,\color{blue}{\ldots},92,96,100\right\}||
Whenever there are units of measure, they can be specified in one of 2 ways.
|\{3\ \text{cm}, 4\ \text{cm}, 5\ \text{cm}, 6\ \text{cm}, 7\ \text{cm}\}|
|\{3,4,5,6,7\}\ \text{cm}|
A set is expressed in interval notation when it is defined by the numbers between which the set is included. These numbers are called the endpoints or bounds of the interval.
An interval is denoted using square brackets |[\ ,\ ].|
-
If the square bracket is turned inwards (closed bracket), the endpoint is included in the interval.
-
If the square bracket faces outwards (open bracket), the endpoint is excluded from the interval.
-
If the set of numbers continues up to positive infinity or negative infinity, the symbols |\infty| or |-\infty| will be used to represent the bound in question. An infinite limit must always have an open square bracket.
This type of representation is limited to real numbers only.
Consider the set of real numbers ranging from |\text{-}1| (included) up to and excluding |24.|
In interval notation, the set is denoted as follows. ||\left[-1,24\right[||
Here, the boundaries of the interval are |-1| and |24.| Note that since |-1| is included in the set, a closed square bracket is used. Since |24| is excluded from the set, an open square bracket is used.
Here are a few examples of sets of real numbers represented as intervals, where one of the limits is infinite.
-
The set of positive real numbers. ||\left[\:0,\infty\:\right[||
-
The set of real numbers less than or equal to |12.| ||\left]\:-\infty,12\:\right]||Since these are numbers less than or equal to |12,| the endpoint |12| is included in the interval.
-
The set of real numbers greater than |3.| ||\left]\:3,\infty\:\right[||Since these are numbers greater than |3,| the endpoint |3| is excluded from the interval.
Whenever there are units of measure, they can be specified in one of 2 ways.
|[20\ \text{kg},25\ \text{kg}[|
|[20,25[\ \text{kg}|
A set is in set builder notation when it is defined by its characteristic properties. The set of numbers is usually indicated between curly brackets, followed by the characteristics of the elements. The vertical line " | " separating the characteristic properties is read "such that".
Consider the set of natural numbers that are multiples of |7.|
In set-builder notation, this set is denoted as follows. ||\left\{x\in\mathbb{N}\mid x \text{ is a multiple of }7\right\}||
Here are some more examples.
-
The set of integers that are greater than |33|.
||\left\{x\in\mathbb{Z}\mid x > 33 \right\}|| -
The set of real numbers between |6| and |42|, inclusive.
||\left\{x\in\mathbb{R}\mid 6 \leq x \leq 42 \right\}|| -
The set of real numbers that are greater than |\text{-}20| and less than or equal to |7|.
||\left\{x\in\mathbb{R}\mid \text{-}20 < x \leq 7 \right\}||
When plotting sets of natural or integer numbers on a number line, the elements are represented by solid points.
-
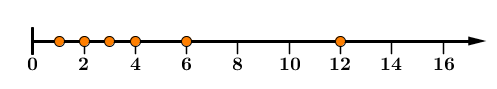
The set of integers less than or equal to |1|.

-
The set of natural divisors of |12|.
When plotting sets of real numbers on the number line, the set is represented by a line bounded by the endpoints of the set.
-
If the limit is included in the interval, it is represented by a solid point.
-
If the limit is excluded from the interval, it is represented by an open point.
The set of real numbers ranging from |\text{-}4|, included, to |2|, excluded.
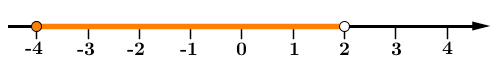
Notice that as the limit |\text{-}4| is included in the set, it is represented by a solid point. Since |2| is excluded from the set, the limit is represented by an open point.
When one of the limits is infinite, it is not plotted on the number line. Simply extend the line as if it continues beyond the number line.
-
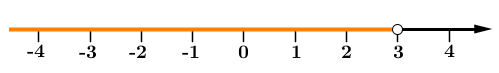
The set of real numbers less than |3.|
-
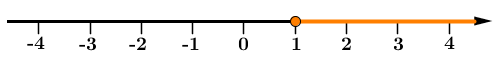
The set of numbers greater than or equal to |1|.