Rounding a number means finding the nearest number that ends in one or more zeros.
We can round to the nearest ten or the nearest hundred.
-
A number rounded to the nearest ten will end with 1 zero.
-
A number rounded to the nearest hundred will end with 2 zeros.
Round the number 236 to the nearest ten and the nearest hundred.
Le nombre 236 est plus proche de 240 que de 230.
Ainsi, lorsque j’arrondis 236 à la dizaine près, j’obtiens 240.
J’arrondis à la centaine près
Je sais que le nombre 236 est situé entre 200 et 300.
Je vérifie si 236 est plus proche de 200 ou de 300 en m'aidant d’une droite numérique.
Le nombre 236 est plus proche de 200 que de 300.
Ainsi, lorsque j’arrondis 236 à la centaine près, j’obtiens 200.
Réponses :
|
Nombre à arrondir |
Nombre arrondi à la dizaine près |
Nombre arrondi à la centaine près |
|---|---|---|
|
236 |
240 |
200 |
Rounding to the Nearest Ten
We know that the number 236 is between 230 and 240.
Using a number line, we can determine if 236 is closer to 230 or 240.
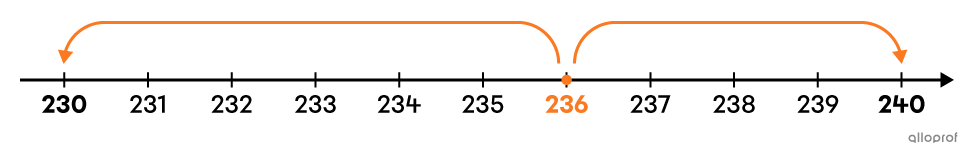
The number 236 is closer to 240 than 230.
So, when we round 236 to the nearest ten, we get 240.
Rounding to the Nearest Hundred
We know that the number 236 is between 200 and 300.
Using a number line, we can determine if 236 is closer to 200 or 300.

The number 236 is closer to 200 than 300.
So, when we round 236 to the nearest hundred, we get 200.
Answers:
|
Number to round |
Number rounded |
Number rounded |
|---|---|---|
|
236 |
240 |
200 |
When a number is exactly halfway between 2 numbers, we always round it up to the higher number.
For example, let’s say we want to round 25 to the nearest ten. Even though 25 is as close to 20 as it is to 30, we have to round it up to 30.
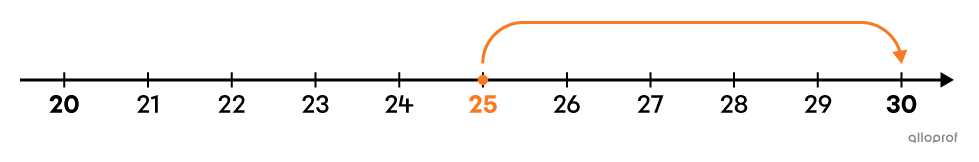
Another example: We want to round 350 to the nearest hundred. Even though 350 is as close to 300 as it is to 400, we have to round it up to 400.
Rounding can be used to approximate the answer to a calculation.
For example, to find the approximate answer to a complex addition problem, we can round the numbers before adding them up.
What is the approximate sum of 57 and 34?
Before adding the numbers, we can round them to the nearest ten.
|
57 is between 50 and 60, but closer to 60, so we know that 57 rounded to the nearest ten is 60. |
|
34 is between 30 and 40, but closer to 30, so we know that 34 rounded to the nearest ten is 30. |
|
57 + 34 |
Answer: The sum of 57 and 34 is approximately 90.
Rounding a number means finding the nearest number that ends in one or more zeros.
A natural number can be rounded up or down to any place value.
For example, we can round to the hundreds place or the tens place. All the digits to the right of the rounded digit will become zeros.
Round the number 236 to the nearest ten and the nearest hundred.
Rounding to the Nearest Ten
We know that the number 236 is between 230 and 240.
Using a number line, we can determine if 236 is closer to 230 or 240.
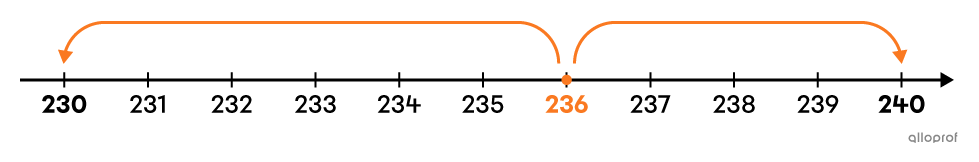
The number 236 is closer to 240 than 230.
So, when we round 236 to the nearest ten, we get 240.
Rounding to the Nearest Hundred
We know that the number 236 is between 200 and 300.
Using a number line, we can determine if 236 is closer to 200 or 300.

The number 236 is closer to 200 than 300.
So, when we round 236 to the nearest hundred, we get 200.
Answers:
|
Number to round |
Number rounded |
Number rounded |
|---|---|---|
|
236 |
240 |
200 |
We can also round a decimal number to any place value. For example, we could round to the ones place or the tenths place. In this case, all the digits to the right of the rounded digit would become zeros.
Round 37.82 to the nearest unit and the nearest tenth.
Rounding to the Nearest Unit
We know that 37.82 is between 37 and 38.
Using a number line, we can determine if 37.82 is closer to 37 or 38.

The number 37.82 is closer to 38 than 37.
So, when we round 37.82 to the nearest unit, we get 38.
Rounding to the Nearest Tenth
We know that 37.82 is between 37.8 and 37.9.
Using a number line, we can determine if 37.82 is closer to 37.8 or 37.9.
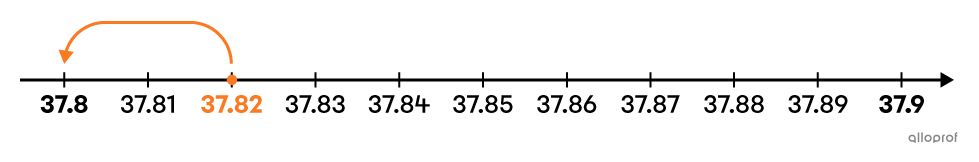
The number 37.82 is closer to 37.8 than 37.9,
So, when we round 37.82 to the nearest tenth, we get 37.8.
Answers:
|
Number to round |
Number rounded |
Number rounded |
|---|---|---|
|
37.82 |
38 |
37.8 |
When a number is exactly halfway between 2 numbers, we always round it up to the higher number.
For example, let’s say we want to round 25 to the nearest ten. Even though 25 is as close to 20 as it is to 30, we have to round it up to 30.

Another example: We want to round 98.50 to the nearest unit. Even though 98.50 is as close to 98 as it is to 99, we have to round it up to 99.
Instead of using a number line to see which number the digit we’re rounding is closer to, we can use the following strategy.
-
Find the digit in the position you’re rounding to.
-
Look at the digit directly to the right.
If it’s 0, 1, 2, 3, or 4, the digit in the position you’re rounding to stays the same.
If it’s a 5, 6, 7, 8, or 9, add 1 to the digit in the position you’re rounding to. -
Replace any digits to the right of the rounded digit with zeros. If any digits to the right of the rounded digit come after a decimal point, you can delete them.
A concert at an outdoor stage attracted a crowd of 91 627 people.
If we round to the nearest ten thousand, approximately how many people attended the concert?
|
The digit in the ten thousands position in 91 627 is 9. |
|
The digit to the right of the 9 in 91 627 is a 1, so we know that the 9 stays the same. |
|
91 627 rounded to the nearest ten thousand is 90 000. |
Answer: Around 90 000 people went to the concert.
Rounding can be used to approximate the answer to a calculation.
For example, to find the approximate answer to a complex addition problem, we can round the numbers before adding them up.
What is the approximate sum of 316 and 588?
Before adding the numbers, we can round them to the nearest hundred.
|
316 is between 300 and 400, but closer to 300, so we know that 316 rounded to the nearest hundred is 300. |
|
588 is between 500 and 600, but closer to 600, so we know that 588 rounded to the nearest hundred is 600. |
|
316 + 588 |
Answer: The sum of 316 and 588 is approximately 900.
Rounding a number means giving a number that is close to the original number based on the desired place value.
Here’s how to round a number.
-
Find the digit in the position you’re rounding to.
-
Look at the digit directly to the right.
If it’s 0, 1, 2, 3, or 4, the digit in the position you’re rounding to stays the same.
If it’s a 5, 6, 7, 8, or 9, add 1 to the digit in the position you’re rounding to. -
Replace the digits to the right of the rounded digit with zeros.
If any digits to the right of the rounded digit come after a decimal point, you can delete them.
A concert at an outdoor stage attracted a crowd of 91 627 people. If we round to the nearest ten thousand, approximately how many people attended the concert?
|
The digit in the ten thousands position in 91 627 is 9. |
|
The digit to the right of the 9 in 91 627 is a 1, so we know that the 9 stays the same. |
|
91 627 rounded to the nearest ten thousand is 90 000. |
Answer: Around 90 000 people went to the concert
Lily spent $24.88 on candy. Approximately how much money is this if we round to the nearest unit?
|
The digit in the ones position in 24.88 is 4. |
|
The digit to the right of the 4 in 24.88 is an 8, so we know that we have to add 1 to the number 4. 4 + 1 = 5 |
|
24.88 rounded to the nearest unit is 25. |
Answer: Lily spent approximately $25 on candy
When rounding up, we need to add a carry digit if the digit in the position we’re rounding to is a 9.
For example, let’s say we want to round 297 to the nearest ten.
|
The digit in the tens position in 297 is 9. |
|
The digit to the right of the 9 in 297 is a 7, so we know that we have to add 1 to the number 9. 9 + 1 = 10 So, we write a 0 in the tens place and add a carry digit of +1 to the hundreds position. +1 |
|
297 rounded to the nearest ten is 300. |
Rounding can be used to approximate the answer to a calculation.
For example, to find the approximate answer to a complex addition problem, we can round the numbers before adding them up.
-
When the result of an operation is exact, we use the symbol “=”, which stands for “equals.”
-
When the result of an operation is exact, we use the symbol “≈”, which stands for “is approximately equal to.”
What is the approximate sum of 120.25 and 45.89?
Before doing the calculation, we need to round both numbers to the nearest whole number.
Rounding 120.25
|
The digit in the ones position 120.25 is 0. |
|
The digit to the right of the 0 in 120.25 is a 2, so we know that the 0 stays the same. |
|
120.25 rounded to the nearest unit is 120.00 or simply 120. |
Rounding 45.89
|
The digit in the ones position in 45.89 is 5. |
|
The digit to the right of the 5 in 45.89 is an 8, so we know that we have to add 1 to the number 5. 5 + 1 = 6 |
|
45.89 rounded to the nearest unit is 46.00 or simply 46. |
Add the rounded numbers.
120.25 + 45.89 = ?
↓ ↓
120 + 46 ≈ 166
Answer: The sum of 120.25 and 45.89 is approximately 166.