Comparing integers makes it possible to sort them in relation to one another. They can then be placed in ascending or descending order.
Sorting numbers in ascending order means sorting them according to their value from smallest to largest, as follows.
||...\ <\ -4\ <\ -3\ <\ -2\ <\ -1\ <\ 0\ <\ 1\ <\ 2\ <\ 3\ <\ 4\ <\ ...||
Sorting numbers in descending order means sorting them according to their value from largest to smallest, as follows.
||...\ >\ 4\ >\ 3\ >\ 2\ >\ 1\ >\ 0\ >\ -1\ >\ -2\ >\ -3\ >\ -4\ >\ ...||
The order of integers is similar to the one for natural numbers. The main difference is that integers contain negative whole numbers.
The order of integers can be represented in several ways. The following are two methods.
Assets and debts are often used to represent the order of integers. The term “debts” refers to negative numbers and the term “assets” refers to positive numbers.
Jeremy has |$2.| His friend, Gregory, owes |$4.|
Jeremy has money. His assets are represented by a positive number. In other words, Jeremy has |+$2.|
Gregory's debt is represented by a negative number because he owes money. Gregory has |-$4.|
We can determine Jeremy has more money than Gregory, i.e., |-4\ <\ 2.|

Generally, a positive number is always greater than a negative number.
The following is another example of how to order integers.
Let's go back to our friend, Gregory, who is in debt |$4.|
He is richer than Sophie, who is in debt |$10.|
Mathematically, it is written |-10<-4.|
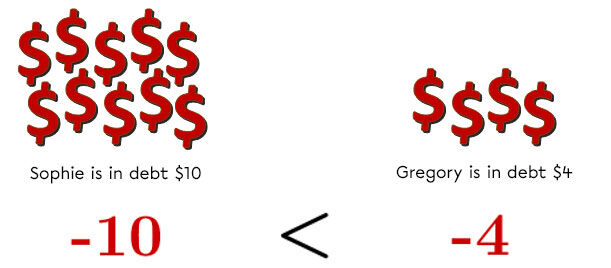
Generally, the further a negative number is from |0,| the smaller it is.
As with natural numbers, it is possible to represent the order of integers using a number line.
Once placed on a number line, the farther a number is placed to the right, the larger it will be.
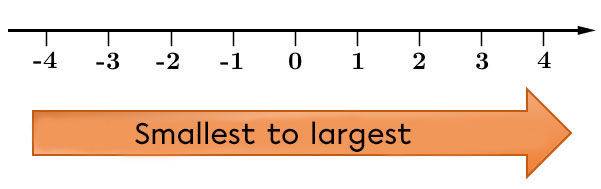
Conversely, the farther a number is positioned to the left, the smaller it will be.
The following numbers are represented on the number line.
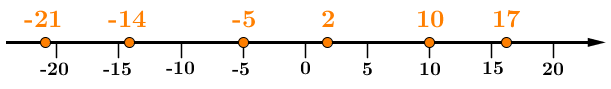
On the number line, |-21| has the smallest value, because it is positioned farthest to the left on the number line. Number |17| has the greatest value, because it is positioned farthest to the right.
The ascending order of the numbers is the result of entering them from left to right. ||-21\ <\ -14\ <\ -5\ <\ 2\ <\ 10\ <\ 17||
We obtain the decreasing order by writing the numbers from right to left. ||17\ >\ 10\ >\ 2\ >\ -5\ >\ -14\ >\ -21||
There are several ways to order integers. Here are two of them.
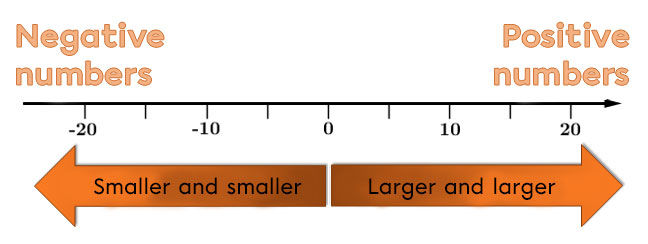
There are three rules for describing the order of integers.
-
The farther a negative number is from |0,| the smaller it is.
-
The farther a positive number is from |0,| the bigger it is.
-
A positive number is always greater than a negative number.
Using the three rules enables integers to be placed in order. This method works as follows.
-
Determine whether to place the numbers in ascending or descending order.
-
Separate the numbers into two groups: negative numbers and positive numbers.
-
Use rules 1 and 2 to sort the negative and positive numbers in the desired order.
-
Use rule 3 to sort the two groups of numbers.
Place the following numbers in ascending order. ||42\qquad 59\qquad\ \text{-}32\qquad \text{-105}\qquad 56\qquad\text{-38}||
-
Determine whether to place the numbers in ascending or descending order
As mentioned in the question, the numbers should be placed in ascending order, i.e., from smallest to largest. -
Sort the numbers into two groups: negative numbers and positive numbers
||\underbrace{\color{blue}{-32},\color{blue}{-105},\color{blue}{-38}}_{\text{Negative numbers}}\qquad \qquad \qquad \underbrace{\color{green}{42},\color{green}{56},\color{green}{59}}_{\text{Positive numbers}}|| -
Use rules 1 and 2 to sort the negative and positive numbers in the desired order
According to rule 1, number |\color{blue}{-105}| is the smallest, followed by number |\color{blue}{-38},| then |\color{blue}{-32}.| ||\color{blue}{-105}\ <\ \color{blue}{-38}\ <\ \color{blue}{-32}||
According to rule 2, number |\color{green}{59}| is the largest, preceded by |\color{green}{56},| then |\color{green}{42}.| ||\color{green}{42}\ <\ \color{green}{56}\ <\ \color{green}{59}|| -
Use rule 3 to combine the two groups of numbers
Rule 3 states that a positive number is always greater than a negative number. This results in the following ascending order. ||\color{blue}{-105}\ <\ \color{blue}{-38}\ <\ \color{blue}{-32}\ <\ \color{green}{42}\ <\ \color{green}{56}\ <\ \color{green}{59}||
Integers can be placed in order using a number line. The steps for this method are as follows.
-
Determine whether to place the numbers in ascending or descending order.
-
If it is not already provided, draw a number line.
-
Accounting for the graduation mark, sort the numbers to be ordered on the number line.
-
Sort the numbers in the desired order. The farther a number is positioned to the right, the larger it is.
Sort the following numbers in descending order. ||-250\qquad 990\qquad -550\qquad -890\qquad 555||
-
Determine whether to place the numbers in ascending or descending order
As stated in the question, the numbers should be placed in descending order, i.e., from largest to smallest. -
If it is not already provided, draw a number line
We decide to draw a number line with a graduation mark of |250.|
-
Accounting for the graduation mark, sort the numbers to be put in order on the number line.
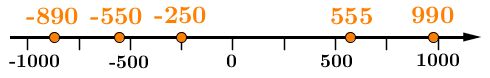
-
Place the numbers in the desired order.
The largest numbers furthest to the right, resulting in the following descending order. ||990\ >\ 555\ >\ -250\ >\ -550\ >\ -890||