When a sour substance is put into aqueous solution, the equilibrium constant takes on a particular form. Scientists have therefore developed a variant of the equilibrium constant to be applied in this situation: the acidity constant. However, an equilibrium reaction can only occur when a weak acid dissociates. This is because strong acids dissociate completely in solution, which is a complete and therefore irreversible reaction.
The strength of an electrolyte, whether acidic or alkaline, corresponds to the percentage of dissociation of the substance into ions when put into solution.
A strong electrolyte is a solute that dissociates completely in water.
A weak electrolyte is a solute that does not dissociate completely in water.
As stated in the Arrhenius and BrØnsted-Lowry theories, acids and bases are substances that release ions when in aqueous solution. They are therefore electrolytes. However, they do not all undergo electrolytic dissociation to the same degree. This explains the presence of strong and weak electrolytes. Before studying the acidity constant, it is important to distinguish between these substances according to their strength, since only weak electrolytes will be in a state of chemical equilibrium.
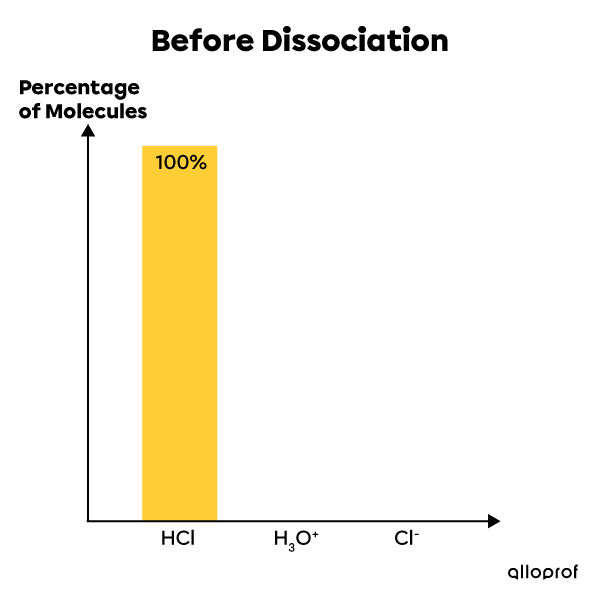
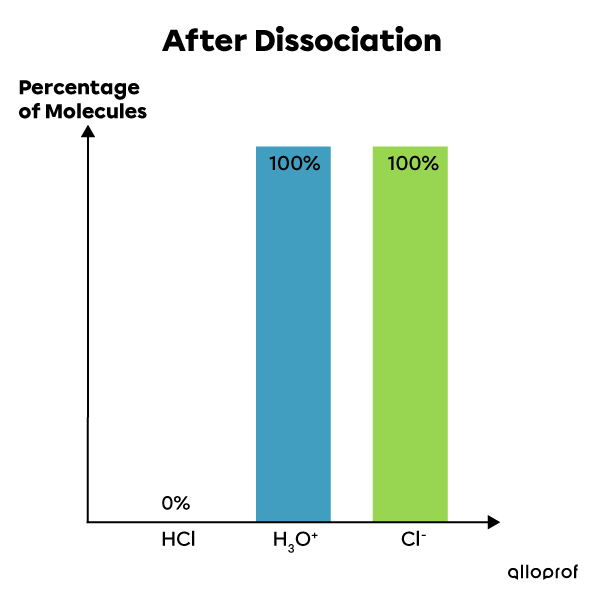
Hydrochloric acid is a strong electrolyte. When dissolved in water, it dissociates completely into ions. 100% of its molecules dissociate to form |H^{+}| and |Cl^{-}| ions. The solution then contains the same percentage of ions after dissociation as there were molecules of acid before dissociation. It is therefore a complete reaction that cannot be at equilibrium. This chemical reaction is written as follows:|HCl_{(aq)} + H_{2}O_{(l)} \rightarrow H_{3}O^{+}_{(aq)} + Cl^{-}_{(aq)}|
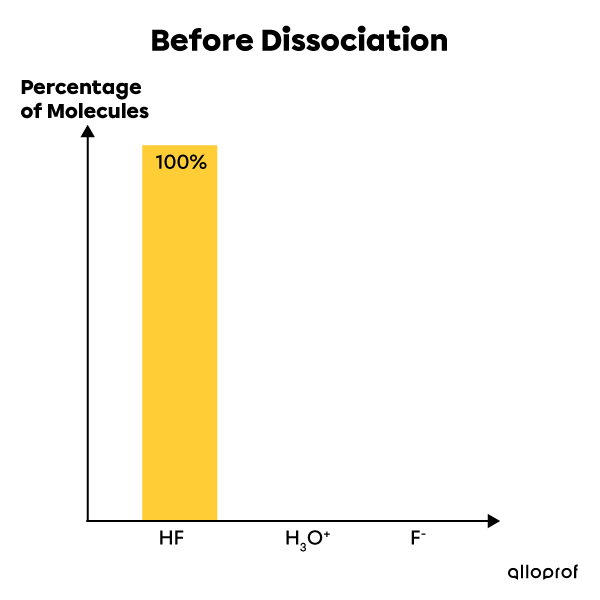
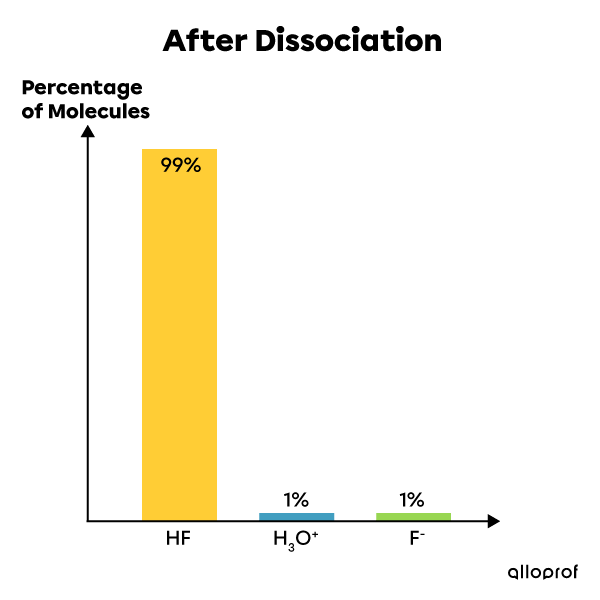
Hydrofluoric acid is a weak electrolyte. When dissolved in water, only a component of its molecules dissociates into ions. Most of its molecules remain whole after dissolution, while a very small percentage dissociate to form |H^{+}| and |F^{-}| ions. The solution therefore contains both reactants (the whole molecule) and products (the ions in solution). It is therefore an incomplete and reversible reaction at equilibrium. This chemical reaction can be written as follows:|HF_{(aq)} + H_{2}O_{(l)} \rightleftharpoons H_{3}O^{+}_{(aq)} + F^{-}_{(aq)}|
Only weak electrolytes can be in a state of equilibrium.
The percentage of molecules that dissociate in a solvent can be calculated using the formula for percentage ionisation:
|\text {Percentage of ionisation} = \displaystyle \frac{[H_{3}O^{+}]}{[HA]}\times 100|
where
|[H_{3}O^{+}]|represents the concentration of ions
|[H_{3}O^{+}]| in equilibrium |\small (\text {mol/L})|
|[HA]| represents the concentration of the acid before dissolution |\small (\text {mol/L})|
As well as giving us the percentage ionisation of the electrolyte, this formula allows us to determine the concentration of ions in an equilibrium situation. We can then calculate the acidity constant.
Most acids are weak acids that can reach equilibrium in aqueous solution. At equilibrium, the molecules of the acid dissociate at the same velocity as the ions formed recombine to reform the molecules of the acid. This reaction can be generalised as follows:
|HA_{(aq)} + H_{2}O_{(l)} \rightleftharpoons H_{3}O^{+}_{(aq)} + A^{-}_{(aq)}|
or even:
|HA_{(aq)} \rightleftharpoons H^{+}_{(aq)} + A^{-}_{(aq)}|
The equilibrium constant associated with the ionisation of an acid, called the ‘acidity constant’, is then expressed as follows:
|K_{a}=\displaystyle \frac{[H^{+}][A^{-}]}{[HA]}|
where |K_{a}| represents the acidity constant
|[H^{+}]| represents the concentration of hydronium ions in water |\small (\text {mol/L})|
|[A^{-}]|represents the concentration of the basic or alkaline conjugate |\small (\text {mol/L})|
|[HA]| represents the concentration of the undissociated acid |\small (\text {mol/L})|
The acidity constant |K_{a}| is a variant of the equilibrium constant . It does not include the concentration of water since water is in the liquid phase. The acidity constant is used to classify acids according to their strength. The smaller the constant, the weaker the acid.
The pH of a solution of barbituric acid |(C_{4}H_{4}N_{2}O_{3})| à |\small \text {0.10 mol/L}| is 2.5. What is its acidity constant? What is its ionisation percentage?
1. Expression of the acidity constant
|[C_{4}H_{4}N_{2}O_{3}] \rightleftharpoons [H^{+}] + [C_{4}H_{3}N_{2}O_{3}^{-}]|
|K_{a} = \displaystyle \frac{[H^{+}][C_{4}H_{3}N_{2}O_{3}^{-}]}{[C_{4}H_{4}N_{2}O_{3}]}|
2. Transformation of pH into molar concentration
|[H^{+}] = 10^{-pH}|
|[H^{+}] = 10^{-2.5}|
|[H^{+}] = 3.16\times 10^{-3} \text { mol/L}|
3. Table of equilibrium concentrations
| | |C_4H_4N_2O_3| | |\rightarrow| | |H^+| | |+| | |C_4H_3N_2O_3^-| |
| [Initial] | |\color{red}{0,10}| | |\huge\Rightarrow| | |\color{red}{0}| | |\huge +| | |\color{red}{0}| |
| [Variation] | |(-3.16\times10^{-3})| | |(+3.16\times10^{-3})| | |(+3.16\times10^{-3})| | ||
| [Equilibrium] | |9.68\times10^{-2}| | |\color{red}{3.16\times10^{-3}}| | |3.16\times10^{-3}| |
4. Calculation of the acidity constant
|K_{a}=\displaystyle \frac{[3,16\times 10^{-3}][3.16\times 10^{-3}]}{[9.68\times 10^{-2}]}|
|K_{a}=1,03\times 10^{-4}|
5. Calculation of the ionisation percentage
|\text {Percentage of ionisation} = \displaystyle \frac{[3.16\times 10^{-3}]}{[0.10]}\times 100|
|\text {Percentage of ionisation} = 3.16| %
What will be the pH of a solution of formic acid |HCOOH| with a concentration of |\small \text {0.10 mol/L}|, if the acidity constant of this acid is |1.77\times 10^{-4}|?
1. Expression of the acidity constant
|[HCOOH] \rightleftharpoons [H^{+}] + [HCOO^{-}]|
|K_{a} = \displaystyle \frac{[H^{+}][HCOO^{-}]}{[HCOOH]}|
2. Table of equilibrium concentrations
| | |HCOOH| | |\rightarrow| | |H^+| | |+| | |HCOO^-| |
| [Initial] | |\color{red}{0,10}| | |\huge\Rightarrow| | |\color{red}{0}| | |\huge +| | |\color{red}{0}| |
| [Variation] | |(-x)| | |(+x)| | |(+x)| | ||
| [Equilibrium] | |0.10 - x| | |x| | |x| |
3. Calculation of the concentration of |H^{+}| ions
|1.77\times 10^{-4} = \displaystyle \frac{[x][x]}{[0.10 - x]}|
This is a second-degree equation in which the x must be insulated. The only possible answer is:
|[H^{+}]| = |4.1\times 10^{-3}\text { mol/L}|
4. Calculating pH
|pH = -\log[H^{+}]|
|pH = -\log[4.1\times 10^{-3}]|
|pH = 2.39|
The following tools can be useful in calculating |K_{a}|: