This Crash Course is a review of the laws of logarithms covered in Secondary 5. The laws of exponents are also used in this video. You can watch the Crash Course on the laws of exponents if you need a review.
To understand this video, you must be able to apply the laws of exponents. You should also be familiar with the following vocabulary words: logarithm, base and argument.
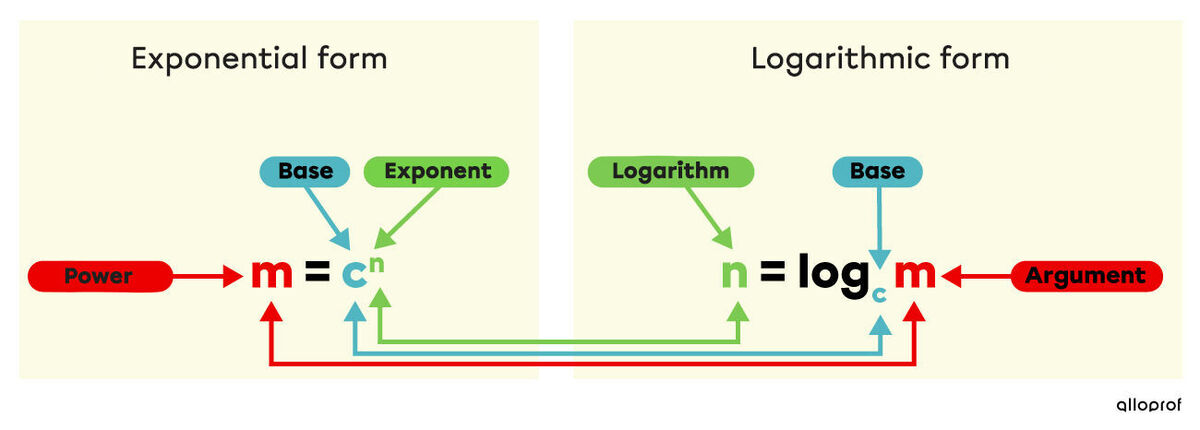
When carrying out exercises on the laws of logarithms and exponents, you need to be able to switch from one form to the other.
A logarithm with the base |e| is called a natural logarithm and is denoted |\ln| rather than |\log_e.|
When the base of a logarithm is |10|, it is simply denoted |\log| rather than |\log_10.|
The change of base is a property that enables us to calculate the value of a logarithm whose base is neither |e| nor |10.|
Example: |\log_{9} 27 = \dfrac{\log_3 27}{\log_3 9} = \dfrac{3}{2}|
Here is a summary of the laws of logarithms and an example of each property:
|
Property |
Rule |
Example |
|---|---|---|
|
Logarithm of 1 |
|\log_c (1) = 0| |
|\log_{9} (1) = 0| |
|
Logarithm where the argument is the same as the base |
|\log_c (c) = 1| |
|\log_7 (7)= 1| |
|
Power law for logarithms |
|\log_c \left(M^n\right) = n\log_c (M)| |
|\log_2 \left(9^5\right) = 5 \log_2 (9)| |
|
Product law for logarithms |
|\log_c \left( M \times N \right) = \log_c (M) + \log_c (N)| |
|\begin{align} \log_3(48) &= \log_3(6\times 8) \\&= \log_3(6)+\log_3(8) \end{align}| |
|
Quotient law for logarithms |
|\log_c \left(\dfrac{M}{N}\right) = \log_c (M) - \log_c (N)| |
|\begin{align} \log_{13}(45)-\log_{13}(5) &= \log_{13} \left(\dfrac{45}{5}\right) \\ &= \log_{13}(9) \end{align}| |
|
Fractional base law for logarithms |
|\log_\frac{1}{c}(M) = -\log_c (M)| |
|\log_\frac{1}{4} (91) = -\log_4 (91)| |
|
Special case |
|\log_{c}\left(c^t\right) = t| |
|\log_{3} (81) = \log_{3} \left(3^4\right) = 4| |