Adding Fractions

1. We look for a common denominator.

2. For each fraction, find an equivalent fraction.

3. We only add the numerators.

||\dfrac{2}{3}+\dfrac{1}{6}||

1. We look for a common denominator.
   
   Here, the common multiple for both |3| and |6| is |6.| Thus, the common denominator is |6.| ||\dfrac{?}{6}+\dfrac{?}{6}||

2. For each fraction, find an equivalent fraction.
   
   To convert the fractions into equivalent fractions, multiply the numerator and the denominator by the same factor. ||\begin{align}\dfrac{2}{3}=\dfrac{2\times{\color{red}2}}{3\times{\color{red}2}}=\dfrac{4}{6}\\\\\dfrac{1}{6}=\dfrac{1\times{\color{red}1}}{6\times{\color{red}1}}=\dfrac{1}{6}\end{align}||

3. We only add the numerators. ||\dfrac{4}{6}+\dfrac{1}{6}=\dfrac{4+1}{6}=\dfrac{5}{6}||

||\dfrac{7}{8}+\dfrac{2}{3}||

1. We look for a common denominator.

   Here, the common multiple for both |8| and |3| is |24.| Thus, the common denominator is |24.| ||\dfrac{?}{24}+\dfrac{?}{24}||

2. For each fraction, find an equivalent fraction.

   To convert the fractions into equivalent fractions, multiply the numerator and the denominator by the same factor.

   ||\dfrac{7}{8}=\dfrac{7\times{\color{red}3}}{8\times{\color{red}3}}=\dfrac{21}{24}||
   
   ||\dfrac{2}{3}=\dfrac{2\times{\color{red}8}}{3\times{\color{red}8}}=\dfrac{16}{24}||

3. We only add the numerators.
   
   ||\dfrac{21}{24}+\dfrac{16}{24}=\dfrac{21+16}{24}=\dfrac{37}{24}||

||2\frac{1}{3}+3\frac{1}{3}||

First, focus on the integers: |2 + 3 = 5.| 

Then the fractions, thus |\dfrac{1}{3}+\dfrac{1}{3}=\dfrac{2}{3}.| 

The result is |2\dfrac{1}{3}+3\dfrac{1}{3} = 5\dfrac{2}{3}.| 
 

||\begin{align} 5\frac{1}{3}+2\frac{2}{5} &= \frac{16}{3}+\frac{12}{5} \\ &= \frac{80}{15} + \frac{36}{15} \\ &= \frac{80+36}{15} \\ &=\frac{116}{15} \\ &=7\frac{11}{15} \end{align}||

For example, using the fraction |\dfrac{3}{4},| the 4^th line marks the whole, or the fraction |\dfrac{4}{4}.|

1. We look for the common denominator of the fractions.

2. For each fraction, we create an equivalent fraction.

3. We segment each whole on the number line into as many parts as the value of the common denominator.

4. We place the 1^st fraction on the number line according to its numerator.

5. We add the 2^nd fraction to the 1^st.

We want to add |\dfrac{3}{8}+\dfrac{1}{4}.|

1. We look for the common denominator of the fractions.
   
   Here, the common denominator of |4| and |8| is |8.|

2. For each fraction, we create an equivalent fraction.
   
   To convert the fractions into equivalent fractions, we multiply the numerator and the denominator by the same factor. ||\begin{align}\dfrac{3}{8}=\dfrac{3\times{\color{red}1}}{8\times{\color{red}1}}=\dfrac{3}{8}\\\\ \dfrac{1}{4}=\dfrac{1\times{\color{red}2}}{4\times{\color{red}2}}=\dfrac{2}{8}\end{align}||

3. We segment each whole on the number line in as many parts as the value of the common denominator.

4. We place the 1^st fraction on the number line according to its numerator.

5. We add the 2^nd fraction to the 1^st.

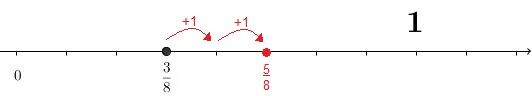
Therefore, |\dfrac{3}{8}+\dfrac{1}{4}=\dfrac{3}{8}+\dfrac{2}{8}=\dfrac{5}{8}.|