To convert a decimal into a fraction, it is important to understand place value and the parts that make up decimals and fractions.
Read the concept sheets Place value, Decimal numbers, and Fractions to learn more.
A decimal number can be converted into a fraction out of ten |\left(\dfrac{?}{10}\right),| out of a hundred |\left(\dfrac{?}{100}\right),| or out of a thousand |\left(\dfrac{?}{1\ 000}\right).|
When the decimal part contains only one digit in the tenths position, it can be converted into a fraction out of ten |\left(\dfrac{?}{10}\right).|
The number |0.8| contains a single digit in the decimal part. |8| occupies the tenths position. So, I can convert |0.8| into a fraction out of ten.
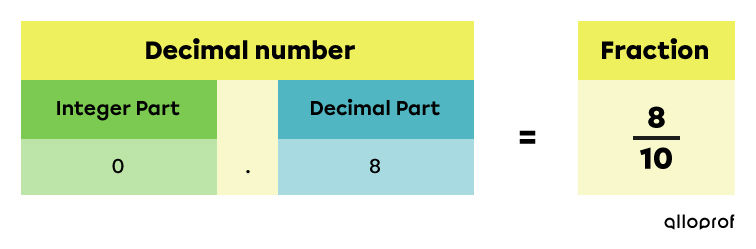
When the decimal part contains a digit in the tenths position and a digit in the hundredths position, it can be converted into a fraction out of a hundred |\left(\dfrac{?}{100}\right).|
The number |0.72| contains two digits in the decimal part. |7| occupies the tenths position and |2| occupies the hundredths position. So, I can convert |0.72| into a fraction out of a hundred.
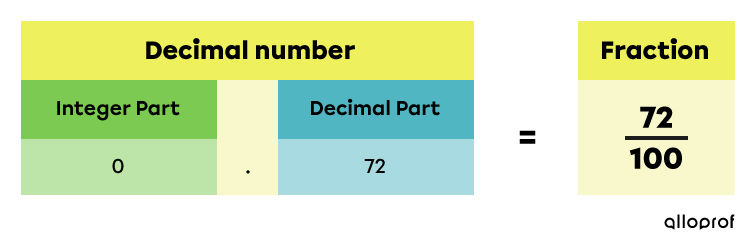
The number |0.04| contains two digits in the decimal part. |0| occupies the tenths position and |4,| the hundredths position. So, I can convert |0.04| into a fraction out of a hundred.
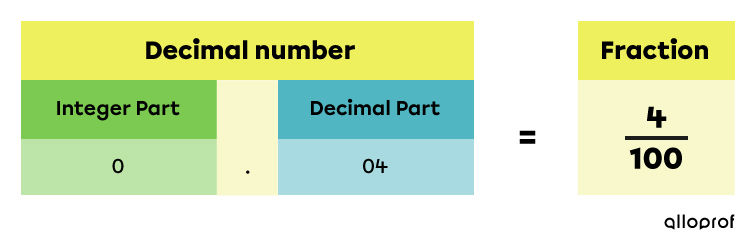
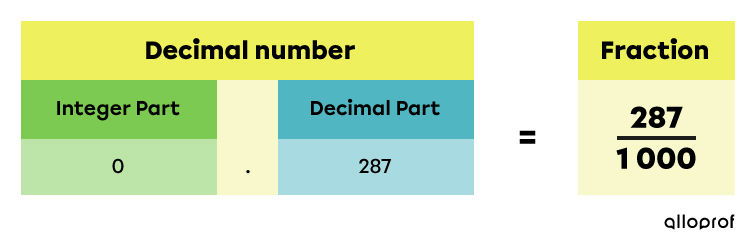
When the decimal part contains a digit in the tenths position, a digit in the hundredths position, and a digit in the thousandths position, it can be converted into a fraction out of a thousand |\left(\dfrac{?}{1\ 000}\right).|
The number |0.287| contains three digits in the decimal part. |2| occupies the tenths position, |8,| the hundredths position, and |7,| the thousandths position. So, I can convert |0.287| into a fraction out of a thousand.
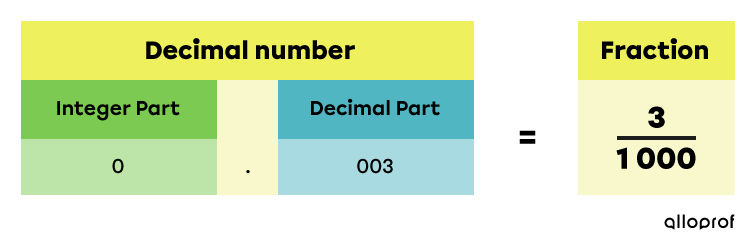
The number |0.003| contains three digits in the decimal part. |0| occupies the tenths and hundredths positions. |3| occupies the thousandths position. Therefore, I can convert |0.003| into a fraction out of a thousand.
Make sure to follow the instructions. Sometimes the answers may have to be expressed in simplest form.
Read the Irreducible fractions concept sheet to learn how.
To convert a decimal number into a mixed number, it is important to understand place value and the parts that make up decimals and mixed numbers.
Read the concept sheets on place value, decimal numbers, and mixed numbers to learn more.
When the decimal part contains only one digit in the tenths position, you can convert it into a mixed number where the fraction is over ten |\left(\dfrac{?}{10}\right).|
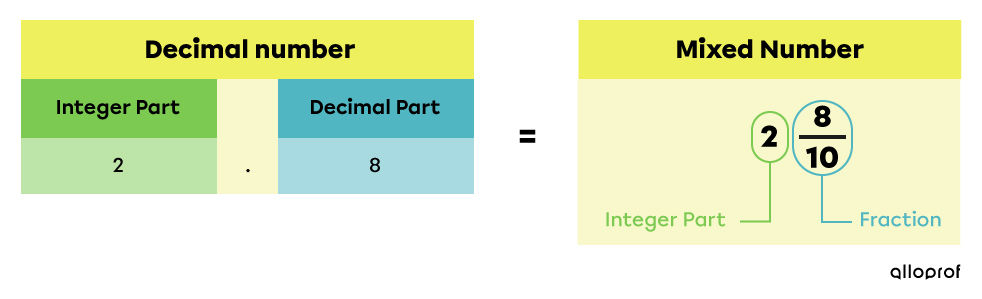
The number |2.8| contains a single digit in the decimal part. |8| occupies the tenths position. So, I can convert |2.8| into a mixed number whose fraction is out of ten.
When the decimal part contains a digit in the tenths position and a digit in the hundredths position, you can convert it into a mixed number whose fraction is over hundred |\left(\dfrac{?}{100}\right).|
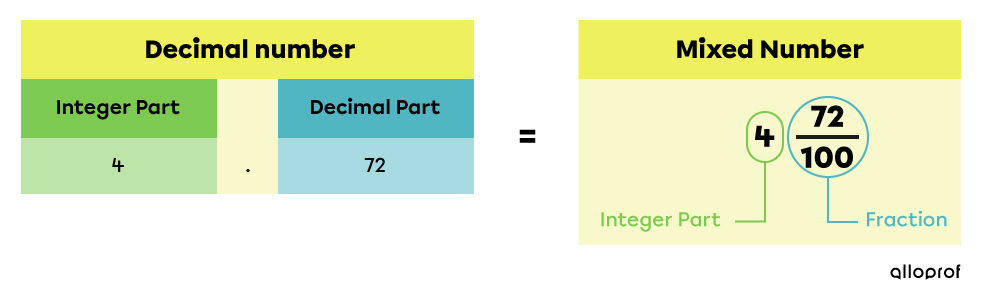
The number |4.72| contains two digits in the decimal part. |7| occupies the tenths position and |2| occupies the hundredths position. So, I can convert |4.72| into a mixed number whose fraction is over a hundred.
When the decimal part contains a digit in the tenths position, a digit in the hundredths position, and a digit in the thousandths position, you can convert it into a mixed number whose fraction is over a thousand |\left(\dfrac{?}{1\ 000}\right).|
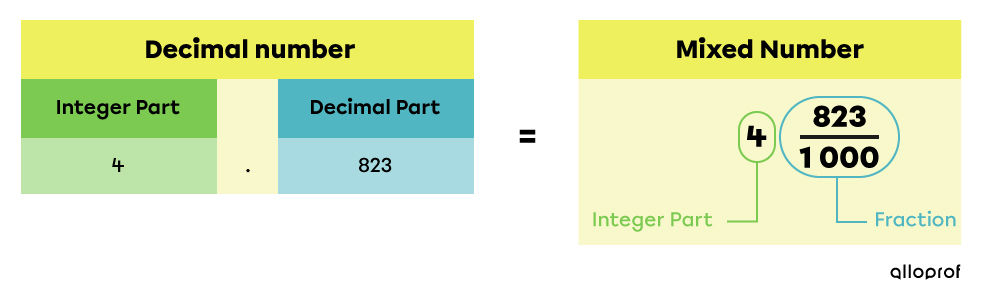
The number |4.823| contains three digits in the decimal part. |8| occupies the tenths position, |2,| the hundredths position, and |3,| the thousandths position. Therefore, I can convert |4.823| into a mixed number whose fraction is out of a thousand.
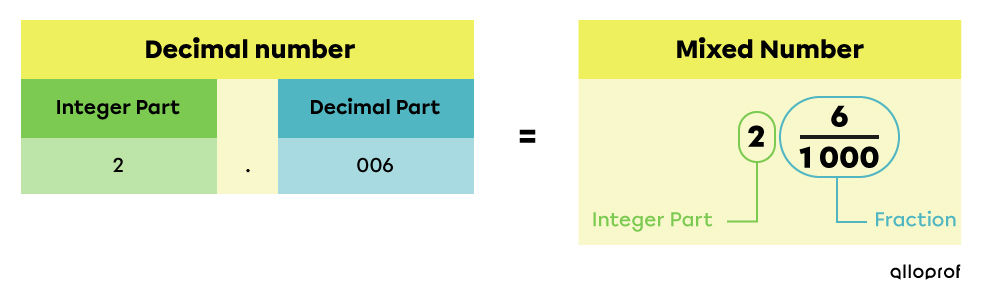
The number |2.006| contains three digits in the decimal part. |0| occupies the tenths and hundredths positions, and |6,| the thousandths position. So, I can convert |0.006| into a mixed number whose fraction is out of a thousand.
The answer can be written as a mixed number or a fraction.

Make sure to follow the instructions. Sometimes the answer will have to be expressed in simplest form.
Read the Irreducible fractions concept sheet to learn how to find an irreducible fraction.
I have to follow these steps to convert a decimal number into a percentage.
-
I multiply by |100.|
-
I write the percent symbol (%).
Convert the decimal number |0.5| into a percentage.
|
0.5 × 100 = 50 |
|
50 % |
|0.5| becomes 50 %.
Convert the decimal number |1.8| into a percentage.
|
1.8 × 100 = 180 |
|
180 % |
|1.8| becomes 180 %.
Convert the decimal number |0.72| into a percentage.
|
0.72 × 100 = 72 |
|
72 % |
|0.72| becomes 72 %.