Translations are used to slide geometric shapes on a plane.
A translation is a geometric transformation that produces an image figure from an initial figure by "sliding" it.
In addition, a translation can be defined by a translation arrow t that indicates:
-
the direction of the displacement, by its slope;
-
the orientation of the displacement, by its point;
-
the distance between the corresponding points of the initial shape and of the image shape, by its length
A translation is a geometric transformation that generates isometric shapes. The initial figure and the image figure are the same shape and have the same dimensions. A translation is an isometry (as are a rotation and a reflection).
All the points of the translated image can be connected to their corresponding points on the initial shape using a single arrow, called the translation arrow.
In other words, points that have the same position in the initial and image figures of a translation are called corresponding points.
To distinguish between the corresponding points in the initial figure and the image figure, we use the ‘’ ' ‘’ symbol (called prime). Vertex A in the initial figure becomes vertex A' in the image figure.
The properties of translations can be used to verify if an image has been generated from a translation, or to demonstrate the construction of an image by translation.
| Properties of a Translation | Example |
|---|---|
|
The corresponding sides of an initial shape and its image are parallel. |
|\overline{AB} // \overline{A'B'},| |\overline{AD} // \overline{A'D'},| |\overline{BC} // \overline{B'C'}| and |\overline{CD} // \overline{C'D'}| |
|
The order and orientation of the corresponding vertices are preserved. |
Vertices |A,| |B,| |C| and |D| are placed in the same order as |A',| |B',| |C'| and |D'.| In this case, they are counterclockwise. |
|
The line segments drawn to connect the corresponding vertices are parallel and isometric. |
|\overline{AA'} // \overline{BB'} // \overline{CC'} // \overline{DD'}| |\overline{AA'} \cong \overline{BB'} \cong \overline{CC'} \cong \overline{DD'}| |
These properties allow us to verify if the translation was carried out correctly and to recognize it amongst the other geometric transformations.
The image figure generated by a translation can be drawn using the following steps:
-
Using a ruler and set square, draw lines that are parallel to translation arrow t through each of the vertices of the figure.
-
Use a ruler or open a compass to the length of the translation arrow and lock it for use in later steps.
-
Position the needle point of the compass on one vertex of the initial shape and mark the compass measurement on the straight line parallel to the translation arrow by drawing a small arc on it. You can also use a ruler.
-
Name the resulting image vertices using the prime symbol (') and then connect them in the correct order to create the image figure.
To construct the image of the polygon below using a translation, follow these steps:
|
1. Using a ruler and set square, draw lines that are parallel to translation arrow t through each of the vertices of the figure. |
2. Use a ruler or open a compass to the length of the translation arrow and lock it for use in later steps. |
|
3. Position the needle point of the compass on one vertex of the initial figure and mark the compass measurement on the straight lines parallel to the translation arrow by drawing small arcs on them. You can also use a ruler. |
4. Label the resulting image vertices using the prime symbol (') and then connect them in the correct order to create the image figure. |
If a diagram shows the initial figure and the image figure generated from a translation, it is possible to find the translation arrow that was used during its construction.
-
Identify the initial figure and the image figure.
The image figure is the figure whose vertices are identified by the " ' " symbol. Here, the image figure is the green triangle, and the blue triangle is the initial figure.
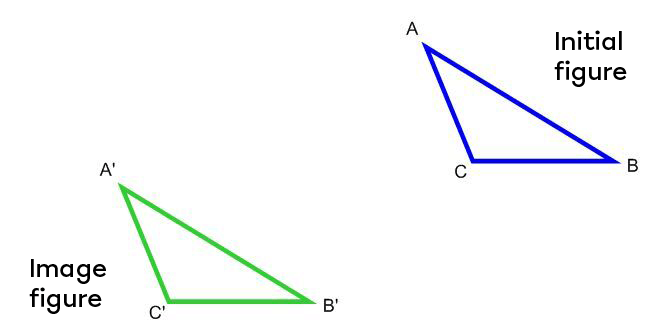
-
Sketch translation arrow t.
To do this, simply connect the corresponding vertices of the two figures with a straight line and indicate the direction of the translation by an arrow.