Pressure is a force applied to a surface.
To determine the pressure exerted on an object or a fluid, use the following formula:
|P= \dfrac{F}{A}|
where
|P:| pressure in Pascals |(\text{Pa})|
|F:| force in Newtons |(\text{N})|
|A:| area to which the force is applied |(\text{m}^2)|
What pressure is exerted by a book that applies a force of |20\ \text{N}| on a |10\ \text{cm}| by |30\ \text{cm}| table?
First, determine the surface area of the book in |\text{m}^2.|
|\begin{align}10\ \text{cm} &= 0.1\ \text{m}\\30\ \text{cm} &= 0.3\ \text{m}\\A_{rectangle} &= b \times h\\A_{rectangle} &= 0.1\ \text{m} \times 0.3\ \text{m}\\A_{rectangle} &= 0.03\ \text{m}^2\end{align}|
Then determine the pressure using the formula in the box above.
|\begin{align} P&=\dfrac{F}{A}\\P&=\dfrac{20\ \text{N}}{0.03\ \text{m}^2}\\P&= 666.67\ \text{Pa} \end{align}|
On Earth, because of gravity, the air around us exerts a pressure called atmospheric pressure.
Two factors influence the pressure exerted by an object.
The greater the mass of an object, the greater the gravitational force it exerts. The greater the force it exerts, the greater the pressure it exerts as well.
When two people are walking on the snow, the person with the larger mass sinks deeper into the snow than the person with the smaller mass.

When the force is distributed over a larger area, the pressure is lower.
If a person wears snowshoes while walking on the snow, they sink less, because their mass is spread over a larger area than just the surface of their boot soles.
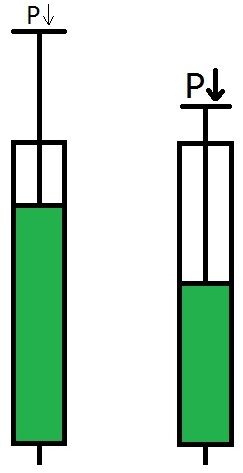
In a compressible fluid, pressure is inversely proportional to volume.
|P \alpha \dfrac{1}{V}|
When the pressure increases, the volume decreases.
When the pressure decreases, the volume increases.
In a bicycle pump, when a small amount of pressure is applied to the piston, the volume of air is large. However, when the piston is compressed, the pressure increases, causing the volume of air to decrease.