The Gay-Lussac's law is a simple gas law.
Simple gas laws apply only for ideal gases.
In other words, the values calculated using simple gas laws give us an approximation of the values that would be measured in reality. As long as the temperature is not too low and the pressure is not too high, these approximate values are very useful for predicting all kinds of situations.
Gay-Lussac's law describes the relationship between the pressure |(P)| and the temperature |(T)| of a gas.
The following images show a certain amount of gas in a container of constant volume.
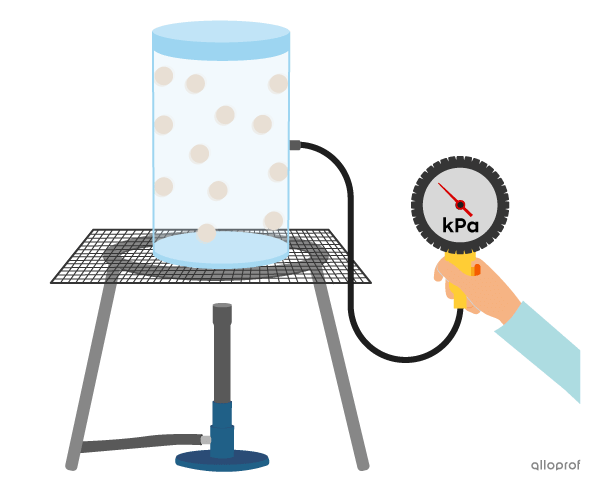
At a given temperature, the particles in the gas exert a certain pressure. This pressure is caused by the particles colliding with the walls of the container.
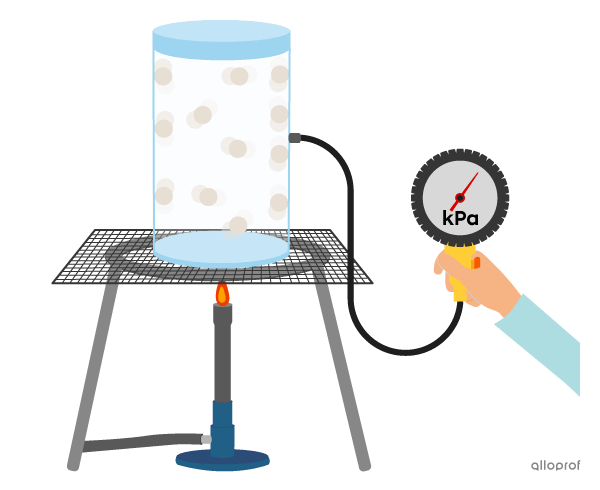
If the temperature of the gas is increased by heating, the kinetic energy of the particles also increases. The particles go into more collisions, so the pressure on the walls of the container increases.
Move the slider up and down to control the gas temperature, then observe the effect on the pressure.
In short, if the number of moles |(n)| and the volume |(V)| are constant, increasing the temperature |(T)| of a gas increases the pressure |(P)| it exerts. On the other hand, when the temperature decreases, the pressure decreases.
Pressure is said to be directly proportional to temperature. This relationship can be expressed using the following formula.
When |V| and |n| are constant:
|P\propto T| or |\dfrac{P}{T}=\text{constant}|
where
|P\ :| pressure often in kilopascals |(\text{kPa})|
|T\ :| temperature in kelvins |(\text{K})|
We can also compare an initial situation and a final situation in which the temperature and pressure of a gas change. The following formula can then be used.
When |V| and |n| are constant:
|\dfrac{P_1}{T_1}=\dfrac{P_2}{T_2}|
where
|P_1\ :| initial pressure often in kilopascals |(\text{kPa})|
|T_1\ :| initial temperature in kelvins |(\text{K})|
|P_2\ :| final pressure often in kilopascals |(\text{kPa})|
|T_2\ :| final temperature kelvins |(\text{K})|
When driving a car, the friction between the road and the tires tends to heat them and the air they contain. This affects the air pressure in the tires.
Before driving the car, we assume that the air temperature in the tire is the same as the outside temperature, i.e. |22{,}00\ ^\circ\text{C}.| Using a manometer, we measure the initial air pressure in one of the tires and obtain |220{,}63\ \text{kPa}.|
After driving for a while, the manometer reads |234{,}42\ \text{kPa}.|
We assume that the amount of air |(n)| in the tire stays the same and we neglect the change in volume |(V),| determine the variation of the air temperature in the tire.
Lorsqu’on roule en voiture, la friction de la route avec les pneus tend à les faire chauffer ainsi que l’air qu’ils renferment. La pression de l’air dans les pneus est donc affectée.
Alors que la voiture n’a pas encore roulé, on considère que la température de l’air dans le pneu est la même que la température extérieure, soit de |22{,}00\ ^\circ\text{C}.| À l’aide d’un manomètre, on mesure la pression initiale de l’air dans l’un des pneus et on obtient |220{,}63\ \text{kPa}.|
Après avoir roulé un certain temps, le manomètre indique |234{,}42\ \text{kPa}.|
En supposant que la quantité d’air |(n)| dans le pneu ne change pas et qu’on néglige la variation du volume |(V),| détermine la variation de la température de l’air du pneu.
First, we need to determine the final temperature |(T_2)| of the air in the tire.
-
Identify the given values and convert the degrees Celsius into kelvins.
|\begin{align}
P_1&=220{.}63\ \text{kPa}\\
T_1&=22{.}00\ ^\circ\text{C}+273{.}15=295{.}15\ \text{K}\\
P_2&=234{.}42\ \text{kPa}\\
T_2&=\ ?
\end{align}|
|n| and |V| are constant.
-
Choose the formula. Because |n| and |V| are constant, we choose:
||\dfrac{P_1}{T_1}=\dfrac{P_2}{T_2}||
-
Isolate |T_2| and plug the given values in the formula.
|\begin{align}
T_2&=\dfrac{P_2\times T_1}{P_1}\\\\
T_2&=\dfrac{234{.}42\ \text{kPa}\times 295{.}15\ \text{K}}{220{.}63\ \text{kPa}}\\\\
T_2&\approx313{.}60\ \text{K}
\end{align}|
-
Now that we know the final temperature |T_2,| we can calculate the temperature variation.
|\begin{align}
\Delta T&=T_2-T_1\\
\Delta T&=313{.}60\ \text{K}-295{.}15\ \text{K}\\
\Delta T&=18{.}45\ \text{K}
\end{align}|
The variation temperature of the air in the tire is |18{.}45\ \text{K}.|
Note: Since a variation of |1\ \text{K}| corresponds to a variation of |1\ ^\circ\text{C},| we can also say that the variation temperature is |18{.}45\ ^\circ\text{C}.|
Louis Joseph Gay-Lussac (1778-1850), a French chemist and physicist, was fascinated by hot air balloons. This passion led him to study gases and their properties. He made numerous ascents to collect air samples and carry out experiments at altitude.
On September 16, 1804, he set the record for the highest hot air balloon ascent by reaching an altitude of 7016 m[1], a record he held until his death.
Source: Niveshkin Nicolay, Shutterstock.com
Barbecue canisters contain propane |(\text{C}_3\text{H}_8)| in a gaseous state. If a propane canister is exposed to a high temperature, the pressure of the propane will increase as stated by Gay-Lussac's law. This high pressure can potentially cause the canister to explode.
This is why gas canisters are usually labelled with a warning to keep them away from sources of extreme heat.
Source: tab62, Shutterstock.com
Video coming soon!
Exercise coming soon!
-
Bataille, X. Louis-Joseph Gay-Lussac : la loi de dilatation des gaz. France Archives. https://francearchives.gouv.fr/fr/pages_histoire/40010