Boyle’s Law is a simple gas law.
Boyle’s law describes the relationship between the pressure |(P)| and the volume |(V)| of a gas.
Consider a constant amount of gas at a given temperature. The gas occupies a certain volume and its particles exert a certain pressure due to their collisions with the walls of the container.
If the gas is compressed so that it takes up a smaller volume, the particles are more squeezed together, resulting in more collisions. The result is greater pressure on the walls of the container.
The height of the piston has an effect on the pressure exerted by the gas on the walls of the container, and its volume. Move the slider up and down to change the height of the piston.
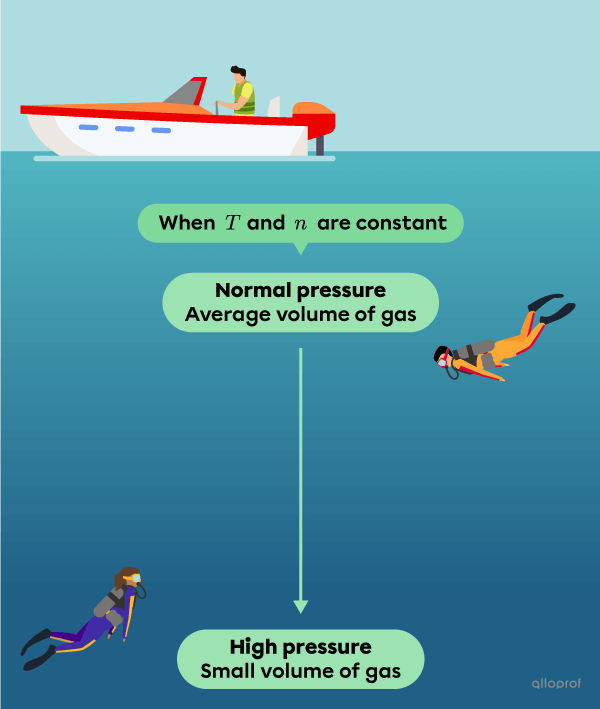
In sum, if the number of moles |(n)| and the temperature |(T)| are constant, decreasing the volume of a gas |(V)| increases the pressure |(P)| it exerts. The other way round, when the volume increases, the pressure decreases.
Pressure is said to be inversely proportional to volume. This relationship can be expressed using the following formula.
When |T| and |n| are constant:
|P\propto\frac{1}{V}| or |PV=\text{constant}|
where
|P :| pressure often in kilopascals |(\text{kPa})|
|V :| volume often in litres |(\text{L})|
We can also compare an initial situation with a final situation in which the volume and pressure of a gas have changed. The following formula can then be used.
When |T| and |n| are constant:
|P_1V_1 = P_2V_2|
where
|P_1 :| initial pressure often in kilopascals |(\text{kPa})|
|V_1 :| initial volume often in litres |(\text{L})|
|P_2 :| final pressure often in kilopascals |(\text{kPa})|
|V_2 :| final volume often in litres |(\text{L})|
Simple gas laws apply only for ideal gases.
In other words, the values calculated using simple gas laws give us an approximation of the values that would be measured in reality. As long as the temperature is not too low and the pressure is not too high, these approximate values are very useful for predicting all kinds of situations.
Robert Boyle was an Anglo-Irish chemist, physicist and theologian who devoted his work to debunking many pseudoscientific myths through his numerous studies of gases. It was in 1662 that he published the mathematical relationship between the pressure and volume of gases, which was not in fact the result of his work. He mentioned the experiments of a scientist called Richard Towneley and referred to it as Towneley's hypothesis. We now know that Towneley did not work alone, but with a physicist called Henry Power. Power had published their work in 1661 in another scientific journal, 1 year before Boyle did [1,2,3,4].
A few years later, Abbé Edme Mariotte, a French botanist, chemist and physicist, discovered this same mathematical relationship. He published his work in his essay De la nature de l'air in 1676[5].
Even if all those people worked on the relationship between the volume and pressure of a gas, only the name of Robert Boyle will be remembered. Boyle’s law could have been called the Towneley-Power hypothesis!
When scuba diving, understanding the consequences of Boyle’s law on respiration is essential for a safe experience.
At the water's surface, air pressure averages |101{.}3\ \text{kPa}|. As a diver goes deeper, the water around him exerts a higher pressure. As Boyle’s law explains, when the pressure of a gas increases, the volume it occupies decreases. A diver's lungs are therefore at risk of collapsing.
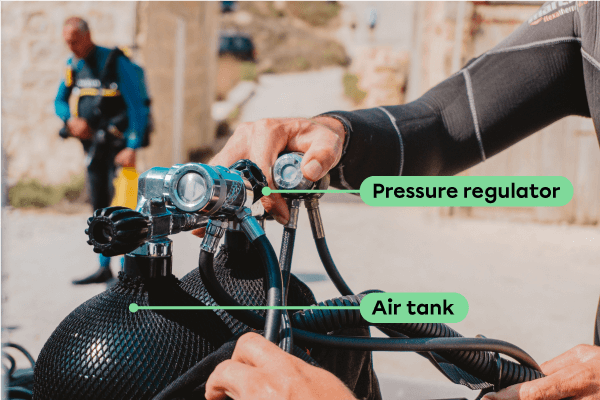
Fortunately, divers are equipped with an air tank fitted with a pressure regulator. This device releases more or less gas for the diver depending on the surrounding pressure.
To avoid crushing the lungs, the pressure regulator increases the flow of air to the lungs as the surrounding pressure increases. In this way, the amount of air inhaled by the lungs increases, which increases the pressure inside the lungs and prevents them from collapsing. This relationship between pressure and the number of moles of gas combines two simple gas laws: Boyle's law and Avogadro's law.
At the surface of the water, a diver has a volume of |5{.}1\ \text{L}| of air in his lungs at a pressure of |101{.}3\ \text{kPa}.|
He then dives to a certain depth. Assuming that the temperature |(T)| and the amount of air |(n)| in his lungs remain the same, what would be the volume of gas in the diver's lungs?
Lorsqu’il se trouve à la surface de l’eau, un plongeur a un volume de |5{,}1\ \text{L}| d’air dans ses poumons à une pression de |101{,}3\ \text{kPa}.|
Il plonge ensuite à une certaine profondeur. La pression environnante est maintenant de |407{,}4\ \text{kPa}.| En supposant que la température |(T)| et la quantité d’air |(n)| dans ses poumons restent les mêmes, quel serait le volume de gaz dans les poumons du plongeur?
-
Identify the given values.
|\begin{align}
P_1 &= 101{.}3\ \text{kPa}\\
V_1 &= 5{.}1\ \text{L}\\
P_2 &= 407{.}4\ \text{kPa}\\
V_2 &= ?
\end{align}|
|n| and |T| are constant. -
Choose the formula. Because |n| and |T| are constant, we choose:
|P_1V_1 = P_2V_2| -
Isolate |V_2| and plug the given values in the formula.
|\begin{align}
V_2 &=\dfrac{P_1V_1}{P_2}\\
V_2 &=\dfrac{101{.}3\ \text{kPa}\times5{.}1\ \text{L}}{407{.}4\ \text{kPa}}\\
V_2 &\approx 1{.}3\ \text{L}
\end{align}|The volume of air in the diver’s lungs would be about |1{.}3\ \text{L}.|
This small volume would collapse the lungs. Fortunately, the diver's air tank is fitted with a pressure regulator that allows this volume to be adjusted to preserve the shape of the lungs.
Exercises coming soon.
1. Webster, Charles (1965). The Discovery of Boyle's Law, and the Concept of the Elasticity of Air in the Seventeenth Century. Archive for History of Exact Sciences. 2 (6). Springer-Verlag: 441–502.
2. Robert Boyle (1662). A Defence Of the Doctrine touching the Spring and Weight Of the Air. p. 60.
3. Henry Power (1663). Experimental Philosophy, in Three Books. London: Printed by T. Roycroft for John Martin and James Allestry: pp. 126–130.
4. Webster, Charles (1963). Richard Towneley and Boyle's Law. Nature, 197 (4864): 226–228.
5. Mariotte, E. (1923). Discours de la nature de l'air, de la végétation des plantes. Nouvelle découverte touchant la vue. Gauthier-Villars, Paris, Réédition.