The mathematical relationship between pressure and the number of moles is a simple gas law.
The relationship between pressure |(P)| and the number of moles |(n)| describes the relationship between those two variables when the volume |(V)| and the temperature |(T)| are constant.
Consider a certain amount of gas in an incompressible container at constant temperature.
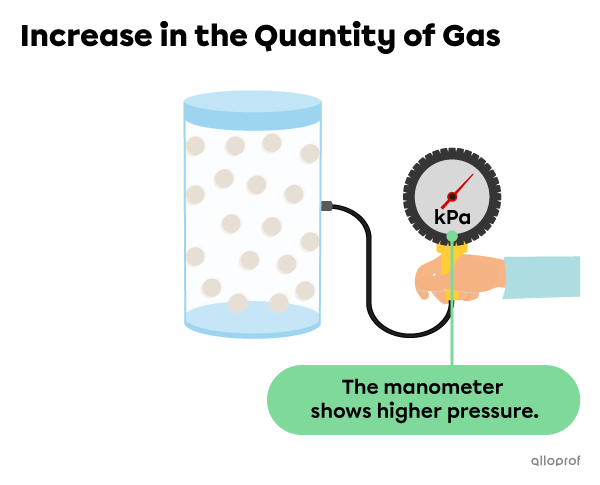
If the amount of gas in the container increases, the particles become more squeezed together, resulting in more collisions. This leads to greater pressure on the walls of the container.
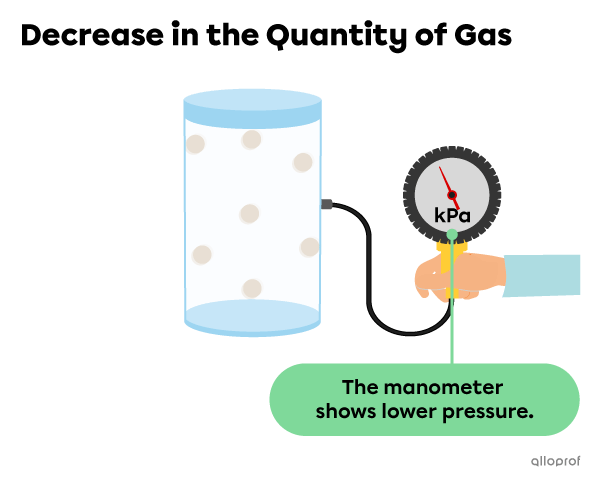
If the amount of gas in the container decreases, the particles become less squeezed together, resulting in less collisions. This leads to lower pressure on the walls of the container.
In short, when the temperature |(T)| and the volume |(V)| are constant, as the amount of gas increases, the pressure increases. Inversely, as the amount of gas decreases, the pressure decreases.
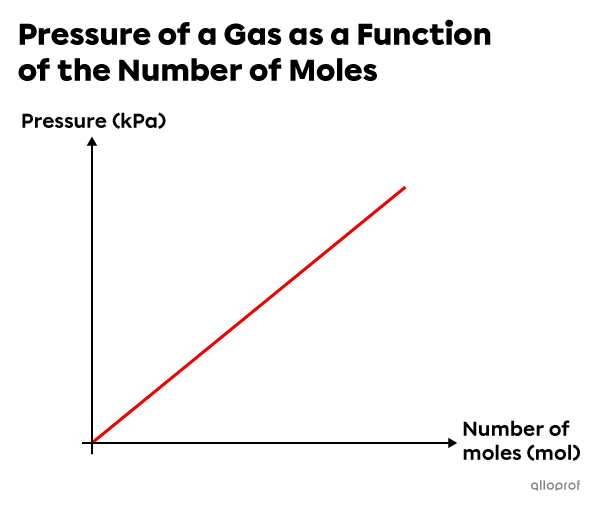
This graph describes the direct variation between the pressure of a gas and the number of moles.
The pressure of a gas is directly proportional to its number of moles. This relationship can be expressed using the following formula.
When |V| and |T| are constant:
|P\propto n| or |\dfrac{P}{n}=\text{constant}|
where
|P : | pressure often in kilopascals |(\text{kPa})|
|n : | quantity of gas in moles |(\text{mol})|
We can also compare an initial situation with a final situation in which the pressure and the number of moles of a gas have changed by using the following formula.
When |V| and |T| are constant:
|\dfrac{P_1}{n_1} = \dfrac{P_2}{n_2}|
where
|P_1 : | initial pressure often kilopascals |(\text{kPa})|
|n_1 : | initial quantity of gas in moles |(\text{mol})|
|P_2 : | final pressure often in kilopascals |(\text{kPa})|
|n_2 : | final quantity of gas in moles |(\text{mol})|
Simple gas laws apply only for ideal gases.
The values calculated using the simple gas laws correspond approximately to the real values, as long as the gas temperature is not too low and the pressure is not too high.
A car tire is punctured. Initially, the air pressure in the tire is |220\ \text{kPa}| and the amount of gas is |0{.}898\ \text{mol}.| After a while, |0{.}122\ \text{mol}| of air escaped from the tire.
What is the final air pressure in the tire?
The volume of the tire and the temperature are assumed to be constant.
A car tire is punctured. Initially, the air pressure in the tire is |220\ \text{kPa}| and the amount of gas is |0{.}898\ \text{mol}.| After a while, |0{.}122\ \text{mol}| of air escaped from the tire.
What is the final air pressure in the tire?
The volume of the tire and the temperature are assumed to be constant.
-
Identify the given values.
|\begin{align}
P_1 &= 220\ \text{kPa}\\
n_1 &= 0{.}898\ \text{mol}\\
P_2 &=?\\
n_2 &= 0{.}898\ \text{mol} - 0{.}122\ \text{mol} = 0{.}776\ \text{mol}
\end{align}|
|V| and |T| are constant.
-
Choose the formula. Since |V| and |T| are constant we choose:
|\dfrac{P_1}{n_1} = \dfrac{P_2}{n_2}|
-
Isolate |P_2| and plug in the given values in the formula.
|\begin{align}
P_2 &=\dfrac{P_1 \times n_2}{n_1}\\
P_2 &=\dfrac{220\ \text{kPa}\times 0{.}776\ \text{mol}}{0{.}898\ \text{mol}}\\
P_2 &\approx 190\ \text{kPa}
\end{align}|
The air pressure in the tire went from |220\ \text{kPa}| to approximately |190\ \text{kPa}.|
Video coming soon!
Exercise coming soon!