Before watching these videos, make sure you're familiar with the formulas for the area and volume of solids. You should also be familiar with general methods of solving equations, such as the balancing equations method. These different notions are essential when you're looking for a missing measurement in solids.
The first video below is aimed at secondary 2 and 3 students, and explains how to find a missing measurement from the area of a solid. The second video is aimed at Secondary 3 students, and explains how to find a missing measurement from the volume of a solid.
The following table is a summary of the various formulas for the area and volume of solids:
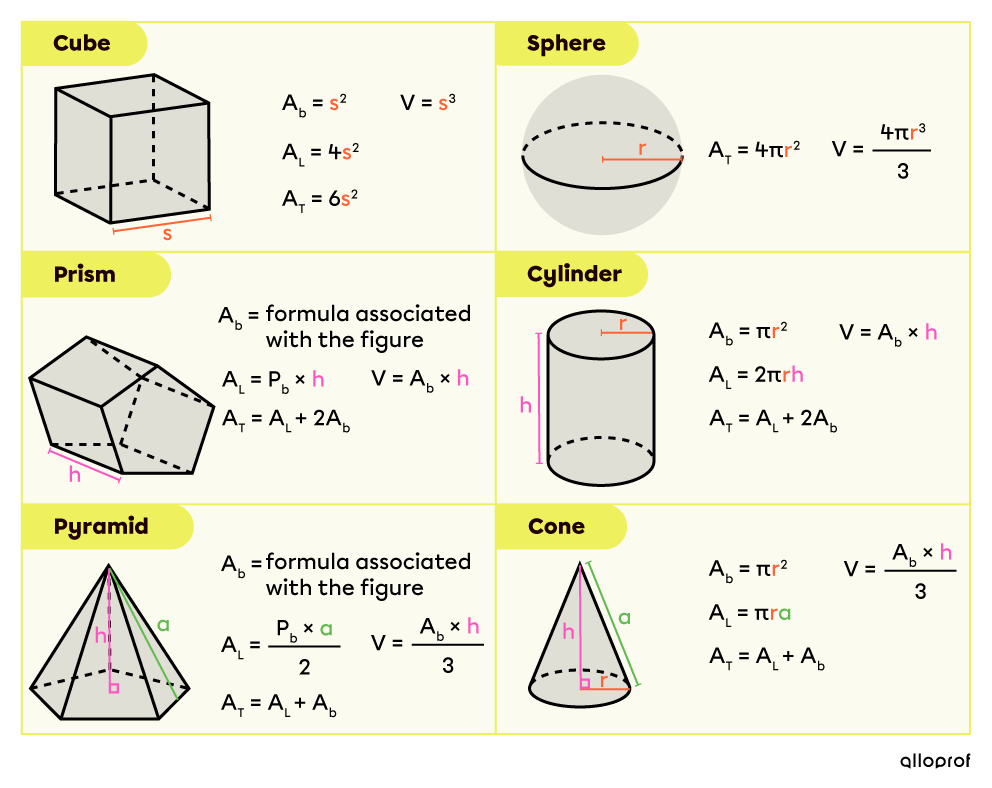
To find a missing measurement in a solid, you need to apply one of the general methods for solving equations to the formula used in the problem. This could be an area or a volume formula.
Here's an example of the steps to follow when you have a problem that involves finding a missing measurement in solids:
The lateral area of a triangular-based regular prism is 4284 mm². If one side of its base measures 8.5 cm, what is the height of the prism?
|
Side of the base: |8.5\ \text{cm}=85\ \text{mm}| |
|---|---|
|
||A_L=P_b\times\boldsymbol{\color{#3A9A38}{h}}|| |
|
||4\ 284=(3\times85)\times\boldsymbol{\color{#3A9A38}{h}}|| |
|
||\begin{align}\dfrac{4284}{\boldsymbol{\color{#EC0000}{255}}}&=\dfrac{255\times\boldsymbol{\color{#3A9A38}{h}}}{\boldsymbol{\color{#EC0000}{255}}}\\16.8\ \text{mm}&=\boldsymbol{\color{#3A9A38}{h}}\end{align}|| |
|
Answer: The height of the prism measures |16.8\ \text{mm}.| |
|