Gravitational force is the physical reaction that causes mutual attraction between two bodies.
In general, this attraction takes place between an object and a celestial body such as the Earth or Moon. This force produces a gravitational acceleration
The Moon revolves around the Earth because it is attracted to it.
When a person jumps rope, he or she falls back to the ground, because the Earth exerts an attraction on that person.
It's important to specify that gravity is always exerted between two objects. If object A attracts object B, then object B also attracts object A with the same force. This is the principle behind Newton's third law.
We use the following formula to find the gravitational force of a mass.
|F_{g} = m \times g|
where
|F_{g}| represents the gravitational force in Newtons |\small (\text {N})|
|m| represents the mass of the body in kilograms |\small (\text {kg})|
|g| represents the intensity of the gravitational field in Newtons par kilogramme |\small (\text {N/kg})|
What is the gravitational force exerted on an apple weighing |\small \text {50 g}| on the Earth and on the Moon?
It's important to convert mass into kilograms.
|m = 50 \: \text {g} = 0.05 \: \text {kg}|
To calculate the gravitational force on the Earth:
||\begin{align} F_{g} = m \times g \quad \Rightarrow \quad
F_{g} &= 0.05 \: \text {kg} \times 9.8 \: \text {N/kg}\\
&= 0.49 \: \text {N} \end{align}||
To calculate the gravitational force on the Moon:
||\begin{align} F_{g} = m \times g \quad \Rightarrow \quad
F_{g} &= 0.05 \: \text {kg} \times 1.6 \: \text {N/kg}\\
&= 0.08 \: \text {N} \end{align}||
When a body is in free fall, it heads towards the ground or, more precisely, towards the center of the Earth. It must follow this trajectory, as there is a force of attraction, the gravitational force, which brings the body closer to the center of the other object attracting it. In the absence of air resistance, free fall will take place with an acceleration equivalent to the Earth's gravitational acceleration, i.e. |\small 9.8 \: \text {m/s}^2|.
The weight of an object is the measure of the gravitational force exerted by the Earth on an object.
The gravitational force exerted by an object is therefore equal to the weight of that object. Thus, two objects of identical mass will necessarily have the same weight if they are both located in the same place. However, if one of these objects were left on Earth while the other was brought to the Moon, the weight would vary, as the gravitational force would no longer be the same. The mass, however, would not change, because, by definition, mass represents the amount of matter that makes up an object.
What is the gravitational force with which the Earth attracts an object of |\small \text {70 kg}| ? What is the mass and weight of this object on the Moon?
To determine the gravitational force on the Earth:
||\begin{align} F_{g} = m \times g \quad \Rightarrow \quad
F_{g} &= 70 \: \text {kg} \times 9.8 \: \text {N/kg}\\
&= 686 \: \text {N} \end{align}||
The mass of the object on the Moon will not change. No matter where the object is located, the amount of matter will be the same. The mass on the Moon is therefore |\small \text {70 kg}|.
To determine the gravitational force on the Moon:
||\begin{align} F_{g} = m \times g \quad \Rightarrow \quad
F_{g} &= 70 \: \text {kg} \times 1.6 \: \text {N/kg}\\
&= 112 \: \text {N} \end{align}||
The law of universal gravitation is an equation that calculates the gravitational force (|F_{g}|) with which two bodies are attracted to each other.
|\displaystyle F_{g} = \frac{G \cdot m_{1} \cdot m_{2}}{r^{2}}|
where
|F_{g}| represents the force of attraction between the bodies |\small (\text {N})|
|G| represents the universal gravitational constant |\small \left( 6.67 \times 10^{-11} \displaystyle \frac {\text {N} \cdot \text {m}^{2}}{\text {kg}^{2}} \right)|
|m_{1}| represents the mass of the first object |\small (\text {kg})|
|m_{2}| represents the mass of the second object |\small (\text {kg})|
|r| represents the distance separating the two objects |\small (\text {m})|
Planet Earth has a mass of about |\small 5.98 \times 10^{24} \: \text {kg}| and rotates at an average distance of |\small 1.50 \times 10^{8} \: \text {km}| from the Sun, whose mass is about |\small 1.99 \times 10^{30} \: \text {kg}|. What is the force that allows the Earth to continue its revolution around the Sun?
The information known in this problem is as follows.
||\begin{align}G &= 6.67 \times 10^{-11} \: \frac {\text {N} \cdot \text {m}^{2}}{\text {kg}^{2}} &m_{\text {Earth}} &= 5.98 \times 10^{24} \: \text {kg} \\
m_{\text {Sun}} &= 1.99\times 10^{30} \: \text {kg} &r_{\text {Earth-Sun}} &= 1.50 \times 10^{11} \: \text {m}\end{align}||
Using the law of universal gravitation, the force can be measured.
||\begin{align} \displaystyle F_{g} &= \frac{G \cdot m_{\text {Earth}} \cdot m_{\text {Sun}}}{r^{2}}\\
&= \frac{{6.67 \times 10^{-11} \displaystyle \frac {\text {N} \cdot \text {m}^{2}}{\text {kg}^{2}} \cdot 5.98 \times 10^{24} \space \text {kg} \cdot 1.99 \times 10^{30} \space \text {kg}}}{(1.50 \times 10^{11} \space \text {m})^{2}} \\
&= 3.53 \times 10^{22} \space \text {N} \end{align}||
A rock weighing |\small \text {300 kg}| is found on the surface of the Moon, whose mass is |\small 7.35 \times 10^{22} \: \text {kg}|. . If the Moon has a radius of about |\small 1.74 \times 10^{6} \: \text {m}|, what force allows the rock to remain on the Moon's surface?
The difficulty in this problem is to estimate the distance between the rock and the Moon. It's wrong to assume that the distance is zero, since we have to use the center of a mass (or the center of the Moon in our case) as a reference point for evaluating distances. The distance separating this object from the center of the Moon will therefore be the lunar radius.
The information known in this problem is as follows.
||\begin{align}G &= 6.67 \times 10^{-11} \: \frac {\text {N} \cdot \text {m}^{2}}{\text {kg}^{2}} &m_{\text {rock}} &= 300 \: \text {kg}\\
m_{\text {Moon}} &= 7.35 \times 10^{22} \: \text {kg} &r_{\text {rock-Moon}} &= 1.74\times 10^{6} \: \text {m}\end{align}||
The law of universal gravitation can be used to calculate the force exerted between the Moon and the rock.
||\begin{align} \displaystyle F_{g} &= \frac{G \cdot m_{\text {Earth}} \cdot m_{\text {Sun}}}{r^{2}} \\
&= \frac{{6.67 \times 10^{-11} \displaystyle \frac {\text {N} \cdot \text {m}^{2}}{\text {kg}^{2}} \cdot 300 \: \text {kg} \cdot 7.35 \times 10^{22} \: \text {kg}}}{(1.74 \times 10^{6} \: \text {m})^{2}} \\
&= 486 \: \text {N} \end{align}||
When an object is on an inclined plane, part of the gravitational force runs parallel to the object's motion. This force is sometimes called the plane force or x-force. To find this force, we need to use trigonometric formulas in a right-angled triangle.
What is the gravitational force parallel to the displacement of a mass of |\small \text {100 kg}| ?
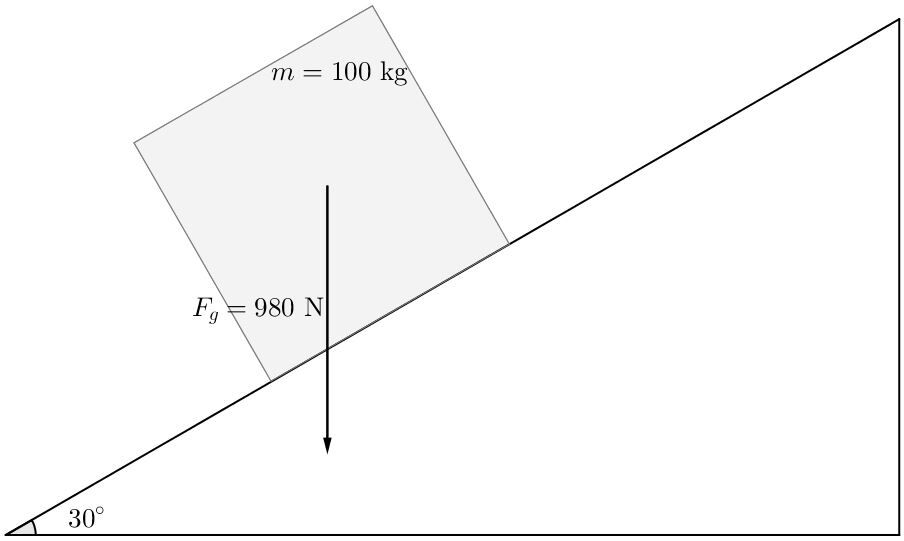
The gravitational force is always directed towards the center of the Earth (towards the ground). It is perpendicular to the surface of the ground.
The normal force is perpendicular to the slope in the plane. If we extend the normal downwards, the angle formed between it and the gravitational force is the same as the angle of the slope.
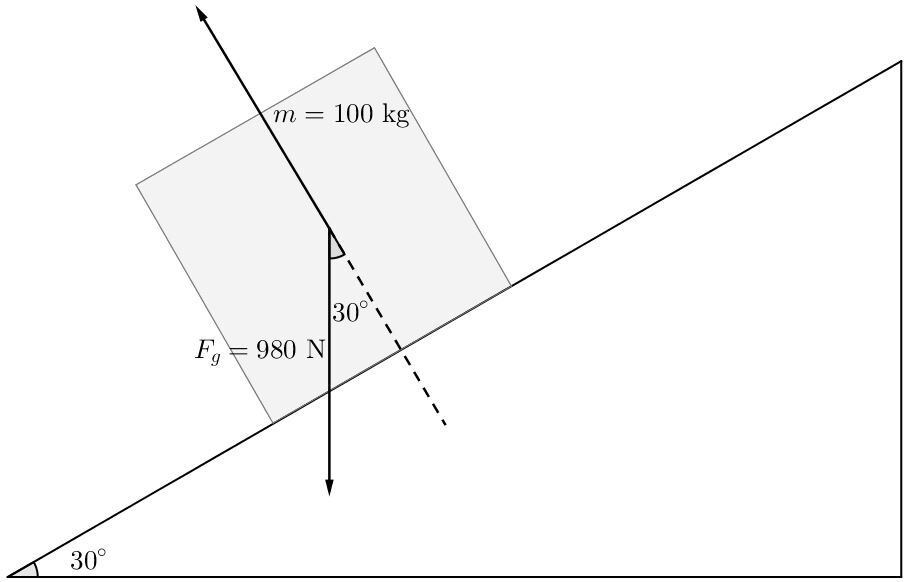
The gravitational force parallel to the displacement will be the force opposite to the angle.
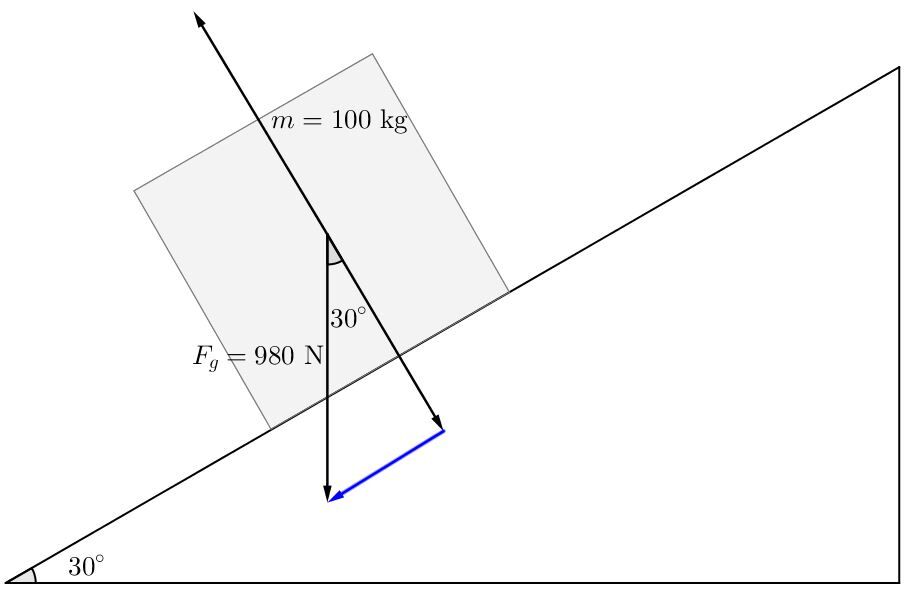
Using trigonometric ratios, it is possible to determine the value of the force parallel to the displacement.
||\begin{align} \sin \Theta = \frac {\text {F}_\text{x}}{\text {F}_\text{g}}
\quad \Rightarrow \quad
\text {F}_\text{x} &= \sin \Theta \times \text {F}_\text{g} \\
&= \sin \: 30^{\circ} \times 980 \space \text {N}\\
&= 490 \: \text {N} \end{align}||
Isaac Newton (1643-1727) was a British physicist renowned for establishing the three universal laws of motion in 1688. These three laws, known as Newton's laws, formed the basis of classical mechanics.
- Newton's first law describes the principle of inertia, i.e. the principle according to which a body retains its state of motion provided no external force acts on it. The application of a force will alter its state of motion, causing it to undergo a vector acceleration (change in velocity and/or orientation).
- Newton's second law describes what happens when a force is exerted on an object. It also provides a mathematical formula for calculating the intensity of that force.
- Newton's third law states that if a force is applied to an object, there is a force of the same magnitude and direction, but in the opposite direction to the first force.
In the following pages, each of these laws will be explained in detail:


