-
Work is a transfer of energy associated with the displacement of an object.
-
The effective force is the component of a force responsible for the displacement of an object.
-
Displacement is the distance from the starting point to the final point of an object.
The work done on an object varies depending on the displacement of this object and the effective force applied to it:
-
the greater the displacement, the greater the work done on this object;
-
the greater the effective force, the greater the work done on the object.
By convention, the unit of measurement of work is the joule (|\text{J}|): 1 joule is the amount of energy needed to displace an object over a distance of 1 metre (|\text{m}|) using an effective force of 1 newton (|\text{N}|).
The following mathematical formula is used to calculate the work exerted on an object.
|W = F_{eff} \cdot \Delta s|
where
|W| represents work (|\text{J}|)
|F_{eff}| represents the effective force (|\text{N}|)
|\Delta s| represents the displacement of the object (|\text{m}|)
The effective force is the component of the applied force that is parallel to the displacement of an object. The effective force has the same direction as the displacement.
It can also be identified as “the force parallel to displacement”.
A crane lifts a beam over a distance of |20\ \text {m}| in height. To achieve this, the crane applies an upward constant tension of |1\ 000\ \text {N}|.
What is the work done by the crane to displace the beam?
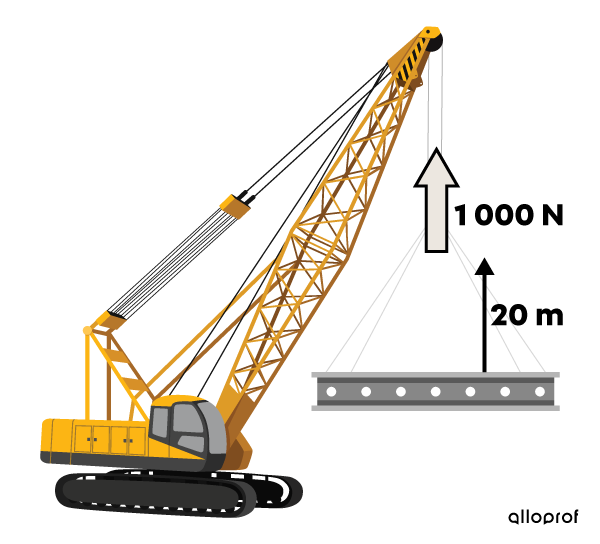
The applied force and the displacement of the beam are parallel (up). The work can be calculated as follows.
First, identify the given values.
|\begin{align} &W=?\ \text{J} &F_{eff}= 1\ 000\ \text{N} &\\ &\Delta s= 20\ \text{m} \end{align}|
Next, identify the formula to use and insert the values.
|\begin{align} W&=F_{eff}\cdot \Delta s\\ W&= 1\ 000\ \text{N} \times 20 \ \text{m}\\ W&=20\ 000\ \text{J}\end{align}|
The crane performs |20\ 000\ \text{J}| (|20\ \text{kJ}|) of work on the beam.
A person horizontally applies a force of |100\ \text{N}| on a trolley loaded with cast iron discs. Despite this effort, the trolley remains motionless.
How much work is the person doing on the trolley?
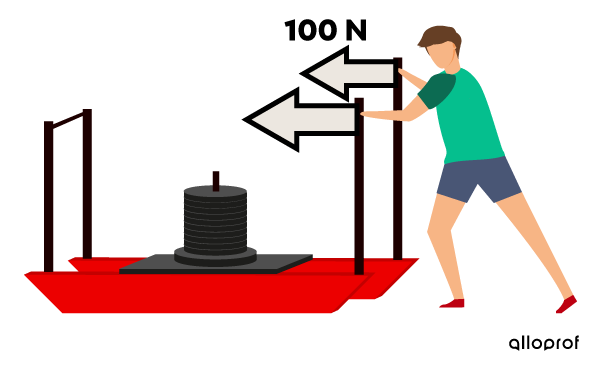
As the work corresponds to the energy provided to displace an object and the trolley undergoes no displacement, the work will be zero.
Here is the process.
First, identify the given values.
|\begin{align} &W=?\ \text{J} &F_{eff}= 100\ \text{N} &\\ &\Delta s= 0\ \text{m} \end{align}|
Next, identify the formula and insert the values.
|\begin{align} W&=F_{eff}\cdot \Delta s\\ W&= 100\ \text{N} \times 0 \ \text{m}\\ W&=0\ \text{J}\end{align}|
The person performs an estimated |0\ \text {J}| of work on the trolley.
The calculation of work is obtained by a scalar product, that is the multiplication of two vectors. This involves a force vector and a displacement vector.
Furthermore, when the force applied to an object is not parallel to the displacement, the effective force must first be determined using a trigonometric ratio in a right-angled triangle before calculating the work.
A person pulls a box over a distance of |5\ \text{m}| by applying a force of |40\ \text {N}| at an angle of |30^\circ| relative to the horizontal.
What is the work performed by the person on the box?
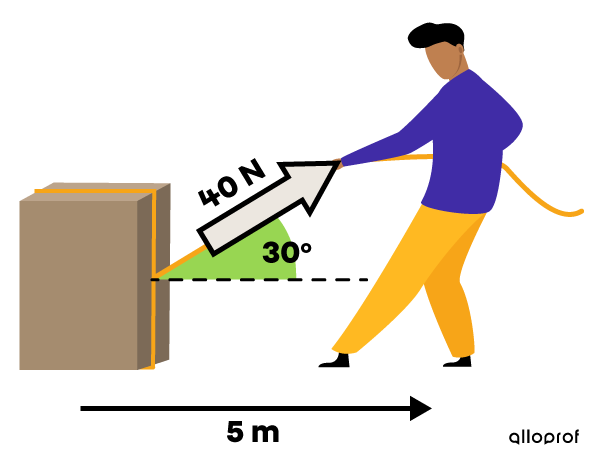
Since the force applied is not parallel to the displacement of the box, the effective force must first be determined using a trigonometric ratio.
-
Draw a right-angled triangle with the hypotenuse corresponding to the force applied to the box (|40\ \text{N}|). The side adjacent to the |30^\circ| angle represents the effective force (in red in the diagram).
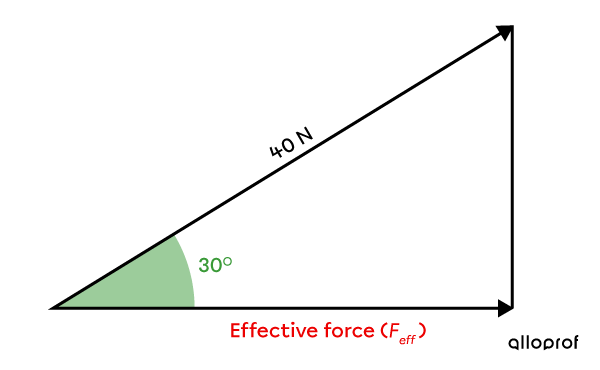
-
Determine the magnitude of the effective force.
First, identify the given values. Next, identify the formula to use and insert the values.
|\begin{align} &\theta=30^\circ\\ &\text{adjacent side (effective force)}=?\ \text{N}\\ &\text{hypotenuse (applied force)}=40\ \text{N} \end{align}| |\begin{align}cos\ \theta&=\frac{\text{ adjacent side}}{\text{hypotenuse}}\\ cos\ 30^{\circ}&=\frac{\color{red}{F_{eff}}}{40\ \text{N}}\\ \color{red}{F_{eff}}&=cos\ 30^{\circ}\times 40\ \text{N}\\\color{red}{F_{eff}}&\approx 34{.}6\ \text{N}\end{align}|
The effective force is approximately |34{.}6\ \text{N}|.
-
Calculate the work.
First, identify the known values. Next, identify the formula to use and replace the variables.
|\begin{align}&W=?\ \text{J} &F_{eff}=34{.}6\ \text{N}\\&\Delta s=5\ \text{m}\end{align}|
|\begin{align}W&=F_{eff}\cdot\Delta s\\W&=34{.}6\ \text{N} \times 5\ \text{m}\\W&=173\ \text{J}\end{align}|
A work of |\text {173 J}| is performed on the box.
When we perform work, we use energy. According to the law of conservation of energy, energy is neither lost nor created, it is transferred or transformed. Thus, the work experienced by an object is equivalent to its change in energy.
The mathematical relationship between work and energy change is as follows:
|W=\Delta E|
where
|W| represents work |\text {(J)}|
|\Delta E| represents the change in energy (kinetic or potential) |\text {(J)}|
A person applies sufficient force to set a grocery cart in motion at a speed of |1{.}5\ \text {m/s}|.
The cart and its contents have a total mass of |15\ \text{kg}|.
What is the work performed by the person to displace the cart at a speed of |1{.}5\ \text{m/s}|?

When the basket starts moving, it acquires kinetic energy, which depends on its mass and speed. The change in energy of the basket |\Delta E| is therefore equivalent to the kinetic energy acquired when it reaches its final speed ( |E_{k_{\text{final}}}|).
||\Delta E=E_{k_{\text{final}}}||
Just find the kinetic energy of the grocery cart. It is equivalent to the work exerted on the cart.
First, identify the given values. Next, identify the formula to use and replace the variables.
|\begin{align}
&E_{k_{\text{final}}}=?\ \text{J}\\
&m=15\ \text{kg}\\
&v=1{.}5\ \text{m/s}
\end{align}|
|\begin{align} E_{k_{\text{final}}} &= \dfrac {1}{2} m v^{2} \\ E_{k_{\text{final}}}&= \dfrac {1}{2} \times 15\ \text {kg} \times \text {(1.5 m/s)}^{2} \\ E_{k_{\text{final}}}&\approx 16{.}9\ \text {J} \\ \end{align}|
Finally, the work is equivalent to the change in kinetic energy.
||\begin{align}
W &=\Delta E \\
W &\approx 16{.}9\ \text {J}
\end{align}||
The work provided by the person is approximately |16{.}9\ \text {J}.|