To model the sine function, it is critical to understand the effects of the various parameters.
Then, proceed step by step in order to solve the problem.
In some cities, such as Niagara Falls or London, a Ferris wheel is installed to offer a panoramic view to tourists.
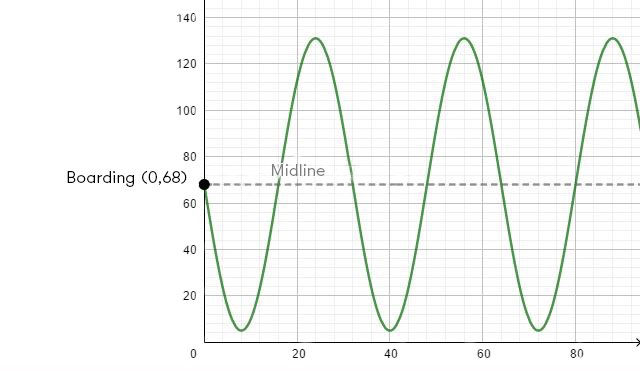
To climb inside the Ferris wheel, there is a platform installed at a height equivalent to half of the height of the Ferris wheel. To avoid collisions with bystanders, the lowest point of the Ferris wheel is |5 \ \text{m}| above the ground. At the summit, the passengers find themselves at an impressive height of |131\ \text{m}.| As soon as the passengers board, the carriage where the passengers are seated descends.
Given that the view becomes particularly spectacular from an altitude of |120\ \text{m}| and the Ferris wheel takes |32\ \text{minutes}| to complete a full turn, how long will tourists be in awe of the scenery?
1) Use the equation of the sine function to determine the value of |a|
Based on the context, the maximum height reached by the wheel is |131\ \text{m}| and the lowest point is |5 \ \text{m}.| So: ||\vert a \vert = \frac{max \ - \ min}{2} = \frac{131 - 5}{2} = 63||Since the Ferris wheel starts by departing towards the ground, the value of the parameter |a| will be negative: |a = -63.|
2) Use the equation of the sine function to determine the value of |(h,k)|
Since the passengers board halfway up the height of the Ferris wheel, so in the middle of the Ferris wheel’s cycle, then: ||\begin{align}
h & = 0 \\
k & = 5 + 63 \\
& = 68 \end{align}||So, |(h,k) = (0,68).|
3) Use the equation of the sine function to determine the value of |b|
By definition, |b = \dfrac{2 \pi}{\text{period}} = \dfrac{2 \pi}{32} = \dfrac{\pi}{16}.|
4) Create and solve the equation related to the situation
Since we want to reach the top of the Ferris wheel, solve using the following. ||\begin{align}
f(x) & = -63 \sin \left(\frac{\pi}{16} (x-0)\right) + 68 \\
120 & = -63 \sin \left(\frac{\pi}{16} x \right) + 68 \\
52 & = -63 \sin \left(\frac{\pi}{16} x \right) \\
-\frac{52}{63} & = \sin \left(\frac{\pi}{16} x \right)\\
-0.971 & \approx \frac{\pi}{16} x \end{align}||
5) Find the first possible |x| value
To obtain the first positive value, add |2 \pi | to the value found, since the period of the basic sine function is |2 \pi.| ||\begin{align}
-0.971 &= -0.971 + 2 \pi \\
&\approx 5{,}309 \\\\
\Rightarrow\ 5.309 &\approx \frac{\pi}{16} x_1 \\
27.05 &\approx x_1 \end{align}||
6) Find the second possible |x| value
According to the properties of the unit circle, there is a second point that is at the same height as the first. Find the point with the following calculation. ||\begin{align}
\pi - \text{first value in radians} & = \pi - -0.971 \\
& \approx 4.111 \end{align}|| Finally, solve the equation again with this new value. ||\begin{align} 4.111 & = \dfrac{\pi}{16} x_2 \\ 20.95 & \approx x_2 \end{align}||
7) Interpret the answer
This means that the view will be spectacular for about |27.05 - 20.95 = 6.10\ \text{minutes}.|
To understand the solution above, the concept sheet on solving trigonometric equations and inequalities is a very useful tool.
The sine function was used here because the situation began on the midline.
If the starting point was a maximum or a minimum, the cosine function would have been a better choice.