Thales' theorem is very useful when finding one or more missing measurements in a figure formed by intersecting transversals that cross parallel lines.
If, in a figure…
-
lines |\color{#3a9a38}{BD}| and |\color{#3b87cd}{CE}| are transversals that intersect at |A,|
-
lines |\color{#ec0000}{BC}| and |\color{#ec0000}{DE}| are parallel,
then the following proportion exists:
||\color{#3a9a38}{\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}}}=\color{#3b87cd}{\dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}}}= \color{#ec0000}{\dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}}||
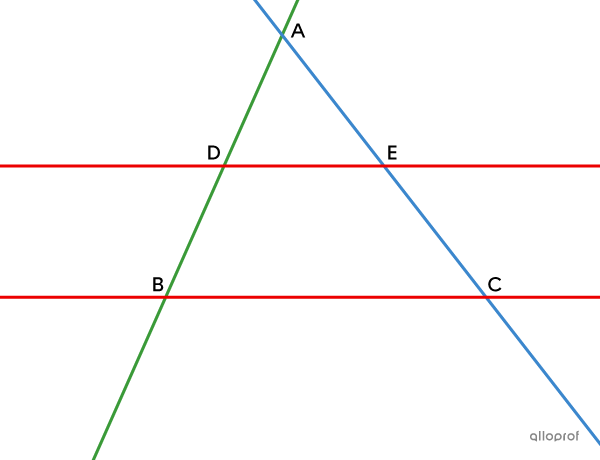
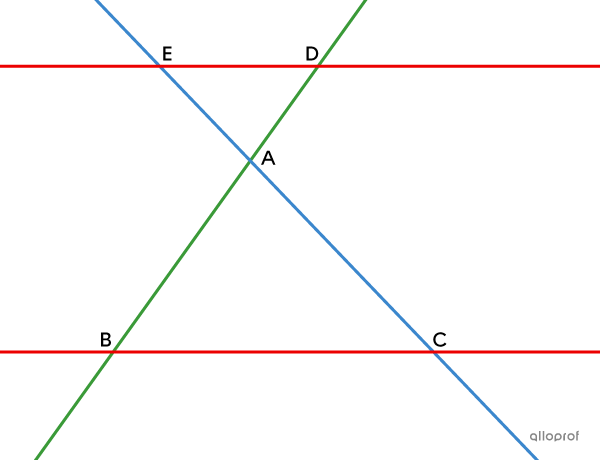
Note: Thales' theorem applies regardless of whether the transversals |(\color{#3b87cd}{EC}| and |\color{#3a9a38}{BD})| intersect inside or outside the parallel lines |\color{#ec0000}{ED}| and |\color{#ec0000}{BC}).|
Thales' theorem originates from Thales of Miletus, an ancient scholar who lived around 600 BC. Here is the method he used to calculate the height of certain Egyptian pyramids using the shadow they cast.
To measure the height of the pyramid, a person must stand so that his or her shadow is aligned with the shadow of the pyramid. This produces two similar triangles. Then just establish the correct proportion and solve it.
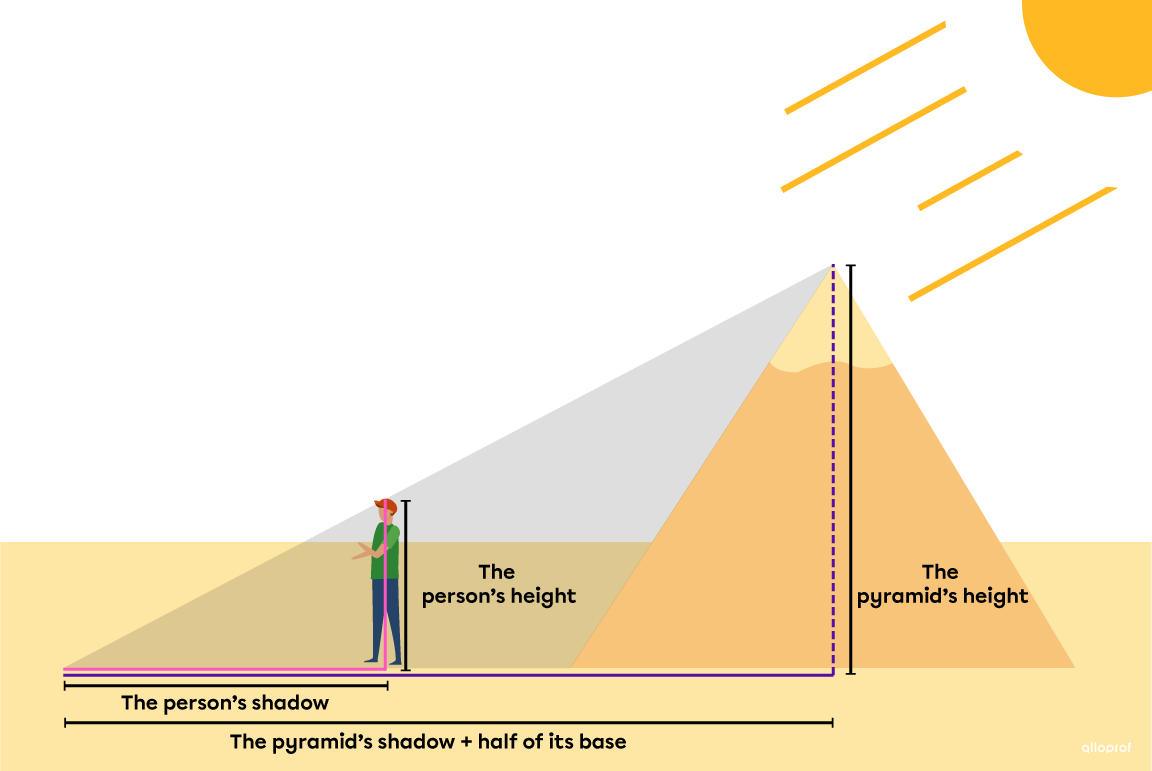
According to Thales' theorem, since the height of the person and the height of the pyramid are parallel segments, we can use the following ratio.
||\dfrac{\begin{gather}\text{Height of}\\\text{the person}\end{gather}}{\begin{gather}\text{Height of}\\\text{the pyramid}\end{gather}}=\dfrac{\begin{gather}\text{Shadow of}\\\text{the person}\end{gather}}{\begin{gather}\text{Shadow of the pyramid}\\\text{+ half of its base}\end{gather}}||
Using this method, you can find the height of anything, such as the height of a house or school. Give it a try!
Si, dans une figure…
-
… les droites |\color{#3a9a38}{BD}| et |\color{#3b87cd}{CE}| sont sécantes en |A;|
-
… les droites |\color{#ec0000}{BC}| et |\color{#ec0000}{DE}| sont parallèles,
alors, on a la proportion suivante.||\color{#3a9a38}{\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}}}=\color{#3b87cd}{\dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}}}= \color{#ec0000}{\dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}}||
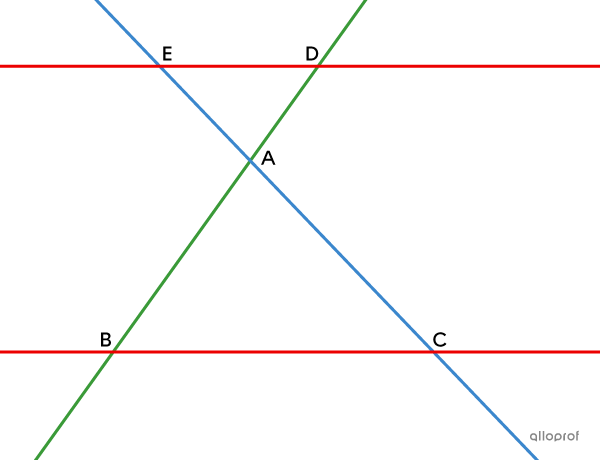
Remarque : Le théorème de Thalès s'applique peu importe si les sécantes |(\color{#3b87cd}{EC}| et |\color{#3a9a38}{BD})| se croisent à l’extérieur ou à l’intérieur des parallèles |(\color{#ec0000}{ED}| et |\color{#ec0000}{BC}).|
Thales' theorem is based on the proportions of corresponding sides in similar triangles. It is proven by showing that |\triangle ABC \sim \triangle ADE| using the minimum condition for similarity AA.
Hypothesis
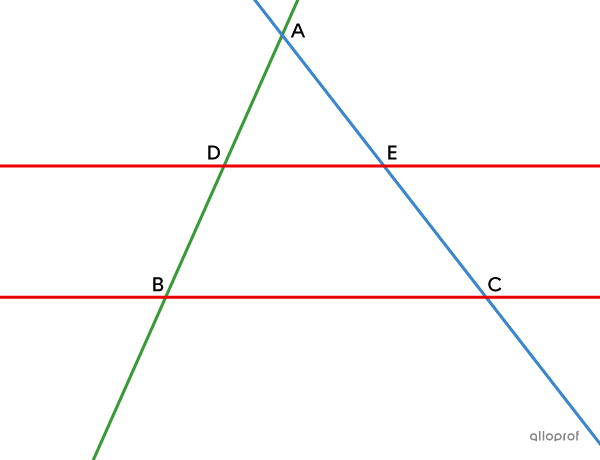
Consider distinct parallel lines |\color{#ec0000}{BC}| and |\color{#ec0000}{DE},| as well as intersecting transversals |\color{#3a9a38}{AB}| and |\color{#3b87cd}{AC}.|
Conclusion
||\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}} = \dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}} = \dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}||
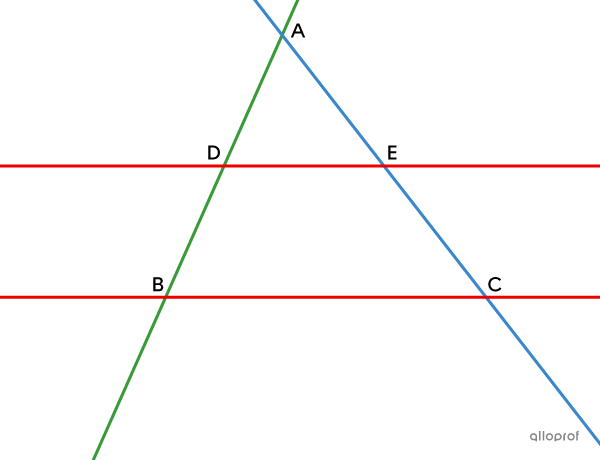
Proof
| Statement | Justification | ||
|---|---|---|---|
|
1 |
Angles |DAE| and |BAC| are congruent.||\angle DAE\cong\angle BAC|| |
A |
It is an angle common to both triangles. |
|
2 |
Angles |ADE| and |ABC| are congruent.||\angle ADE\cong\angle ABC|| |
A |
Corresponding angles formed by parallel lines |(\color{#ec0000}{BC}| and |\color{#ec0000}{DE})|, cut by a transversal |(\color{#3a9a38}{AB})| are congruent. |
|
3 |
Triangles |ABC| and |ADE| are similar.||\triangle ABC\sim\triangle ADE|| |
They satisfy the minimum condition AA: two triangles are similar if they have 2 pairs of corresponding congruent angles. |
|
|
4 |
||\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}} = \dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}} = \dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}|| |
The corresponding sides of similar triangles are proportional. |
|
The proof of Thales' theorem when the intersection point |(A)| of the transversals is situated inside the 2 parallel lines is also established using the minimum condition of similarity AA.
Hypothesis
Consider the distinct parallel lines |\color{#ec0000}{BC}| and |\color{#ec0000}{DE},| as well as the intersecting transversals |\color{#3a9a38}{AB}| and |\color{#3b87cd}{AC}.|
Conclusion
||\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}} = \dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}} = \dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}||
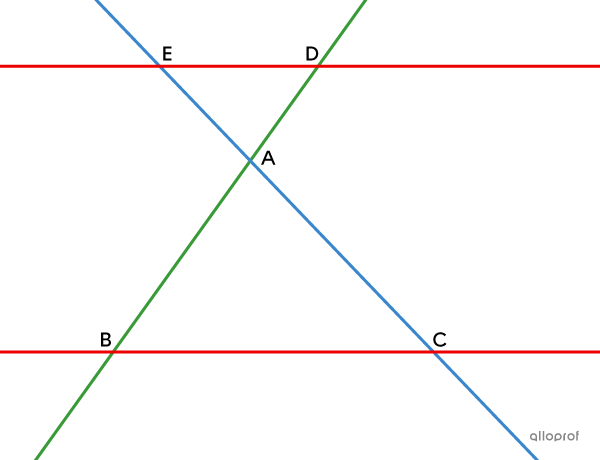
Proof
| Statement | Justification | ||
|---|---|---|---|
|
1 |
Angles |DAE| and |BAC| are congruent.||\angle DAE\cong\angle BAC|| |
A |
They are vertically opposite angles. |
|
2 |
Angles |ADE| and |ABC| are congruent.||\angle ADE\cong\angle ABC|| |
A |
Alternate-interior angles formed by parallel lines |(\color{#ec0000}{BC}| and |\color{#ec0000}{DE}),| cut by a transversal |(\color{#3a9a38}{BD}),| are congruent. |
|
3 |
Triangles |ABC| and |ADE| are similar.||\triangle ABC\sim\triangle ADE|| |
They satisfy the minimum condition AA: triangles are similar if they have 2 pairs of corresponding congruent angles. |
|
|
4 |
||\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}} = \dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}} = \dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}|| |
The corresponding sides of similar triangles are proportional. |
|
Once we know that there are 2 parallel lines cut by 2 intersecting transversals, we can use Thales' theorem to solve the proportion in the triangles without having to prove that they are similar.
Find the missing measurement, given that lines |\color{#ec0000}{ED}| and |\color{#ec0000}{BC}| are parallel.
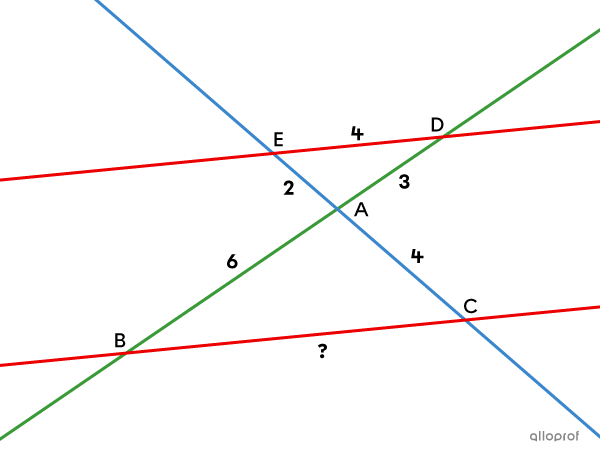
According to Thales’ theorem, since lines |\color{#ec0000}{ED}| and |\color{#ec0000}{BC}| are parallel, the following proportion exists:
||\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}}= \dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}}= \dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}||
To find |\text{m}\overline{BC},| we can solve the following equation:
||\begin{align}\dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}}&= \dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}\\ \dfrac{2}{4}&=\dfrac{4}{\text{m}\overline{BC}}\\\\ \text{m}\overline{BC}&=\dfrac{4\times 4}{2}\\&=8\end{align}||
Answer: |\text{m}\overline{BC} = 8\ \text{units}|
In the situations associated with Thales' theorem, if the lines are parallel, then a proportion can be derived. However, it is also possible to apply the opposite reasoning, in other words, if the proportion is valid, then we can conclude that the lines are parallel. This is called the converse of Thales' theorem.
Hypothesis
Transversal lines |\color{#3a9a38}{BD}| and |\color{#3b87cd}{CE},| intersecting at |A,| are placed so that the following proportion is respected.
||\color{#3a9a38}{\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}}}=\color{#3b87cd}{\dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}}}= \color{#ec0000}{\dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}}||
Conclusion
Lines |\color{#ec0000}{BC}| and |\color{#ec0000}{DE}| are parallel.
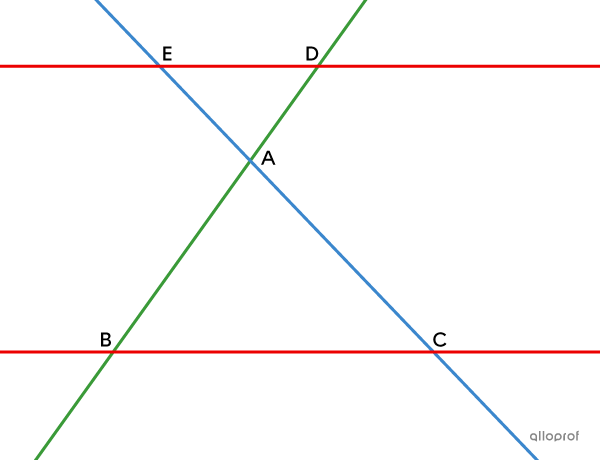
Proof
| Statement | Justification | ||
|---|---|---|---|
|
1 |
Triangles |ABC| and |ADE| are similar. ||\triangle ABC \sim \triangle ADE|| |
By hypothesis, the corresponding sides of triangles |ABC| and |ADE| are proportional. They therefore respect the minimum condition SSS: triangles are similar if the measures of their corresponding sides are proportional. |
|
|
2 |
Angles |ADE| and |ABC| are congruent. ||\angle ADE\cong\angle ABC|| |
The corresponding angles of similar triangles are congruent. |
|
|
3 |
Lines |\color{#ec0000}{BC}| and |\color{#ec0000}{DE}| are parallel. |
Two lines |(\color{#ec0000}{BC}| and |\color{#ec0000}{DE}),| cut by a transversal |(\color{#3a9a38}{BD}),| form congruent alternate-interior angles |(\angle ADE| and |\angle ABC),| if and only if these lines are parallel. |
|
Given that transversal lines |\color{#3b87cd}{EC}| and |\color{#3a9a38}{BD}| intersect at |A,| prove that lines |\color{#ec0000}{ED}| and |\color{#ec0000}{BC}| are parallel.
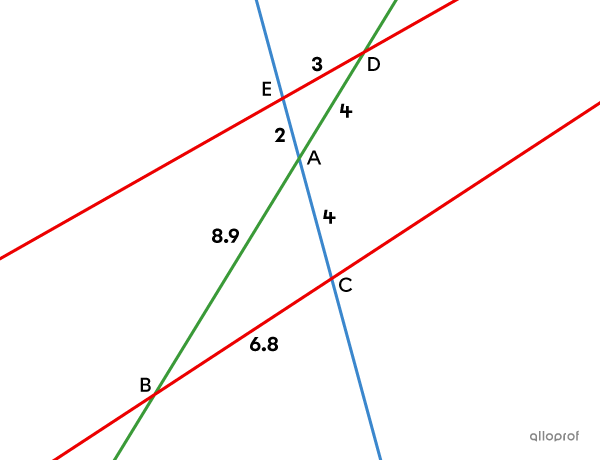
We must verify if the proportion of Thales' theorem between the corresponding sides of the triangles |ADE| and |ABC| is true. If we show that the 3 ratios are equivalent, then we can conclude that the lines are parallel.
||\begin{align}\color{#3a9a38}{\dfrac{\text{m}\overline{AD}}{\text{m}\overline{AB}}}&=\color{#3b87cd}{\dfrac{\text{m}\overline{AE}}{\text{m}\overline{AC}}}= \color{#ec0000}{\dfrac{\text{m}\overline{DE}}{\text{m}\overline{BC}}}\\ \color{#3a9a38}{\dfrac{4}{8.9}}\quad &\neq\quad\color{#3b87cd}{\dfrac{2}{4}}\quad \neq\quad\color{#ec0000}{\dfrac{3}{6.8}}\end{align}||
Conclusion: Since the 3 ratios are not equal, lines |\color{#ec0000}{ED}| and |\color{#ec0000}{BC}| are not parallel.
When we have several parallel lines intersected by 2 transversals, it is possible to use the generalized Thales' theorem.
Given that, in a figure:
-
lines |\color{#ec0000}{AA’},| |\color{#ec0000}{BB’}| and |\color{#ec0000}{CC’}| are parallel
-
points |A,| |B| and |C| are aligned
-
points |A’,| |B’| and |C’| are aligned
then the following proportion exists:
||\dfrac{\color{#3b87cd}{\text{m}\overline{A’B’}}}{\color{#3a9a38}{\text{m}\overline{AB}}} = \dfrac{\color{#3b87cd}{\text{m}\overline{B’C’}}}{\color{#3a9a38}{\text{m}\overline{BC}}} = \dfrac{\color{#3b87cd}{\text{m}\overline{A’C’}}}{\color{#3a9a38}{\text{m}\overline{AC}}}||
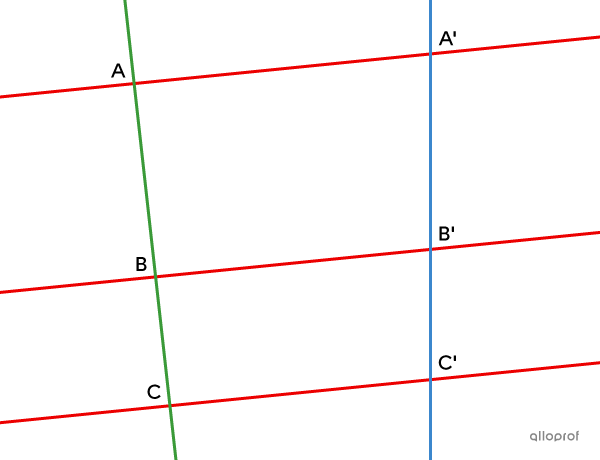
In the generalized Thales' theorem, we cannot establish a proportion between the segments located on the parallel lines |(AA’,| |BB’| and |CC’).|
||\dfrac{\text{m}\overline{AA’}}{\text{m}\overline{BB'}}\boldsymbol{\color{#ec0000}\neq} \dfrac{\text{m}\overline{BB’}}{\text{m}\overline{CC'}}\boldsymbol{\color{#ec0000}\neq} \dfrac{\text{m}\overline{AA’}}{\text{m}\overline{CC'}}||
Given that lines |\color{#ec0000}{AA’},| |\color{#ec0000}{BB’}| and |\color{#ec0000}{CC’}| are parallel, find |\text{m}\overline{B'C'}.|
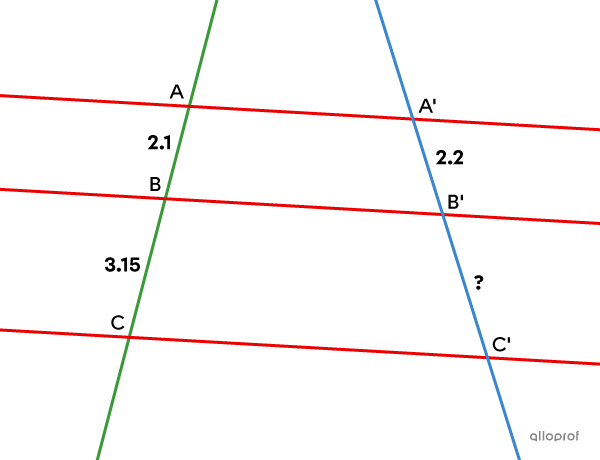
According to the generalized Thales’ theorem, since the lines |\color{#ec0000}{AA’},| |\color{#ec0000}{BB’}| and |\color{#ec0000}{CC’}| are parallel, the following proportion can be applied: ||\dfrac{\color{#3b87cd}{\text{m}\overline{A’B’}}}{\color{#3a9a38}{\text{m}\overline{AB}}} = \dfrac{\color{#3b87cd}{\text{m}\overline{B’C’}}}{\color{#3a9a38}{\text{m}\overline{BC}}} = \dfrac{\color{#3b87cd}{\text{m}\overline{A’C’}}}{\color{#3a9a38}{\text{m}\overline{AC}}}||
To find |\text{m}\overline{B’C’},| we solve the following equation:
||\begin{align}\dfrac{\color{#3b87cd}{\text{m}\overline{A'B'}}}{\color{#3a9a38}{\text{m}\overline{AB}}}&= \dfrac{\color{#3b87cd}{\text{m}\overline{B'C'}}}{\color{#3a9a38}{\text{m}\overline{BC}}}\\ \dfrac{\color{#3b87cd}{2.2}}{\color{#3a9a38}{2.1}}&= \dfrac{\color{#3b87cd}{x}}{\color{#3a9a38}{3.15}}\\\\ x&=\dfrac{3.15\times 2.2}{2.1}\\&=3.3\end{align}||
Answer: |\text{m}\overline{B’C’}=3.3\ \text{units}|