The volume of a sphere corresponds to the space occupied inside it. To find its volume, simply apply the following formula.
||V=\dfrac{4\pi r^3}{3}||where||\begin{align}V&:\text{Volume}\\ r&:\text{Radius of the sphere}\end{align}||
As with the area of a sphere, only the radius measurement is needed to calculate the volume of a sphere.
For the volume of a hemisphere, simply calculate the volume of the sphere and divide the result by 2.
To keep the water in a swimming pool clean, a company manufactures chlorine granules in the shape of a sphere.
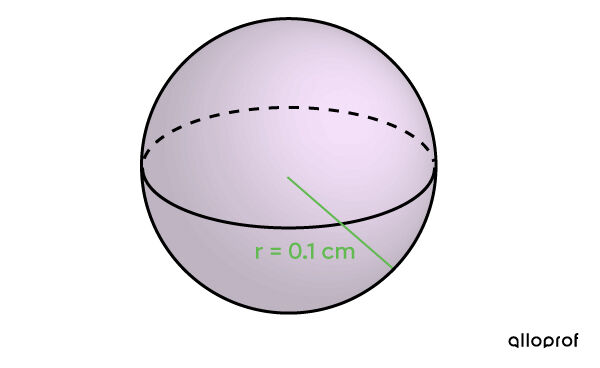
Assuming that the granules are well-compacted so the loss of space is negligible, how many granules will fit into a |5\ 000\ \text{cm}^3| container?
-
Identify the solid
In this context, it is clearly mentioned that the solid is a sphere. -
Apply the formula
||\begin{align}V&=\dfrac{4\pi r^3}{3}\\\\&=\dfrac{4\pi(0.1)^3}{3}\\\\&\approx0.004\ \text{cm}^3\end{align}|| -
Interpret the answer
To determine the number of granules, all that remains is the following division.||5\ 000\ \text{cm}^3\div0.004\ \text{cm}^3/\text{granule}=1\ 250\ 000\ \text{granules}||There are |1\ 250\ 000\ \text{granules}| in a |5\ 000\ \text{cm}^3| container.
Finally, remember the relationship between the radius, height and width of a sphere.
In some problems, we must find the measure of the radius when the volume is given. This is called finding a missing measure of a sphere from the volume.