The volume of a cylinder relates to the space it occupies in its environment. Even though the solid is not part of the prisms family, when calculating its volume, the same formula is used. The only difference is the area of a base will always be that of a circle.
|V = A_b \times h| or |V=\pi r^2\times h|
where
||\begin{align} A_b &= \text{Area of a base}\\ h &= \text{height of the cylinder}\\ r &= \text{radius of the base}\end{align}||
Health organizations set up vaccination programmes to protect people from various diseases. They use the following equipment to give people vaccines.
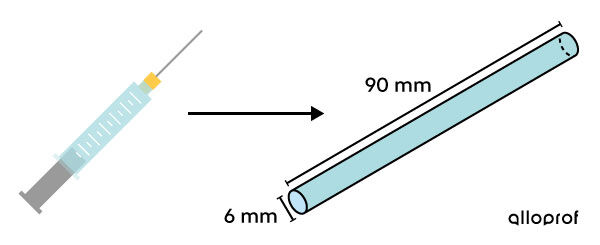
According to the example, what is the maximum volume of medicine, in |\text{mm}^3,| that this syringe can hold?
-
Identify the solid
From the illustration, we can determine it is a cylinder. -
Apply the formula
||\begin{align}V &= A_b \times h\\ \\ &= \pi r^2 \times h \\\\ &= \pi \left(\dfrac{6}{2} \right)^2 \times 90 \\\\ &= \pi(3)^2\times 90 \\\\ &\approx 2\ 544{.}69 \ \text{mm}^3\end{align}|| -
Interpret the answer
From the given information, the syringe can contain a maximum amount of approximately |2\ 544{.}69 \ \text{mm}^3| of medication.
Note : the radius must be used – not the diameter.
Sometimes, the base measurement or the height of the cylinder must be found, but only the volume is given. This situation is called finding a missing measurement of a cylinder from the volume. In this case, the approach is a little different, but it is still necessary to remember the formula used for the volume of a cylinder.