The area of a plane figure can be found by counting square units.
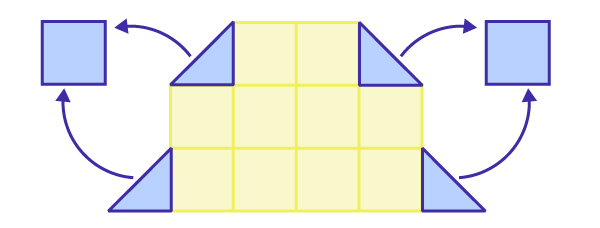
Some figures may contain half squares. Two half squares form a whole square unit.

Follow these steps to calculate a figure’s area.
-
If there are any half squares, join them to form whole-square units.
-
Count the total number of square units, including those formed using the half squares.
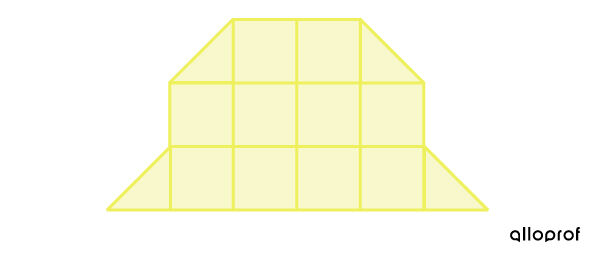
What is the area of this figure?
|
 |
|
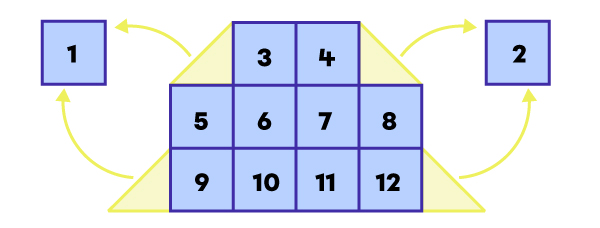 |
This figure’s area is 12 square units.
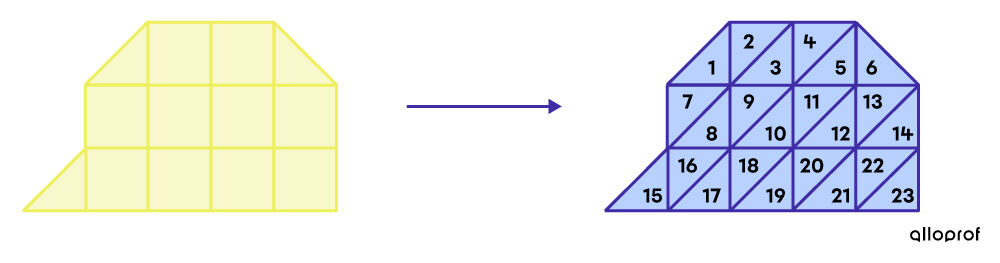
If a figure has an odd number of half squares, calculate the total area of the figure in terms of half-square units. To do this, each square unit must be divided into two half squares.
Example:
This figure has an area of 23 half squares.
To find the area of a square or rectangle, multiply the measure of its length by the measure of its width.
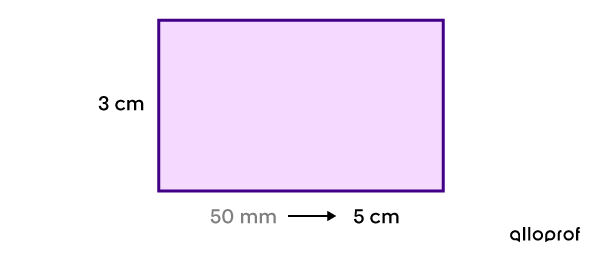
When calculating area, make sure that the same unit of measurement is used. If the units of measurement are different, first convert one to the other.
To learn how, consult the concept sheet Converting Units for Measuring Length.
Example:
I choose to convert 50 mm into cm to have a single unit of measurement.
Follow these steps to calculate the area of a figure.
-
I verify that the measurements are given using the same unit. If not, I convert them.
-
I multiply the length by the width to obtain the area.
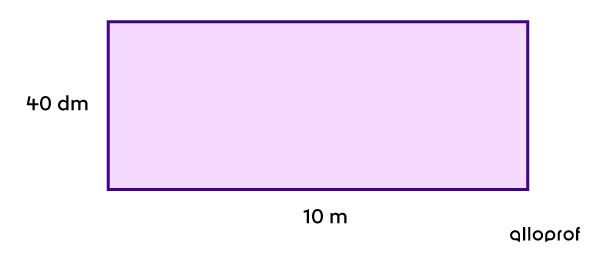
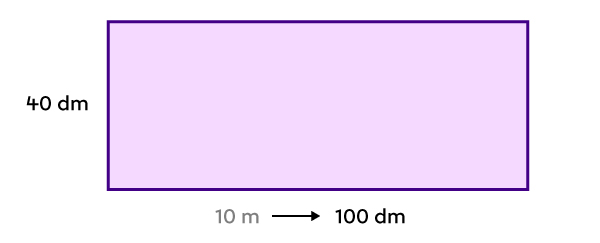
What is the area of the figure in square decimetres (dm²)?
|
 |
|
100 dm × 40 dm = 4 000 dm² |
The figure has an area of 4 000 dm².
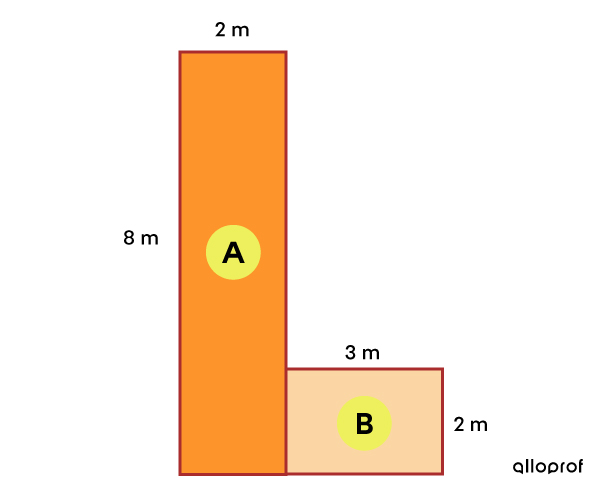
To find the area of a complex figure, first decompose the figure into squares or rectangles. Then, find the area of each of these sections.
Follow these steps to calculate the area of a complex figure:
-
I verify if the measurements are given using the same unit. If not, I convert them.
-
I decompose the figure into squares or rectangles.
-
I calculate the area of each section by multiplying the length by the width.
-
I add the areas of each section to obtain the total area.
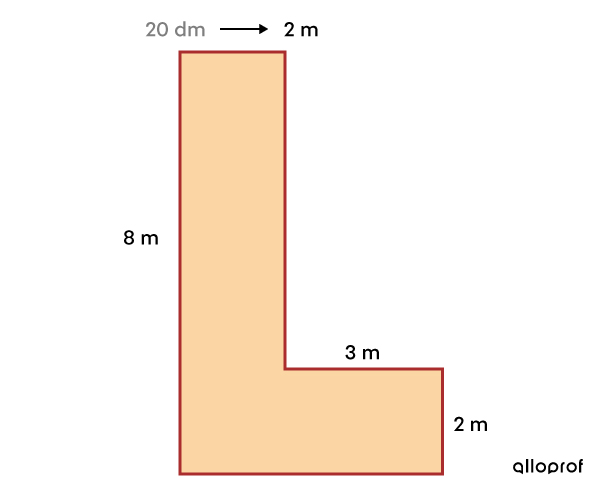
What is the area of the figure in square meters (m²)?
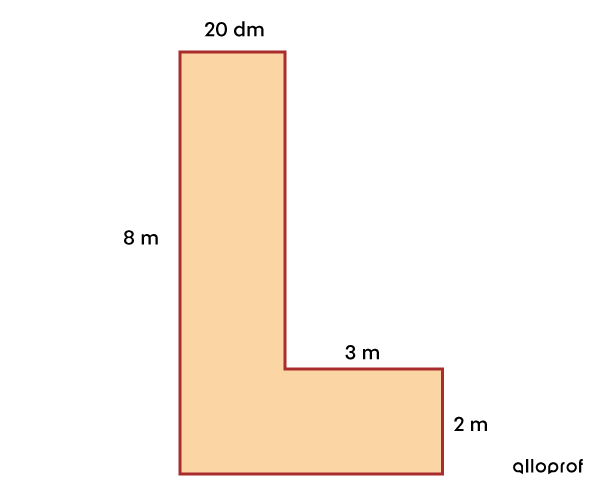
|
 |
|
 |
|
A: 8 m × 2 m = 16 m² B: 3 m × 2 m = 6 m² |
|
16 m² + 6 m² = 22 m² |
The area of the figure is 22 m².
Pour valider ta compréhension à propos de l'aire de façon interactive, consulte la MiniRécup suivante :
