The Pythagorean Theorem (also called the Pythagorean relationship) applies only to right triangles. The theorem is used to find the measure of one side when the measures of the other two sides are known.
The legs of a right triangle, often denoted |a| and |b,| correspond to the 2 sides that form the right angle.
The hypotenuse of a right triangle, denoted |c,| is the longest side. In other words, the hypotenuse is the side that is opposite the right angle.

The Pythagorean Theorem is stated as follows:
In a right triangle, the sum of the measures of the legs squared is equal to the measure of the hypotenuse squared.||a^2+b^2=c^2||
The Pythagorean Theorem is one of the oldest and most famous theorems in mathematics. Today, the mathematical community has compiled over 350 different proofs of this theorem.
In the following interactive animation, move the cursor to observe the reasoning behind one of these proofs.
Consider any right triangle with leg measurements |\boldsymbol{\color{#fa7921}{a}}| and |\boldsymbol{\color{#3b87cd}{b}}| and hypotenuse measurement |\boldsymbol{\color{#7cca51}{c}}.|
Four copies of the triangle are made, and placed in such a way as to form a square whose sides measure |\boldsymbol{\color{#fa7921}{a}+\color{#3b87cd}{b}}.| Inside this square, there is another square whose sides measure |\boldsymbol{\color{#7cca51}{c}}.| The area of this square is therefore |\boldsymbol{\color{#7cca51}{c^2}}.|
Then, by translating each triangle, the square whose area is represented by |\boldsymbol{\color{#7cca51}{c^2}}| is transformed into 2 other squares whose areas can be represented as |\boldsymbol{\color{#fa7921}{a^2}}| and |\boldsymbol{\color{#3b87cd}{b^2}}.| Therefore, the sum of the area of the square with side measurement |\boldsymbol{\color{#fa7921}{a}}| and the area of the square with side measurement |\boldsymbol{\color{#3b87cd}{b}}| is equal to the area of the square with side measurement |\boldsymbol{\color{#7cca51}{c}}.|
Below is the algebraic proof:||\begin{alignat}{13}4\times A_\Delta\,&+A_\boldsymbol{\color{#7cca51}{c}}&&=\ A_\boldsymbol{\color{#3b87cd}{a}+\color{#fa7921}{b}}\\4\times\dfrac{\boldsymbol{\color{#3b87cd}{a}\color{#fa7921}{b}}}{2}&+\boldsymbol{\color{#7cca51}{c}}^2&&=(\boldsymbol{\color{#3b87cd}{a}}+\boldsymbol{\color{#fa7921}{b}})^2\\\color{#ec0000}{\cancel{\color{black}{2ab}}}&+\boldsymbol{\color{#7cca51}{c}}^2&&=\boldsymbol{\color{#3b87cd}{a}}^2+\color{#ec0000}{\cancel{\color{black}{2ab}}}+\boldsymbol{\color{#fa7921}{b}}^2\\[3pt]&\quad\boldsymbol{\color{#7cca51}{c}}^2&&=\boldsymbol{\color{#3b87cd}{a}}^2+\boldsymbol{\color{#fa7921}{b}}^2\end{alignat}||
The Pythagorean Theorem can be represented using variables other than |a,| |b| and |c.| For example, the equation |x^2+y^2=z^2| is equally valid as the equation |a^2+b^2=c^2.|
Here are some examples of how to apply the Pythagorean Theorem.
What is the measure of the hypotenuse of triangle |ABC|?

Triangle |ABC| is a right triangle because the angle at vertex |C| is a 90° angle. The Pythagorean Theorem can therefore be used to find the measure of hypotenuse |(c),| since the measures of legs |(a=3| and |b=4)| are known.||\begin{align}a^2+b^2&=c^2\\3^2+4^2&=c^2\\9+16&=c^2\\25&=c^2\\\color{#ec0000}{\sqrt{\color{black}{25}}}&=\color{#ec0000}{\sqrt{\color{black}{c^2}}}\\5&=c\end{align}||Answer: The hypotenuse measures |5| units.
In the previous example, the right triangle has the distinction of having 3 natural numbers as side measures. We say that the numbers |3,| |4| and |5| form a Pythagorean triple, or a set of 3 natural numbers with which we can construct a right triangle. Here are some examples:||\begin{alignat}{13}\{3,4,5\}\ \ &\quad\Rightarrow\quad\,&&3^2&&+\ 4^2&&=\ 5^2\\\{5,12,13\}&\quad\Rightarrow\quad\,&&5^2&&+12^2&&=13^2\\\{8,15,17\}&\quad\Rightarrow\quad\,&&8^2&&+15^2&&=17^2\\\{7,24,25\}&\quad\Rightarrow\quad\,&&7^2&&+24^2&&=25^2\end{alignat}||Combined with the concept of similar figures, Pythagorean triples can be very useful for mentally calculating a missing measurement of a right triangle. For example, from the triplet |\{3,4,5\},| we can deduce the Pythagorean triplet |\{6,8,10\}| by multiplying each number by |2.|

What is the measure of leg |\overline{BC}| in triangle |ABC|?
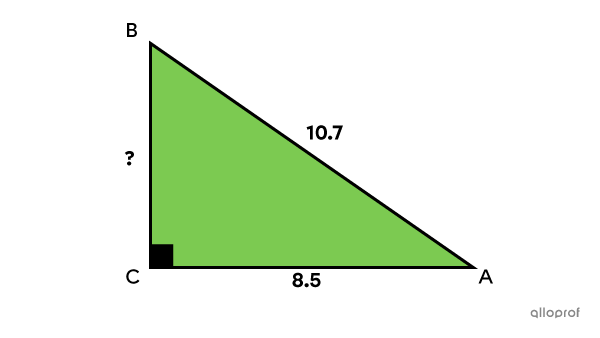
Triangle |ABC| is a right triangle because the angle at vertex |C| is a 90° angle. The Pythagorean Theorem can therefore be used to find the measure of the missing leg |(a),| since the measure of the other leg |(b=8.5)| and that of hypotenuse |(c=10.7)| are known.||\begin{align}a^2+b^2&=c^2\\a^2+8.5^2&=10.7^2\\a^2+72.25&=114.49\\a^2+72.25\boldsymbol{\color{#ec0000}{-72.25}}&=114.49\boldsymbol{\color{#ec0000}{-72.25}}\\a^2&=42.24\\\color{#ec0000}{\sqrt{\color{black}{a^2}}}&=\color{#ec0000}{\sqrt{\color{black}{42.24}}}\\a&\approx6.5\end{align}||Answer: The missing leg measures |6.5| units.
What is the measure of the legs in right triangle |ABC|?
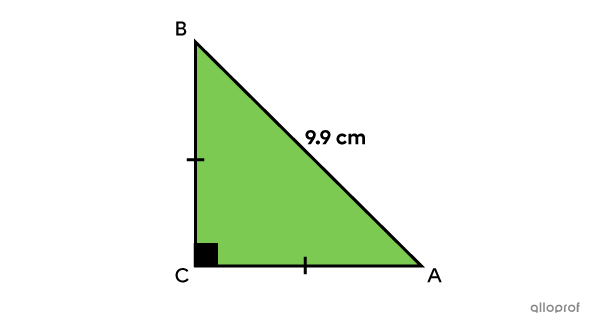
Triangle |ABC| is a right triangle, so the Pythagorean Theorem can be used to find the measure of the legs.||\begin{align}\left(\text{m}\overline{AC}\right)^2+\left(\text{m}\overline{BC}\right)^2&=\left(\text{m}\overline{AB}\right)^2\\[3pt]\left(\text{m}\overline{AC}\right)^2+\left(\text{m}\overline{BC}\right)^2&=9.9^2\\[3pt]\left(\text{m}\overline{AC}\right)^2+\left(\text{m}\overline{BC}\right)^2&=98.01\end{align}||In addition, triangle |ABC| is isosceles, since sides |\overline{AC}| and |\overline{BC}| are congruent. In other words, we can substitute |\text{m}\overline{AC}| for |\text{m}\overline{BC}| in the above equation.||\begin{align}\left(\textbf{m}\boldsymbol{\overline{AC}}\right)^2+\left(\text{m}\overline{BC}\right)^2&=98.01\\[3pt]\left(\textbf{m}\boldsymbol{\overline{BC}}\right)^2+\left(\text{m}\overline{BC}\right)^2&=98.01\\[3pt]2\left(\text{m}\overline{BC}\right)^2&=98.01\end{align}||Lastly, isolate |\text{m}\overline{BC}.|||\begin{align}\color{#ec0000}{\dfrac{\color{black}{2\left(\text{m}\overline{BC}\right)^2}}{\boldsymbol{2}}}&=\color{#ec0000}{\dfrac{\color{black}{98.01}}{\boldsymbol{2}}}\\[3pt]\color{#ec0000}{\sqrt{\color{black}{\left(\text{m}\overline{BC}\right)^2}}}&=\color{#ec0000}{\sqrt{\color{black}{\dfrac{98.01}{2}}}}\\[3pt]\text{m}\overline{BC}&=\text{m}\overline{AC}\approx7\end{align}||Answer: The legs measure approximately |7\text{ cm}.|
The Pythagorean Theorem can also be used to verify if a triangle is right-angled or not. A triangle is right-angled if and only if it satisfies the Pythagorean Theorem.
-
If the relationship |a^2+b^2=c^2| is true, the triangle is right-angled.
-
If the relationship |a^2+b^2=c^2| is false, the triangle is not right-angled.
Is triangle |ABC| a right triangle?
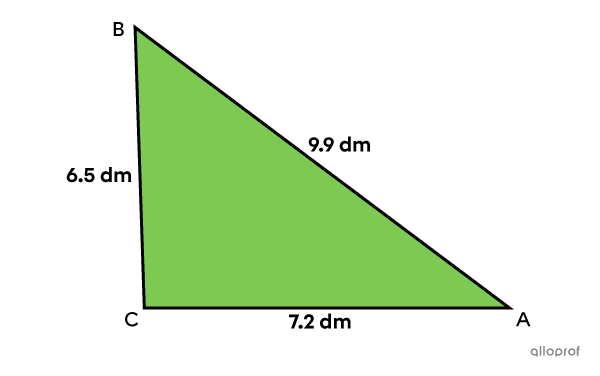
If triangle |ABC| is right-angled, its 3 sides must satisfy the Pythagorean Theorem. Let's verify if this is the case.||\begin{align}a^2+b^2&\stackrel{?}{=}c^2\\6.5^2+7.2^2&\stackrel{?}{=}9.9^2\\42.25+51.84&\stackrel{?}{=}98.01\\[3pt]94.09&\color{#ec0000}{\ne}98.01\end{align}||Triangle |ABC| does not respect the Pythagorean Theorem, since the answers are not equal.
Answer: Triangle |ABC| is not a right triangle since it does not respect the Pythagorean Theorem.