An irrational equation or inequality contains a variable under a root.
There are 2 restrictions that must always be taken into account when solving an equation or an inequality containing a square root.
-
A square root is always greater than or equal to |0.|
||\sqrt{a}\ge0|| -
The term under a square root, called the radicand, is always greater than or equal to |0.|
||a\ge0||
Referring to the restrictions makes it possible to validate the solutions obtained when solving the equation or inequality.
Here's how to solve an equation with one or more square roots.
-
Isolate the square root(s).
-
Check if the square root(s) is/are greater than or equal to |0| and calculate the restriction(s), if necessary.
-
Square both sides of the equation.
-
Solve the equation.
-
Verify the solution(s).
-
Give the solution set.
Solve the equation |4\sqrt{3x}=60.|
-
Isolate the square root
||\begin{align}4\sqrt{3x}&=60\\\sqrt{3x}&=15\end{align}|| -
Calculate the restrictions
Note that |\sqrt{3x}| is greater than |0,| because it is equal to |15.| It suggests that there is at least one solution. We find the restriction so the term under the root is positive.
||\begin{align}3x&\ge0\\x&\ge0\end{align}|| -
Square both sides of the equation
||\begin{align}\sqrt{3x}&=15\\\color{#EC0000}{(\color{black}{\sqrt{3x}})^2}&=15^{\color{#EC0000}{2}}\end{align}|| -
Solve the equation
||\begin{align}3x&=225\\\color{#3A9A38}{x}&=\color{#3A9A38}{75}\end{align}|| -
Verify the solution
The restriction |x\ge0| calculated in step 2 is respected because |\color{#3A9A38}{75}\ge0.| -
Give the solution
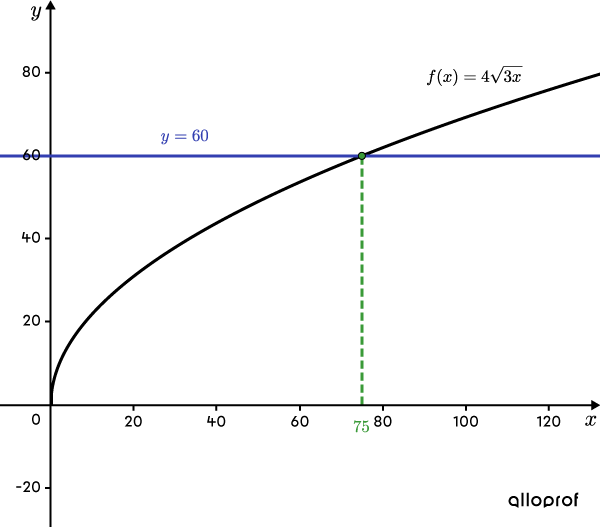
The following graph represents the function |f(x)=4\sqrt{3x}| and the line |\color{#333fb1}{y=60}.| Note that the line intersects the square root function at a point. The |x|-coordinate of the point corresponds to the value calculated in step 4.
The solution of the equation |4\sqrt{3x}=60| is |\color{#3A9A38}{x\color{black}{=}75}.|
There are 2 ways to verify the solution in step 5.
-
Ensure that the solution meets the restriction determined in step 2, as shown in the previous example.
-
Substitute the solution into the original equation. In the previous example, variable |x| is replaced in the equation |4\sqrt{3x}=60| and the left side of the equation is equivalent to the right side.
||\begin{align}4\sqrt{3\color{#EC0000}{x}}&=60\\4\sqrt{3(\color{#EC0000}{75})}&\overset{?}{=}60\\4\sqrt{225}&\overset{?}{=}60\\60&=60\end{align}||
The last equality is true, confirming that the solution |x=75| is valid.
Solve the equation |\sqrt{x-7}+2=9.|
-
Isolate the square root
||\begin{align}\sqrt{x-7}+2&=9\\\sqrt{x-7}&=7\end{align}|| -
Calculate the restrictions
Note that |\sqrt{x-7}| is greater than |0|, because it is equal to |7.| It suggests there is at least one solution. For the second restriction, we have:
||\begin{align}x-7&\ge0\\x&\ge7\end{align}|| -
Square both sides of the equation
||\begin{align}\sqrt{x-7}&=7\\\color{#EC0000}{(\color{black}{\sqrt{x-7}})^2}&=7^{\color{#EC0000}{2}}\end{align}|| -
Solve the resulting equation
||\begin{align}x-7&=49\\\color{#3A9A38}{x}&=\color{#3A9A38}{56}\end{align}|| -
Verify the solution obtained
The restriction |x\ge7| calculated in step 2 is respected, because |\color{#3A9A38}{56}\ge7.| -
Give the solution
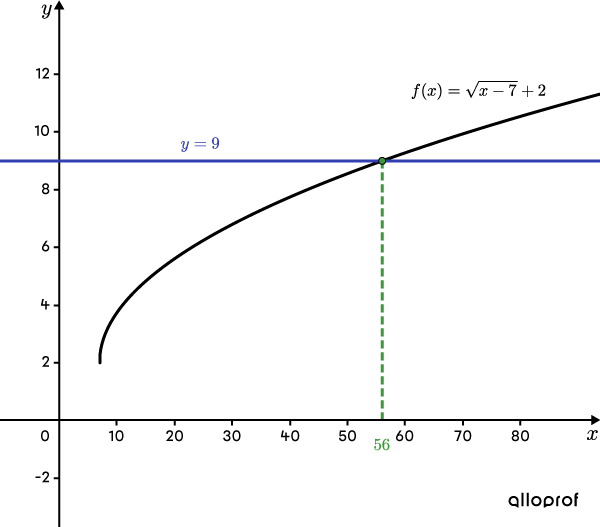
The following graph represents the function |f(x)=\sqrt{x-7}+2| and the line |\color{#333fb1}{y=9}.| Note that the line intersects the square root function at a point. The |x|-coordinate of the point corresponds to the value calculated in step 4.
The solution for the equation |\sqrt{x-7}+2=9| is |\color{#3A9A38}{x\color{black}{=}56}.|
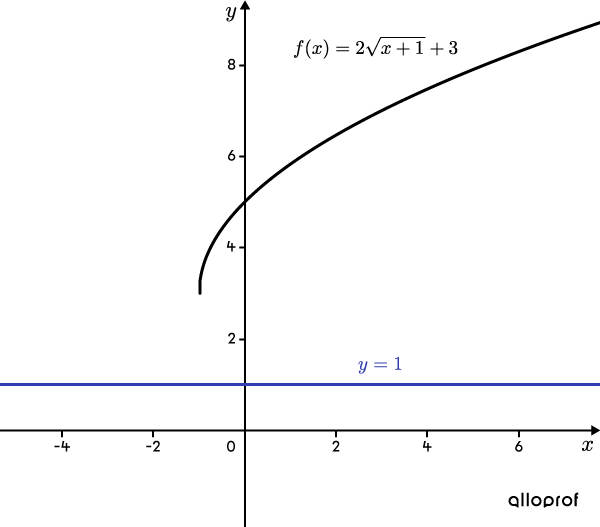
Here is an example of when the equation has no solution.
Solve the equation |2\sqrt{x+1}+3=1.|
-
Isolate the square root
||\begin{align}2\sqrt{x+1}+3&=1\\2\sqrt{x+1}&=-2\\\sqrt{x+1}&=-1\end{align}|| -
Calculate the restrictions
A square root must always be greater than or equal to |0,| which is not the case here, because we obtain the equation |\sqrt{x+1}=-1.|
The graph below represents the function |f(x)=2\sqrt{x+1}+3| and the line |\color{#333fb1}{y=1}.| Note that the line never meets the square root function. Since there is no point of intersection, there is no solution to the equation |2\sqrt{x+1}+3=1.|
The following is an example of when the solution brings up a second-degree equation.
Solve the equation |3\sqrt{x+3}-6=x-2.|
-
Isolate the square root
||\begin{align}3\sqrt{x+3}-6&=x-2\\3\sqrt{x+3}&=x+4\\\sqrt{x+3}&=\dfrac{x+4}{3}\end{align}|| -
Calculate the restrictions
Note that |\sqrt{x+3}| is greater than or equal to |0| only when |\dfrac{x+4}{3}| is as well. Therefore, it is necessary to determine the restriction.
||\begin{align}\dfrac{x+4}{3}&\ge0\\x+4&\ge0\\x&\ge-4\end{align}||
When |x\ge-4,| it suggests that there is at least one solution. We also need to find the restriction so that the term under the root is positive.
||\begin{align}x+3&\ge0\\x&\ge-3\end{align}||
We have |2| restrictions where |x\ge-4| and |x\ge-3.| Sometimes, like here, one of the |2| restrictions is unnecessary. So, it is sufficient to use only the |x\ge-3,| restriction, because a number |x| that is more than |-3| is greater than |-4.| Keep only |x\ge-3.| -
Square both sides of the equation
||\begin{align}\sqrt{x+3}&=\dfrac{x+4}{3}\\\color{#EC0000}{(\color{black}{\sqrt{x+3}})^2}&=\color{#EC0000}{\left(\color{black}{\dfrac{x+4}{3}}\right)^2}\end{align}|| -
Solve the resulting equation
This is a second-degree equation, so it is best to transform it to equal |0.|||\begin{align}x+3&=\dfrac{x^2+8x+16}{9}\\9(x+3)&=x^2+8x+16\\9x+27&=x^2+8x+16\\0&=x^2-x-11\end{align}||
Then use the quadratic formula to find the values of |x.|
||\begin{align}x&=\dfrac{-b\pm\sqrt{b^2-4ac}}{2a}\\&=\dfrac{-(-1)\pm\sqrt{(-1)^2-4(1)(-11)}}{2(1)}\\&=\dfrac{1\pm\sqrt{45}}{2}\\\color{#3A9A38}{x}&\in\{\color{#3A9A38}{-2.85},\color{#3A9A38}{3.85}\}\end{align}|| -
Check the solutions
The restriction |x\ge-3| calculated in step 2 is respected in both cases, because |\color{#3A9A38}{-2.85}\ge-3| and |\color{#3A9A38}{3.85}\ge-3.| -
Give the solution set
The following graph represents the function |f(x)=3\sqrt{x+3}-6| and the line |\color{#333fb1}{y=x-2}.| Note that the line intersects the square root function at 2 points. The |x|-coordinate for each point corresponds to one of the values calculated in step 4.
The solution set of the equation |3\sqrt{x+3}-6=x-2| is |x\in\{\color{#3A9A38}{-2.85},\color{#3A9A38}{3.85}\}.|
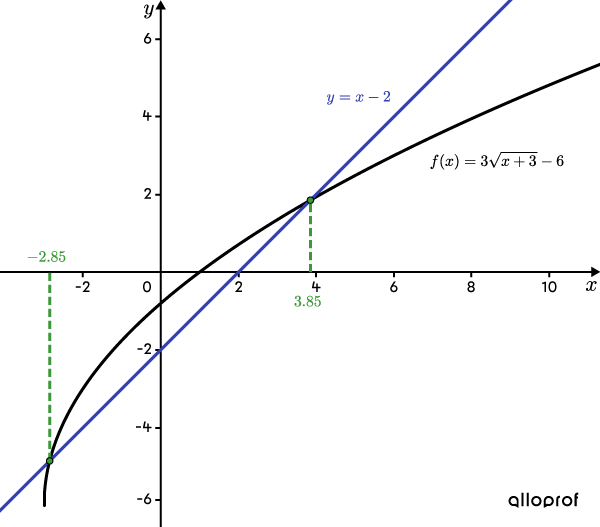
The following shows another example of when there is no solution to the equation.
Solve the equation |\sqrt{x-3}+\sqrt{x}=1.|
-
Isolate one of the square roots
||\begin{align}\sqrt{x-3}+\sqrt{x}&=1\\\sqrt{x-3}&=1-\sqrt{x}\end{align}|| -
Calculate the restrictions
Note that |\sqrt{x-3}| is greater than |0| only when |1-\sqrt{x}| is as well. So, it is necessary to determine the restriction.
||\begin{align}1-\sqrt{x}&\ge0\\1&\ge\sqrt{x}\\1^\color{#EC0000}{2} &\ge\color{#EC0000}{(\color{black}{\sqrt{x}})^2}\\1&\ge x\end{align}||
It suggests there is at least one solution when |x\le1.| Find the restriction so the term under the root is positive.
||\begin{align}x-3&\ge0\\x&\ge3\end{align}||
We have |2| conflicting restrictions where |x\le1| and |x\ge3.| Since there is no number less than |1| and more than |3,| there is no solution to the equation |\sqrt{x-3}+\sqrt{x}=1.|
The method for solving an inequality containing a square root is similar to the one for solving a square root equation. The most important difference is that it is always better to draw a graph of the function or number line to determine the solution. Including the restrictions and the visuals makes it easier to correctly identify the inequality’s solution set.
-
Replace the inequality symbol with the equal symbol.
-
Isolate the square root.
-
Check if the square root is greater than or equal to |0| and calculate the restriction, if necessary.
-
Square both sides of the equation.
-
Solve the equation.
-
Verify the solution set of the equation.
-
Determine the solution set of the inequality using a graph or a number line.
Solve the inequality |\sqrt{x-3}>2.|
-
Replace the inequality symbol with the equal symbol
||\sqrt{x-3}=2|| -
Isolate the square root
The square root is already isolated. -
Calculate the restrictions
Note that |\sqrt{x-3}| is greater than |0|, because it is equal to |2.| It suggests there is at least one solution. As for the restriction under the root, we have:
||\begin{align}x-3&\ge0\\x&\ge3\end{align}|| -
Square both sides of the equation
||\begin{align}\sqrt{x-3}&=2\\\color{#EC0000}{(\color{black}{\sqrt{x-3}})^2}&=\color{#EC0000}{\left(\color{black}{2}\right)^2}\end{align}|| -
Solve the equation
||\begin{align}x-3&=4\\x&=7\end{align}|| -
Validate the solution of the equation
The value |x=7| is valid because it respects the restriction |x\ge3| calculated in step 3. -
Determine the solution set of the inequality
To determine the solution set, we can draw the graph of the square root function |f(x)=\sqrt{x-3}.| Here's how.
The vertex of the function |f(x)=\sqrt{x-3}| is |(3,0),| because |h=3| and |k=0.| Moreover, it was determined in step 5 that the function passes through the point |\color{#333fb1}{(7,2)}.| With the 2 points, the curve of the square root function can be drawn.
Next, on the same Cartesian plane, draw the line with equation |\color{#333fb1}{y=2}| and indicate the region to be analysed. In this case, the straight line is dotted to clearly represent that we are only interested in the points of the curve where |f(x)| is strictly greater than 2.
Then, analyse the graph to determine whether the solution set is between |7| and |+\infty.|
The solution set of the inequality |\sqrt{x-3}>2| is |x\in\ ]7,+\infty[.|
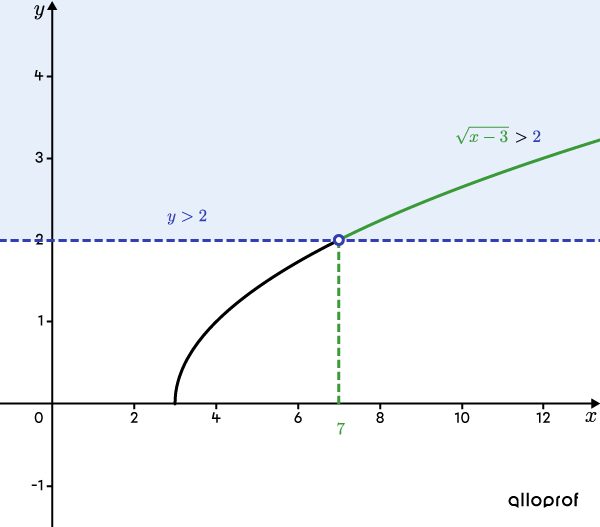
Graphing the function to determine the solution set in step 7 is not the only option. Sometimes, a number line is enough to get the same conclusion. Here's how.
First, we draw a number line and represent, with a solid point, the restriction |x\ge3| determined in step 3. Next, place an empty point at |x=7,| the solution obtained in step 5. The point is empty, because the radical |\sqrt{x-3}| is strictly greater than |2.|
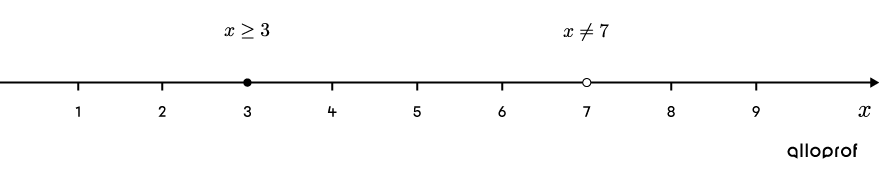
The 2 values of |x| divide the number line into 3 intervals: |]-\infty, 3],| |[3,7[,| and |]7,+\infty[.| It is necessary to validate a random value in each section of the initial inequality. However, it is not necessary to check the interval |]-\infty,3[,| because, as demonstrated in step 3, the solution to the inequality must be greater than or equal to |3.|
-
For |[3,7[,| use |x=5.|
||\begin{align}\sqrt{\color{#3A9A38}{x}-3}&>2\\\sqrt{\color{#3A9A38}{5}-3}&\stackrel{?}{>}2\\\sqrt{2}&\not>2\end{align}||
This is impossible and suggests the interval |[3,7[| is not part of the solution set.
-
For |]7,+\infty[,| use |x=9.|
The answer obtained is |\sqrt{6}>2,| which is true. Therefore, interval |]7,+\infty[| is part of the solution set.
On the number line, we can finally colour the section that corresponds to the solution set.
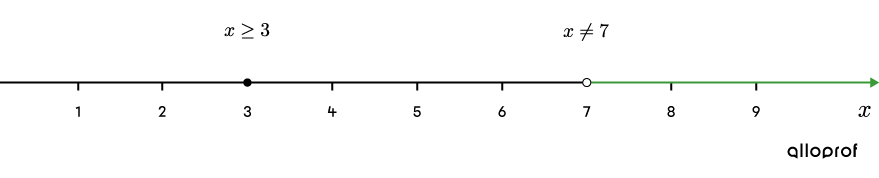
Therefore, as with the graph method, we determine that the solution set of the inequality |\sqrt{x-3}>2| is |]7,+\infty[.|
The following example shows when the solution is found using a number line.
Solve the inequality |\sqrt{2x-5}\le5.|
-
Replace the inequality symbol with an equal symbol
||\sqrt{2x-5}=5|| -
Isolate the square root
The square root is already isolated. -
Calculate the restrictions
Note that |\sqrt{2x-5}| is greater than |0|, because it is equal to |5.| It suggests there is at least one solution. As for the restriction under the root, we have:
||\begin{align}2x-5&\ge0\\2x&\ge5\\x&\ge\dfrac{5}{2}\end{align}||
On a number line, the restriction is indicated with a solid point.
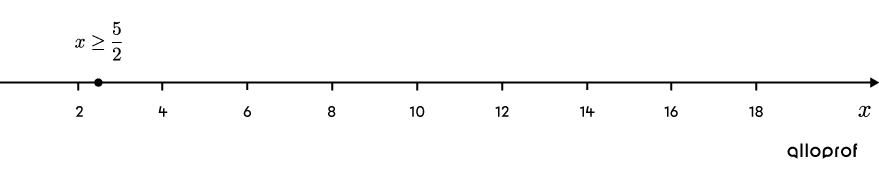
-
Square both sides of the equation
||\begin{align}\sqrt{2x-5}&=5\\\color{#EC0000}{(\color{black}{\sqrt{2x-5}})^2}&=\color{#EC0000}{\left(\color{black}{5}\right)^2}\end{align}|| -
Solve the equation
||\begin{align}2x-5&=25\\2x&=30\\x&=15\end{align}||
Add a solid point to |x=15| on the number line. The point is solid, because the symbol for the inequality is |\le.|
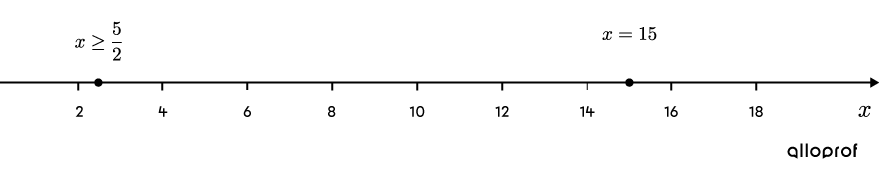
-
Verify the solution of the equation
The value |x=15| is valid, because it respects the restriction |x\ge\dfrac{5}{2}| calculated in step 3. -
Determine the solution set of the inequality
Now, check which interval is part of the solution set by replacing |x| in the original inequality. It is not necessary to check the interval |\left]-\infty,\dfrac{5}{2}\right[,| because it was proven in step 3 that the solution of the inequality is not in this interval.
Then, take a value of |x| between |\dfrac{5}{2}| and |15,| for example, |x=10.| We have:
||\begin{align}\sqrt{2\color{#EC0000}{x}-5}&\le5\\\sqrt{2(\color{#EC0000}{10})-5}&\stackrel{?}{\le}5\\\sqrt{15}&\stackrel{?}{\le}5\\3.87&\le5\end{align}||
The inequality is true, suggesting the interval |\left[\dfrac{5}{2},15\right]| is part of the solution set. Similarly, by replacing |x| by a number greater than |15,| such as |x=20,| it can be determined that the interval |]15,+\infty[| is not part of the solution set.

The solution set of the inequality |\sqrt{2x-5}\le5| is |\left[\dfrac{5}{2},15\right].|
Here is an example of when the square root function intersects a linear function. In this case, solve a second-degree equation and keep only one of the two solutions.
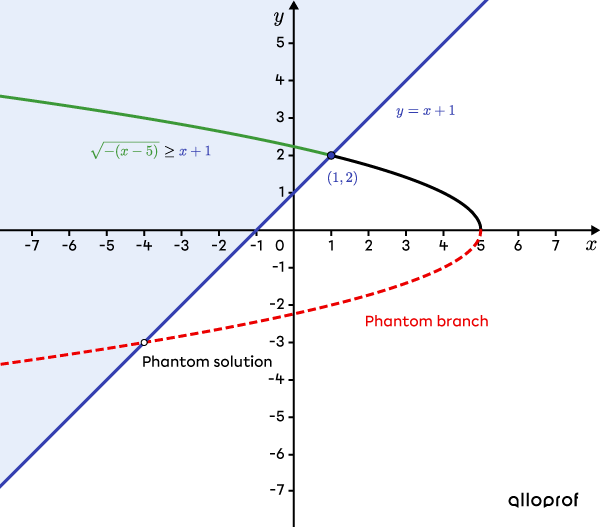
Solve the inequality |\sqrt{-(x-5)}\ge x+1.|
-
Replace the inequality symbol with the equal symbol
||\sqrt{-(x-5})=x+1|| -
Isolate the square root
The square root is already isolated. -
Calculate the restrictions
In the equation, |\sqrt{-(x-5)}| is greater than or equal to |0| when the following restriction is respected.
||\begin{align}x+1&\ge0\\x&\ge-1\end{align}||
For the restriction under the root, we have:
||\begin{align}-(x-5)&\ge0\\\color{#EC0000}{-1\times}-(x-5)&\color{#EC0000}{\le}0\color{#EC0000}{\times-1}\\x-5&\le0\\x&\le5\end{align}|| -
Square both sides of the equation
||\begin{align}\sqrt{-(x-5)}&=x+1\\\color{#EC0000}{\left(\color{black}{\sqrt{-(x-5)}}\right)^2}&=\color{#EC0000}{\left(\color{black}{x+1}\right)^2}\end{align}|| -
Solve the equation
First, transform the equation so it is equal to |0| and then use the quadratic formula.
||\begin{align}-(x-5)&=x^2+2x+1\\-x+5&=x^2+2x+1\\0&=x^2+3x-4\\\\x&=\dfrac{-b\pm\sqrt{b^2-4ac}}{2a}\\\\x&=\dfrac{-(3)\pm\sqrt{(3)^2-4(1)(-4)}}{2(1)}\\\\x&=\dfrac{-3\pm\sqrt{25}}{2}\\\\x&\in\{-4,1\}\end{align}|| -
Verify the solutions of the equation
The value |x=-4| is not valid, because it does not respect the restriction |x\ge-1| calculated in step 3. However, the value |x=1| is valid, because it respects both the restrictions |x\ge-1| and |x\le5.| -
Determine the solution set of the inequality
To determine the solution set, sketch the graph of the square root function |f(x)=\sqrt{-(x-5)}.| Here's how.
Function |f(x)=\sqrt{-(x-5)}| has a vertex at |(5,0)| and passes through the point |\color{#333fb1}{(1,2)}.| The 2 points can be used to draw the curve of the function.
Next, on the same Cartesian plane, draw the line with equation |\color{#333fb1}{y=x+1}| and indicate the region under analysis. In this case, the straight line should be solid to indicate that we are interested in the points on the curve where |f(x)| is greater than or equal to |\color{#333fb1}{x+1}|.
By studying the graph, we determine that the solution-set of the inequality |\sqrt{-(x-5)}\ge x+1| is |]-\infty,1].|
Note: The phantom branch is a consequence of the algebraic calculations in step 4. The second branch is generated by squaring the 2 sides of the equation. The branch crosses the line |\color{#333fb1}{y=x+1}| at |x=-4,| therefore, the reason why we must reject this solution at step 6.