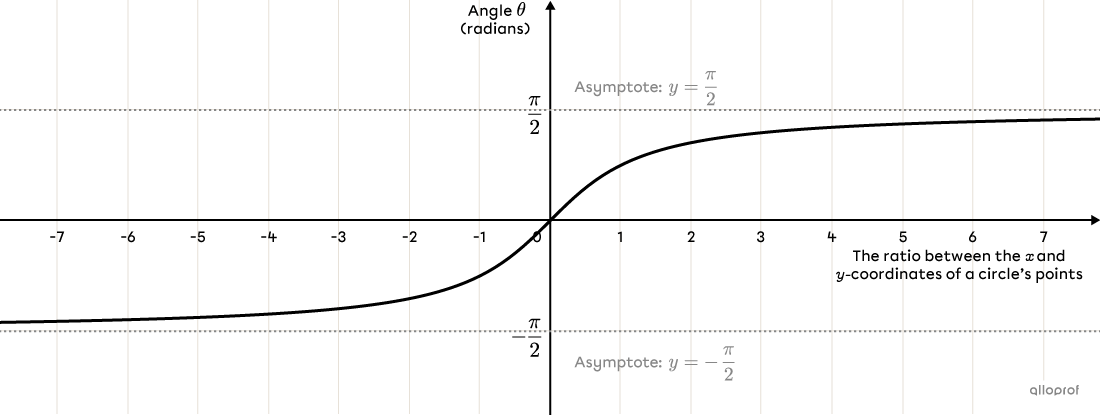
The inverse of the basic tangent function is the arc tangent function, which examines the measure of the angles of the unit circle (in radians) in relation to the ratio between the |y| and |x|-coordinates of the circle's points.
The rule for the basic arc tangent function is |f(x)=\arctan (x).| This function is also denoted |f(x)=\tan^{-1}(x).|
Note: Do not confuse the notation |\tan^{-1}(x)| with |\dfrac{1}{\tan (x)}.|
The following is a summary of the properties of the |\arctan(x)| function.
-
The function intersects the origin of the Cartesian plane.
-
The function has 2 horizontal asymptotes: |y=\dfrac{\pi}{2}| and |y=-\dfrac{\pi}{2}.|
-
The domain of the function is |\mathbb{R}.|
-
The range (codomain) is |\left]-\dfrac{\pi}{2}, \dfrac{\pi}{2}\right[.|
-
The function increases over its domain.
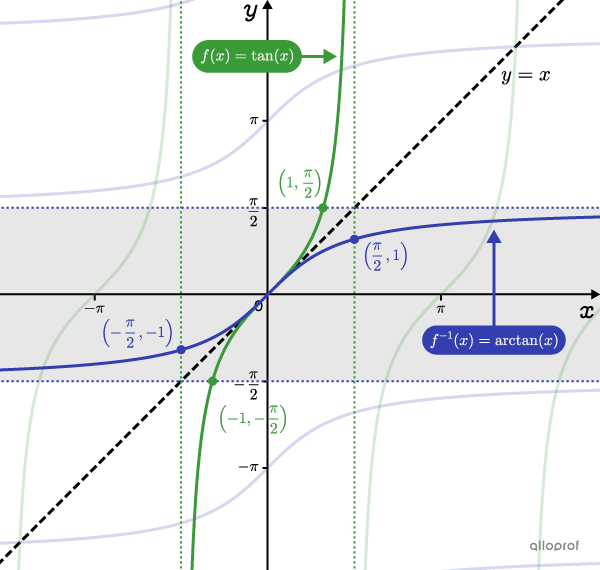
The inverse of a tangent function is not a function. For it to become a function, we must restrict its range.
On the Cartesian plane below, we plotted the basic tangent function. To plot its inverse, we interchange the |x| and |y| coordinates of the points of the function. We can also perform a reflection of the points with respect to the line of equation |y=x.| For example, the point |\left(1,\dfrac{\pi}{2}\right)| becomes the point |\left(\dfrac{\pi}{2},1\right).|
By doing so, we obtain another curve which is not a function. By restricting the range of the inverse to the interval |\left]-\dfrac{\pi}{2}, \dfrac{\pi}{2}\right[,| we create the arc tangent function.
To find the rule of the inverse of a tangent function, take the following steps.
-
Interchange |x| and |y| in the rule.
-
Isolate the |y|-variable.
-
Write the rule of the inverse.
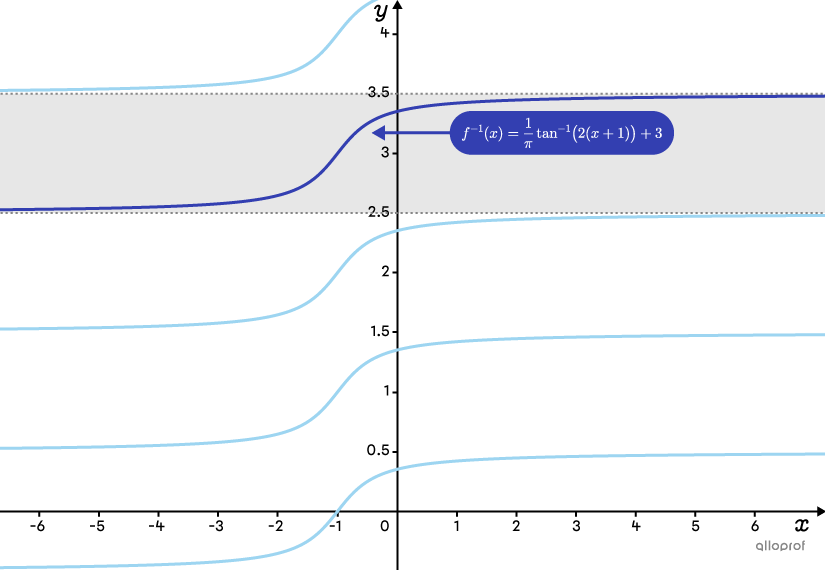
Find the rule of the inverse of the function |f(x)=\dfrac{1}{2}\tan\!\big(\pi(x-3)\big)-1.|
-
Interchange |\boldsymbol{x}| and |\boldsymbol{y}| in the rule||\begin{align}\color{#3B87CD}y&=\dfrac{1}{2}\tan\!\big(\pi(\color{#FF55C3}x-3)\big)-1\\ \color{#FF55C3}x&=\dfrac{1}{2}\tan\!\big(\pi(\color{#3B87CD}y-3)\big)-1\end{align}||
-
Isolate the |\boldsymbol{y}|-variable||\begin{align}x&=\dfrac{1}{2}\tan\!\big(\pi(\color{#3B87CD}y-3)\big)-1\\
x+1&=\dfrac{1}{2}\tan\!\big(\pi(\color{#3B87CD}y-3)\big)\\
\dfrac{x+1}{\frac{1}{2}}&=\tan\!\big(\pi(\color{#3B87CD}y-3)\big)\end{align}||To isolate |y,| it is necessary to eliminate |\tan| by performing the inverse operation, |\tan^{-1}.|||\begin{align}\color{#EC0000}{\tan^{-1}\!\big(\color{black}{2(x+1)}\big)}&=\pi(\color{#3B87CD}y-3)\\\dfrac{1}{\pi}\tan^{-1}\!\big(2(x+1)\big)&=\color{#3B87CD}y-3\\\dfrac{1}{\pi}\tan^{-1}\!\big(2(x+1)\big)+3&=\color{#3B87CD}y\end{align}|| -
Write the rule of the inverse
The rule of the inverse of the tangent function is the following.||f^{-1}(x)=\dfrac{1}{\pi}\tan^{-1}\!\big(2(x+1)\big)+3||
Note: For the inverse to become a function, we must restrict its range.
The following is the graphical representation of the arc tangent function from the previous example. For the inverse to become a function, we must restrict its range. In this example, we must restrict the range to the interval |]2.5,3.5[.|