Rational numbers include the set of integers, and therefore the set of natural numbers. However, unlike the numbers in these 2 sets, rational numbers can have a non-zero decimal part. The decimal expansion of a rational number can be either finite or infinite and periodic.
Rational numbers expressed in decimal notation have 2 possible forms:
-
A finite decimal expansion
-
An infinite and periodic (repeating) decimal expansion
Finite decimal development
The number |\dfrac{5}{4}| is a rational number. Dividing the numerator by the denominator equals the decimal number |1.25.|
This rational number has a finite decimal expansion, because its decimal expansion is made up of a finite number of digits (2 digits).
Infinite and periodic decimal development
The number |\dfrac{1}{3}| is a rational number. Dividing the numerator by the denominator equals |0.333333333\dots|
This rational number has an infinite decimal expansion. However, note that the number |3| repeats itself. This is the period. This rational number can be expressed as follows:||\dfrac{1}{3}=0.\overline{3}||
All rational numbers with finite decimal expansion are grouped together in a subset called the set of decimal numbers, represented by the symbol |\mathbb{D}.|
Rational numbers with an infinite and periodic decimal expansion are sometimes referred to as periodic numbers.
On a number line, rational numbers can be represented by points at the position of any number that can be written as a quotient of 2 whole numbers (a fraction).
The points on the number line below represent some elements of the set of rational numbers.

The rational numbers |(\mathbb{Q})| form a subset of the real numbers and include both the natural number set and the set of integers. The following notation can be used to represent the relationship between these sets. ||\mathbb N\subset\mathbb Z\subset \mathbb Q\subset \mathbb R|| Here's how to read this: "The set of natural numbers is included in the set of integers, which is included in the set of rational numbers, which itself is included in the set of real numbers."
Here's a diagram showing the location of rational numbers in the set of real numbers.
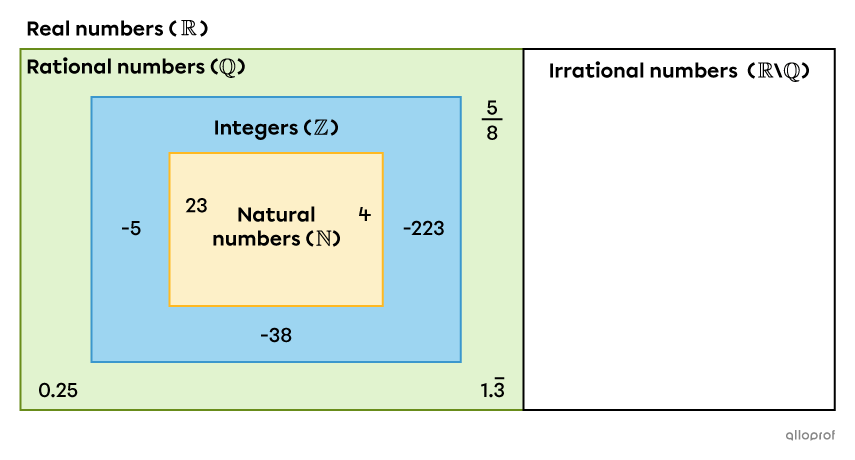
If a number cannot be written as a fraction of two integers, it is an irrational number.
-
The number |\dfrac{3}{4}| is a fraction of the form |\dfrac{a}{b}.| It is therefore a rational number.
-
The number |0.25| is a finite decimal number whose |\dfrac{a}{b}| form is |\dfrac{1}{4}.| It is therefore also a rational number.
-
The number |0.333\ldots=0.\overline{3}| is a number whose decimal expansion is infinite and periodic. It is therefore a rational number. Moreover, this number can be expressed as a fraction: |\dfrac{1}{3}.|
-
The integer |-7| can be written in the form |\dfrac{a}{b}| as |-\dfrac{7}{1}.| It is therefore also a rational number.
-
The number |2 \dfrac{1}{3}| is a mixed number whose |\dfrac{a}{b}| form is |\dfrac{7}{3}| and whose decimal expansion is |2.\overline{3}.| It therefore belongs to the set of rational numbers.
-
The numbers |\sqrt2| and |\pi| are examples of numbers that cannot be expressed in the form |\dfrac{a}{b}| where |a| and |b| are both integers, and whose decimal expansion is infinite and non-periodic. They are therefore not part of the set of rational numbers. They are irrational numbers.
-
Using appropriate notation, we get |0.25\in \mathbb{Q}| and |\sqrt2\notin \mathbb{Q}.|
-
We denote |\mathbb{Q}^*| the set of rational numbers excluding the number |0.|
-
We denote |\mathbb{Q}_+| the set of positive rational numbers.
-
We denote |\mathbb{Q}_-| the set of negative rational numbers.
-
It is possible to combine these notations. For example, we denote |\mathbb {Q}_-^*| the set of negative rational numbers excluding the number |0.|
To order rational numbers, it's helpful to transform them into the same form of representation (fractions, mixed numbers or decimal numbers).