Here is a brief study guide containing all the concepts covered in the Secondary 4 SN mathematics pathway. To explain everything, each formula will be followed by an example and a link to a concept sheet in our virtual library.
Dividing polynomials is done the same way as dividing two numbers using long division.
Find the result of the following division:
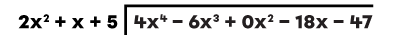
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
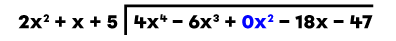 |
Ensure that there is a term for each of the |\color{blue}{degrees}| of the variable |x| in the dividend. |
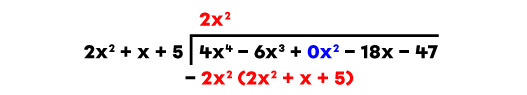 |
Determine the |\color{red}{monomial}| that you must multiply |2x^2 + x + 5| by to cancel the |x^4| term of the polynomial. |
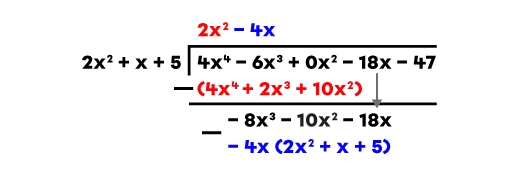 |
Determine the |\color{blue}{monomial}| that you must multiply |2x^2 + x + 5| by to cancel the |x^3| term in the polynomial. |
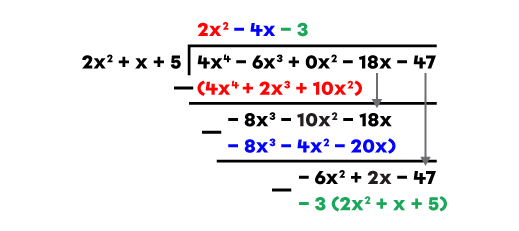 |
Determine the |\color{green}{monomial}| that you must multiply |2x^2 + x + 5| by to cancel the |x^2| term in the polynomial. |
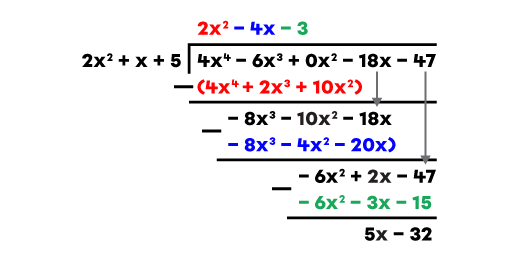 |
Identify the remainder when the degree of the subtraction’s result is smaller than the binomial in the divisor. |
|
The result of the division is |2x^2 - 4x - 3| remainder |5x - 32| or |2x^2 - 4x - 3 + \dfrac{5x - 32}{2x^2 + x + 5}.| |
|
You can follow the steps below to add or subtract rational expressions:
-
Factor the numerator and denominator of each fraction.
-
Establish all of the restrictions (denominators other than 0).
-
If possible, cancel the common factors in each of the fractions.
-
Find a common denominator.
-
Complete the addition or subtraction with the numerator.
-
If possible, simplify the final rational expression by factoring the numerator and denominator.
Simplify the following algebraic expression:
|\displaystyle \frac{x-2}{x+5} - \frac{3}{-3x-12}|
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align} &\dfrac{x-2}{x+5} - \dfrac{3}{-3x-12} \\\\ =\ &\dfrac{x-2}{x+5}\color{red}{-}\dfrac{\color{red}{3}}{\color{red}{-3}(x+4)} \end{align}| |
Factor the numerator and denominator of each fraction. |
|
|\begin{align} x+5\neq 0\ &\rightarrow\ x\neq -5 \\ -3(x+4)\neq 0\ &\rightarrow\ x\neq -4 \end{align}| |
Establish all of the restrictions (denominators other than 0). |
|
|\begin{align} =\ &\dfrac{x-2}{x+5}\color{red}{-}\dfrac{\color{red}{3}}{\color{red}{-3}(x+4)} \\ =\ &\dfrac{x-2}{x+5}+\dfrac{1}{x+4} \end{align}| |
Simplify the common factors. Here, |\color{red}{-3}| can be simplified. |
|
|=\ \dfrac{(x-2)\color{red}{(x+4)}}{(x+5)\color{red}{(x+4)}}+\dfrac{1 \color{red}{(x+5)}}{(x+4)\color{red}{(x+5)}}| |
Place all the fractions over a common denominator. |
|
|\begin{align} =\ &\dfrac{(x-2)(x+4)+1(x+5)}{(x+4)(x+5)} \\ =\ &\dfrac{x^2-2x+4x-8+x+5}{(x+4)(x+5)} \\ =\ &\dfrac{x^2+3x-3}{(x+4)(x+5)} \end{align}| |
Complete the operation on numerators and simplify like terms. |
|
|=\ \dfrac{x^2+3x-3}{(x+4)(x+5)}| |
In this case, it is not possible to factor the numerator using the product-sum method. Even using the completing the square method, factorization would not simplify the numerator and denominator. |
|
So, the result of the difference of these two rational expressions is |\dfrac{x^2+3x-3}{(x+4)(x+5)}| where |x\neq -4| and |x\neq -5.| |
|
It is important to master the concept of distributivity associated with multiplication:
-
If possible, simplify expressions in brackets.
-
Distribute each of the terms in the first bracket over all the terms in the second bracket.
-
Simplify by adding and subtracting like terms.
Find the simplified algebraic expression for the following multiplication: ||(7x+4)(2x^2-4x+3)||
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align} &(7x+4)(2x^2−4x+3)\\ |
Distribute each of the terms in the first bracket to the terms in the second bracket. |
|
|\begin{align} &14x^3 + −28x^2 + 21x + 8x^2 + −16x + 12 \\ =\ &14x^3 + −20x^2 + 5x + 12\end{align}| |
Perform addition and subtraction on the like terms. |
|
The simplified algebraic expression is: |14x^3 − 20x^2 + 5x + 12| |
|
See also
Sometimes several factorization methods are required to factor an algebraic expression. As a result, it is important to master all of them.
|
EXAMPLE OF THE PRODUCT-SUM METHOD |
|
|---|---|
|
What measurements (in numerical form or as an algebraic expression) can be associated with each of the dimensions of a rectangular prism whose volume is |4x^2+8x−32\ \text{cm}^3|? |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} &4x^2+8x−32 \\ =\ &4(x^2+2x−8)\end{align}| |
If possible, factor out the greatest common factor, making sure that all coefficients remain whole numbers. |
|
|\begin{align} &4(\color{blue}{x^2}+\color{red}{2x}\color{green}{−8}) \\\\ P =\ &\color{blue}{1}\times \color{green}{−8}=−8 \\ S =\ &\color{red}{2} \end{align}| The numbers are |4| and |−2,| because |4\times -2 = -8| and |4+-2=2.| |
Determine the numbers that correspond to the product and the sum of the polynomial in brackets. |
|
|\begin{align} &4(x^2+\color{red}{2x}−8) \\ |
Separate the |\color{red}{x}| term into two terms, using the two numbers found and factor using the grouping method. |
|
The three dimensions measurement are |4,| |(x+4)| and |(x−2)| cm. |
|
|
EXAMPLE OF A DIFFERENCE OF SQUARES |
|
|---|---|
|
What are the algebraic expressions that represent the measurement of the base and the height of a triangle whose area is |(2x^2 −8)\ \text{m}^2\ ?| |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} \frac{\color{blue}{b}\times \color{red}{h}}{2} &= 2x^2 - 8 \\ \Rightarrow\ \color{blue}{b}\times \color{red}{h} &=4x^2 -16 \end{align}| |
Create the equation that represents the situation. |
|
|\begin{align} \sqrt{4x^2} &= 2x \\ \sqrt{16} &= 4 \end{align}| This is a subtraction between two terms. |
Verify that the binomial meets the criteria of a difference of squares. |
|
|\begin{align} \color{blue}{b} \times \color{red}{h} &= 4x^2 − 16 \\ \Rightarrow\ \color{blue}{b} \times \color{red}{h} &= \color{blue}{(2x−4)}\color{red}{(2x+4)} \end{align}| |
Factor according to this model: |a^2-b^2=(a-b)(a+b)| |
|
Therefore, we can conclude that |\color{blue}{b = (2x−4)}| and |\color{red}{h = (2x+4)}\ \text{m}.| |
|
|
EXAMPLE OF A PERFECT SQUARE TRINOMIAL |
|
|---|---|
|
What is the algebraic expression associated with the measurement of one side of a square that has an area of |\color{blue}{9}x^2 − \color{red}{42}x + \color{green}{49}\ \text{m}^2|? |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} \sqrt{\color{blue}{a}} &= \sqrt{\color{blue}{9}} = \color{blue}{3} \\ \sqrt{\color{green}{c}} &= \sqrt{\color{green}{49}} = \color{green}{7} \\\\ \color{red}{c} &\overset{?}{=} 2\sqrt{\color{blue}{a}}\sqrt{\color{green}{c}} \\ \Rightarrow\ \color{red}{42} &= 2\times \color{blue}{3} \times \color{green}{7} \end{align}| |
Verify that the trinomial is indeed a perfect square trinomial. |
|
|\begin{align} &\color{blue}{9}x^2 − \color{red}{42}x + \color{green}{49} \\ =\ &(\color{blue}{3}x-\color{green}{7})^2 \end{align}| |
Factor according to the perfect square trinomial model. |
|
Since the area of a square is calculated using the formula |A=c^2,| we can deduce that |A=(\color{blue}{3}x-\color{green}{7})^2.| By association, we obtain |c=(\color{blue}{3}x-\color{green}{7})\ \text{m}.| |
|
|
EXAMPLE USING THE COMPLETING THE SQUARE METHOD |
|
|---|---|
|
Find the equation of this parabola in its factored form: ||f(x) = -3x^2 - \frac{1}{2}x + 6|| |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} &\color{blue}{-3}x^2 - \dfrac{1}{2}x + 6 \\ =\ &\color{blue}{-3}\left(x^2 + \color{red}{\dfrac{1}{6}}x - 2\right) \end{align}| |
Factor out a common factor to ensure that the coefficient of the term in |x^2 = 1.| |
|
|\begin{align} &\left(\dfrac{\color{red}{b}}{2}\right)^2 \\ =\ &\left(\dfrac{\color{red}{\frac{1}{6}}}{2}\right)^2 \\ =\ &\color{green}{\frac{1}{144}} \end{align}| |
Calculate the value of |\left(\dfrac{\color{red}{b}}{2}\right)^2|. |
|
|\begin{align} &-3\left(x^2 + \frac{1}{6}x \color{green}{+ \frac{1}{144} - \frac{1}{144}} - 2\right) \\ = &-3 \left(\left[x^2 + \frac{1}{6}x \color{green}{+ \frac{1}{144}}\right] \color{green}{- \frac{1}{144}} - 2\right) \\ = &-3 \left(\left[x + \frac{1}{12}\right]^2 - \frac{289}{144}\right) \end{align}| |
Add and then subtract this value to get a perfect square trinomial. |
|
|\begin{align} &-3 \left(\left[x + \frac{1}{12}\right]^2 - \frac{289}{144}\right) \\ = &-3 \left(\Big(x + \frac{1}{12} + \frac{17}{12}\Big)\Big(x + \frac{1}{12} - \frac{17}{12}\Big)\right) \\ = &-3 \left(x + \frac{18}{12}\right) \left(x - \frac{16}{12}\right) \\ = &-3 \left(x + \frac{3}{2}\right) \left(x - \frac{4}{3}\right) \end{align}| |
Factor using a difference of squares with the terms in brackets. |
|
The factored form of the starting equation is: |f(x) = -3 \left(x + \dfrac{3}{2}\right) \left(x - \dfrac{4}{3}\right).| |
|
|
EXAMPLE OF THE QUADRATIC FORMULA |
|
|---|---|
|
What are the algebraic expressions or measurements that can be associated with the measurements of the three dimensions of a rectangular prism whose volume is |(\color{blue}{3}x^2 + \color{green}{4}x \color{red}{- 8})\ \text{mm}^3|? |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} &\dfrac{^-\color{green}{b} \pm \sqrt{\color{green}{b}^2 - 4 \color{blue}{a} \color{red}{c}}}{2 \color{blue}{a}} \\ =\ &\dfrac{^-\color{green}{4} \pm \sqrt{\color{green}{4}^2 - 4 (\color{blue}{3})(\color{red}{^-8})}}{2 (\color{blue}{3})} \end{align}| |
Apply the quadratic formula: |\dfrac{^-b \pm \sqrt{b^2 - 4ac}}{2a}| |
|
|\begin{align} &\dfrac{^-\color{green}{4} \pm \sqrt{\color{green}{4}^2 - 4 (\color{blue}{3})(\color{red}{^-8})}}{2 (\color{blue}{3})} \\ =\ &\dfrac{^-\color{green}{4} \pm \sqrt{112}}{2 (\color{blue}{3})} \end{align}| |
Simplify the radicand. |
|
|\begin{align} &\dfrac{^-\color{green}{4} \pm \sqrt{112}}{2 (\color{blue}{3})} \\\\ \Rightarrow x_1=\ &\dfrac{^-\color{green}{4} + \sqrt{112}}{2 (\color{blue}{3})} &&\text{et}\ x_2 = \dfrac{^-\color{green}{4} - \sqrt{112}}{2 (\color{blue}{3})} \\ \Rightarrow x_1 \approx\ &1{.}1 &&\text{et}\ x_2\ \approx ^-2{.}43 \end{align}| |
Identify the two possible answers. |
|
|\color{blue}{3}x^2 + \color{green}{4}x \color{red}{- 8}= 3(x-1{.}1)(x-^-2{.}43)| |
Write the factorization of the initial polynomial. |
|
Arbitrarily, the measurements of width, depth, and height of the prism can be associated with |3\ \text{mm},| |(x-1{.}1)\ \text{mm}| and |(x+2{.}43)\ \text{mm}.| |
|
See also
Functional form (Standard form): |f(x) = a(x-h)^2 + k| where |(h,k)| is the coordinate of the vertex.
General form: |f(x) = ax^2 + bx + c|
Factored form: |f(x) = a (x - z_1) (x - z_2)| where |z_1| and |z_2| are the zeros of the parabola.
Use the information provided in the table below to determine the parabola’s equation in its three different forms.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align} \color{blue}{f(x)} &= a (\color{blue}{x} - \color{red}{h})^2 + \color{red}{k} \\ \color{blue}{f(x)} &= a \left(\color{blue}{x} - \color{red}{\dfrac{-17}{20}}\right)^2 + \color{red}{\dfrac{-169}{32}} \\ \color{blue}{\dfrac{-61}{50}} &= a \left(\color{blue}{2} - \color{red}{\dfrac{-17}{20}}\right)^2 + \color{red}{\dfrac{-169}{32}} \\ \dfrac{1}{2} &= a \\\\ \Rightarrow\ f(x) &= \dfrac{1}{2} \left(x + \dfrac{17}{20}\right)^2 - \dfrac{169}{32} \end{align}| |
To find the equation in function form (standard form), we replace the coordinates of the vertex |\color{red}{(h,k) = (\frac{-17}{20}, \frac{-169}{32})}| and find the value of parameter |a| by substituting in the coordinates of another point |\color{blue}{(x,y) = (2, \frac{-61}{50})}|. |
|
|\begin{align} f(x) &= \dfrac{1}{2} \left(x + \dfrac{17}{20}\right)^2 - \dfrac{169}{32} \\ \Rightarrow\ f(x) &= \dfrac{x^2}{2} + \dfrac{17x}{20} - \dfrac{123}{25} \end{align}| |
To find the equation in general form, simply expand the polynomial expression of the standard form. |
|
|\begin{align} f(x) &= \frac{1}{2} \left(x + \frac{17}{20}\right)^2 - \frac{169}{32} \\ \Rightarrow\ f(x) &= \frac{1}{2} \left(x - \frac{12}{5}\right) \left(x + \frac{41}{10}\right) \end{align}| |
To find the equation in factored form, find the zeros of the function using the quadratic formula or by factoring through completing the square. |
For second-degree polynomial functions: |a \ne \dfrac{\Delta y}{\Delta x}|
See also
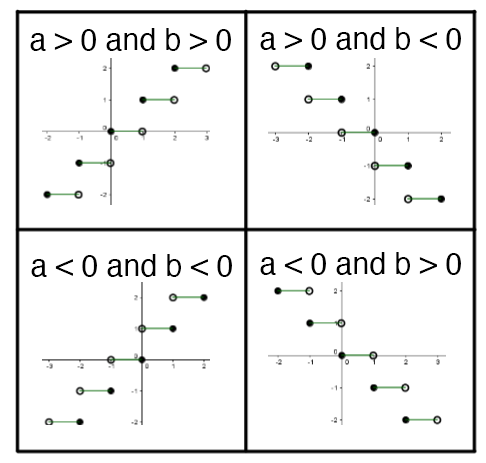
A step function’s equation is written in the form ||f(x) = a \left[ b(x-h)\right] + k||
where
|(h,k) = | coordinates of a closed point
|{\mid}a{\mid} = | vertical distance or height between two steps and
|\dfrac{1}{\mid b \mid} = | length of a step
To determine the signs of |a| and |b,| we can refer to the direction of the open and closed points and the increasing or decreasing variation of the graph:
A grocery store has a new reward program that offers stamps for discounts on certain items.
Customers receive five stamps with a minimum purchase of $5. They receive seven more stamps for every additional $22 they spend.
Using this information, what interval corresponds to a customer's total bill if they receive 47 stamps from their purchase?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
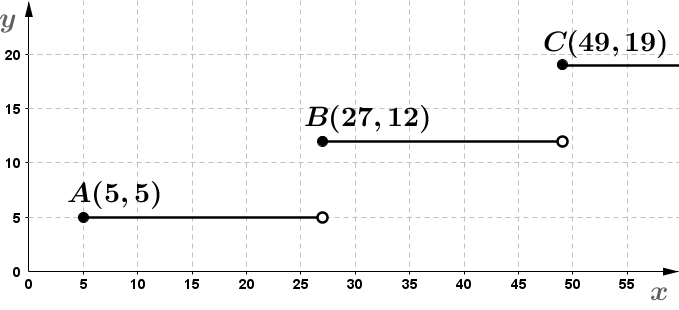 |
Sketch the graph associated with this situation. |
|
|\begin{align}{\mid}\color{red}{a}{\mid} &= 12 - 5 = 7 \\\\ \dfrac{1}{{\mid}\color{blue}{b}{\mid}} &= 27 - 5 = 22 \\ \Rightarrow\ {\mid}\color{blue}{b}{\mid} &=\dfrac{1}{22} \\\\ (h,k) &= (5,5) \end{align}| |
Find the value of |\mid \color{red}{a} \mid,| of |\mid \color{blue}{b} \mid| , and of |(h,k).| |
|
|\begin{align} f(x) &= \color{red}{a} \left[ \color{blue}{b}(x-h) \right] + k \\ \Rightarrow\ f(x) &= \color{red}{7} \left[ \color{blue}{\dfrac{1}{22}} ( x - 5) \right] + 5 \end{align}| |
Write the equation of this function taking into account the orientation of the open and closed points. |
|
|\begin{align} f(x) &= \color{red}{7} \left[ \color{blue}{\dfrac{1}{22}}(x - 5)\right] + 5 \\ |
Find the value of |x| when |f(x)| is worth |47.| |
|
|x \in \left[137, 159\right[| |
Determine the interval in |x| of the solution. |
|
Thus, the purchase amount must be at least |\$137| but less than |\$159|. |
|
See also
The inverse of function |f(x)|, denoted as |f^{-1}(x)|, is obtained by inverting the coordinates of the points such that |(x,y) \rightarrow (y,x)|
Sketch the inverse of the following function:
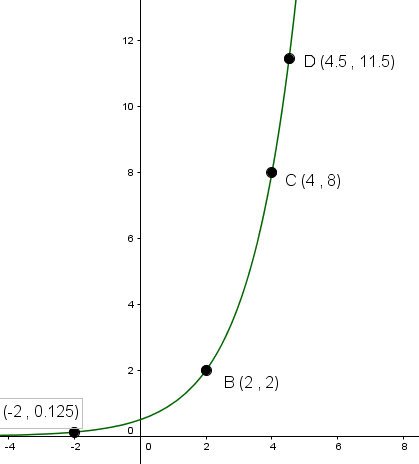
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
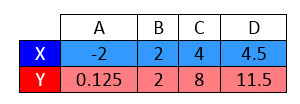 |
Create a table of values based on the given points. |
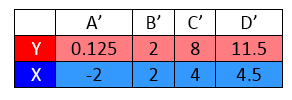 |
Invert the coordinates: |(\color{blue}{x}, \color{red}{y}) \rightarrow (\color{red}{y}, \color{blue}{x})| |
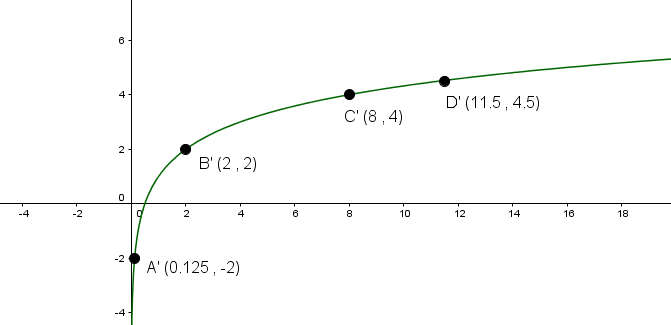 |
Sketch the graph of the inverse using this new table of values. |
The inverse of a function is not always a function itself. In the cases studied here, these functions are inverses of each other:
-
Exponential and logarithmic functions;
-
A linear function with respect to itself;
-
A second-degree function (with a restricted domain) and a square root function.
See also
The same criteria must always be analyzed when studying a function:
-
the domain: all possible values of |x|
-
the range: all possible values of |y|
-
the x-intercept (zeros): the value(s) of |x| when |y=0|
-
the y-intercept: the value of |y| when |x=0|
-
the maximum: the greatest value of |y|
-
the minimum: the smallest value of |y|
-
increasing: when the graph "goes upwards" or is constant
-
decreasing: when the graph "goes downwards" or is constant
-
positive sign: portion of the graph that is above or equal to the |x|-axi
-
negative sign: portion of the graph that is below or equal to the |x|-axis
As an accountant of a large company, you must give a detailed account of the trends in profits over the past year. To help, here is a graph of the last 12 months.
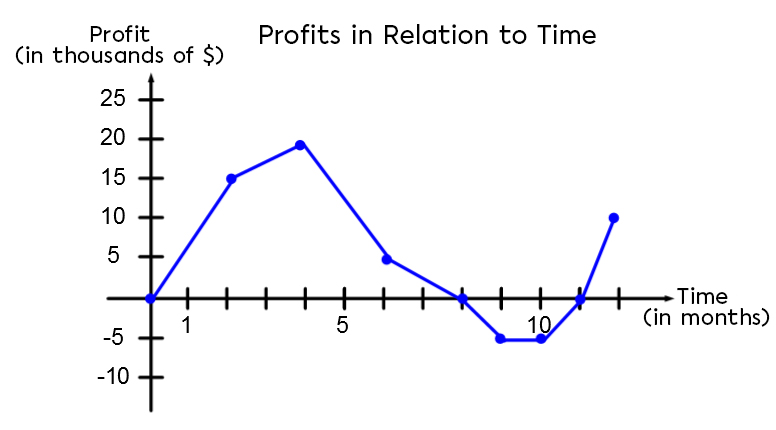
You must perform a complete study of this graph to prepare for your presentation speech and properly support your argument.
|
PROPERTIES |
EXPLANATIONS |
|---|---|
|
Domain: |[0, 12]| |
The smallest value on the |x|-axis is |0| and the biggest is |12.| |
|
Range: |[-5, 20]| |
The smallest value on the |y|-axis is |-5| and the biggest is |20.| |
|
Increasing: |[0, 4] \cup [9, 12]| |
By analyzing the |x| values, these are the two portions of the graph that go upwards or are constant. |
|
Decreasing: |[4, 10]| |
By analyzing the |x| values, it is the only portion of the graph that goes downwards or is constant. |
|
Maximum: |\left\{20 \right\}| |
According to the |y| values, this is the largest value reached by the graph. |
|
Minimum: |\left\{-5\right\}| |
According to the |y| values, it is the smallest value reached by the graph. |
|
X-intercepts (Zeros): |0,| |8| and |11| |
These are the coordinates of the points where the graph touches the |x|-axis. |
|
Y-intercept: |(0,0)| |
It is the coordinate of the point where the graph touches the |y|-axis. |
|
Positive: | [0,8] \cup [11,12]| |
Among the |x| values, these are the portions of the graph that are above or equal to the |x|-axis. |
|
Negative: |\{0\}\cup [8,11]| |
Among the |x| values, these are the portions of the graph that are below or equal to the |x|-axis. |
See also
We can follow these steps to solve a system of equations:
-
Identify the variables related to the unknown values.
-
Create the equations according to the situation.
-
Use the appropriate method to solve this system (comparison, substitution, or reduction) according to the form of the equations found.
-
Substitute the value of the found variable into one of the starting equations to find the value of the other variable.
To respect the constraints imposed by the city, an entrepreneur must divide his wooded lot into two different rectangular lots.
Given that the area of the lots must be equivalent, determine the possible dimensions, in decametres, of these two lots.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|x=| Missing measurement (in dam) |y=| Area of lot (in dam2) |
Identify the variables. |
|
|\begin{align} &\color{blue}{y = (2x-4) (x-7)} \\ &\color{red}{y = (x+8) (x-2)} \end{align}| |
Write a system of equations. |
|
|\begin{align} \color{blue}{(2x-4) (x-7)} &= \color{red}{(x+8)(x-2)} \\ \color{blue}{2x^2 - 18x + 28} &= \color{red}{x^2 + 6x - 16} \\ x^2 - 24x + 44 &= 0 \end{align}| |
Solve the system of equations (by comparison, substitution, or elimination). In this case, since the |y| is isolated in both equations, we can use the comparison method. |
| |\begin{align} x &= \dfrac{-b \pm \sqrt{b^2 - 4 a c}}{2a} \\ x &= \dfrac{-(-24) \pm \sqrt{(-24)^2 - 4 (1)(44)}}{2(1)} \\ x &= \dfrac{24 \pm 20}{2} \\ \Rightarrow\ x_1 &= \dfrac{24 + 20}{2} = 22\ \text{ and }\ x_2 = \dfrac{24 - 20}{2} = 2 \end{align}| |
Factor according to the appropriate method (quadratic formula, factoring by grouping, completing the square, etc.). |
|
|\begin{align} &\color{blue}{(x-7)(2x - 4)} \\ \rightarrow\ &\color{blue}{(22-7)\big(2(22) - 4\big)}= \color{blue}{15 \times 40} \end{align}| |\begin{align} &\color{red}{(x+8) (x-2)} \\ \rightarrow\ &\color{red}{(22+8) (22-2) } = \color{red}{30 \times 20} \end{align}| |
Determine the possible measurements based on |x_1 = 22.| |
|
|\begin{align} &\color{blue}{(x-7)(2x - 4)} \\ \rightarrow\ &\color{blue}{(2-7)\big(2 (2) - 4\big)} = \color{blue}{-5 \times 0} \end{align}| |\begin{align} &\color{red}{(x+8) (x-2)} \\ \rightarrow\ &\color{red}{(2+8) (2-2) } = \color{red}{10 \times 0} \end{align}| |
Determine the possible measurements based on |x_2 = 2.| |
|
Since the measurements obtained using the value of |x_2| are impossible in this context (negative or zero values), we can determine that the measurements of the first field are 15 dam by 40 dam, and those of the second are 20 dam by 30 dam. |
|
See also
Two figures are equivalent when they have the same area.
To make the cost of paving his new driveway the same as paving his old one, Julien wants the two driveways to be equivalent.
What should be the width of his new driveway?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\color{red}{A_\text{old}} = \color{blue}{A_\text{new}}| |
The two figures are equivalent. |
|
|\begin{align} \color{red}{A_\text{old}} &= \color{blue}{A_\text{new}} \\ \color{red}{b\times h} &= \color{blue}{b\times h} \\ \color{red}{8 \times 12} &= \color{blue}{b \times 10} \\ \color{red}{96} &= \color{blue}{10b} \\ 9{.}6\ \text{m} &= \color{blue}{b} \end{align}| |
Create an equation with the area formulas and solve. |
|
The width of the new driveway must be |9{.}6\ \text{m}.| |
|
See also
Two solids are equivalent when they have the same volume.
A company that works with outdoor gear wants to offer two different tent models. To keep production costs the same, they want these two models to be equivalent.
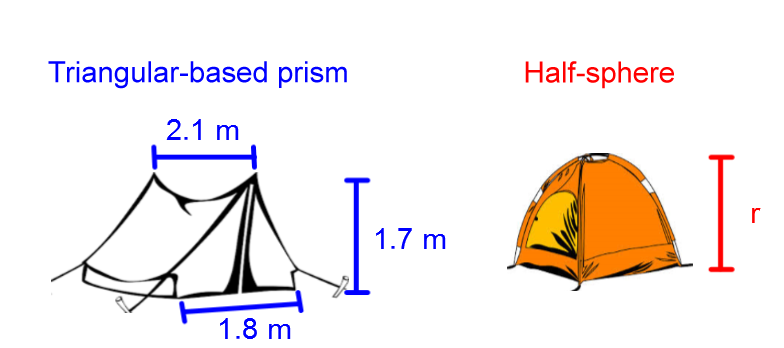
What must be the height of the second model for the tents to be equivalent?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\color{blue}{V_\text{prism}} = \color{red}{V_\text{half-sphere}}| |
The two solids are equivalent. |
|
|\begin{align} \color{blue}{A_b \times h} &= \color{red}{\dfrac{4 \pi r^3}{3} \div 2} \\ \color{blue}{\dfrac{1{.}8 \times 1{.}7}{2} \times 2{.}1} &= \color{red}{\dfrac{4 \pi r^3}{6}} \\ \color{blue}{3{.}21} &\approx \color{red}{\frac{4 \pi r^3}{6}} \\ 1{.}53 &\approx \color{red}{r^3} \\ 1{.}15\ \text{m} &\approx \color{red}{r} \end{align}| |
Create an equation with the respective volume formulas and solve it. |
|
The radius (height) of the half-sphere tent should be approximately |1{.}15\ \text{m}.| |
|
See also
Using the angle |\theta| as a reference, we have:
|\sin \theta = \dfrac{\text{Measure of the side opposite}\ \theta}{\text{Measure of the hypotenuse}}|
|\cos \theta = \dfrac{\text{Measure of the side adjacent}\ \theta}{\text{Measure of the hypotenuse}}|
|\tan \theta = \dfrac{\text{Measure of the side opposite} \ \theta}{\text{Measure of the side adjacent to} \ \theta}|
A house's roof trusses must have a minimum angle of elevation of |25^\circ| to meet building standards. To ensure that this constraint is respected, a manufacturer decides to establish this angle at |35^\circ.| If we know that the length of the roof truss is 13 metres, what are the measurements of the other two sides?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\sin \color{red}{35^\circ} = \dfrac{\color{red}{c}}{\color{green}{13}}| |
Identify the correct trigonometric ratio: |\sin \theta = \dfrac{\text{Opposite}}{\text{Hypotenuse}}| |
|
|\begin{align} \sin \color{red}{35^\circ} &= \dfrac{\color{red}{c}}{\color{green}{13}} \\ \color{green}{13}\sin \color{red}{35^\circ} &= \color{red}{c} \\ 7{,}46 &\approx \color{red}{c} \end{align}| |
Solve the equation. |
|
|\cos \color{red}{35^\circ} = \dfrac{\color{blue}{b}}{\color{green}{13}}| |
Identify the correct trigonometric ratio: |\cos \theta = \dfrac{\text{Adjacent}}{\text{Hypotenuse}}| |
|
|\begin{align} \cos \color{red}{35^\circ} &= \dfrac{\color{blue}{b}}{\color{green}{13}} \\ \color{green}{13} \cos \color{red}{35^\circ} &= \color{blue}{b} \\ 10{.}65 &\approx \color{blue}{b} \end{align}| |
Solve the equation. |
|
So, |\color{blue}{m \overline {AC}} \approx 10{.}65 \ \text{m}| and |\color{red}{m \overline {AB}} \approx 7{.}46 \ \text{m}.| |
|
To determine the route a helicopter should take to pick up distressed people in the forest, a map of the region has been triangulated with the current locations of the helicopter, the hospital, and the people in distress.
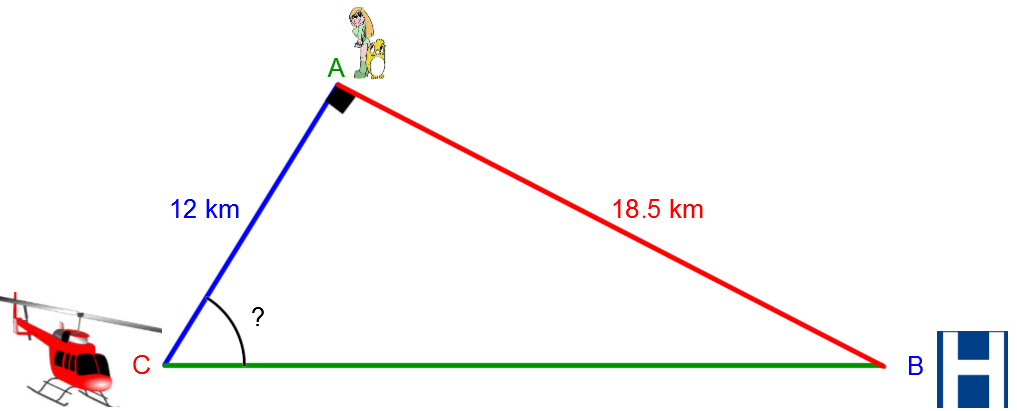
According to this drawing, what angle of orientation should the helicopter use to reach the people as quickly as possible?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\tan ? = \dfrac{\color{red}{18{.}5}}{\color{blue}{12}}| |
Identify the correct trigonometric ratio: |\tan \theta = \dfrac{\text{Opposite}}{\text{Adjacent}}| |
|
|\begin{align} \tan ? &= \dfrac{\color{red}{18{.}5}}{\color{blue}{12}} \\ \tan ? &\approx 1{.}54 \\ ? &= \tan^{-1}(1{.}54) \\ ? &\approx 57^\circ \end{align}| |
Solve the equation. |
|
The helicopter's angle of orientation should be |57^\circ.| |
|
See also
A series of equivalences can be deduced for any given triangle.
|\dfrac{a}{\sin A} = \dfrac{b}{\sin B} = \dfrac{c}{\sin C}|
Horse races are sometimes organized during western festivities to liven up the events. During these races, cowboys must ride around each of three barrels, which are arranged in the shape of an isosceles triangle.
Using the measurements provided, what is the distance between each of the barrels?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
| |
Identify the vertices and edges of the triangles. |
| |
If possible, deduce the other measurements of the triangle (using the sum of interior angles of a triangle and properties of the isosceles triangle). |
|
|\begin{align} \dfrac{\color{green}{a}}{\sin 40^\circ} &= \displaystyle \dfrac{\color{blue}{20}}{\sin \color{blue}{70^\circ}} \\\\ \Rightarrow\ \color{green}{a} &= \dfrac {\color{blue}{20} \sin 40^\circ}{\sin \color{blue}{70^\circ}} \\ \color{green}{a} &\approx 13{.}68 \ \text{m} \end{align}| |
Apply the law of sines and isolate the variable. |
|
So, |m \overline{AB} = m \overline {AC} = 20 \ \text{m}| and |m \overline {BC} \approx 13{.}68 \ \text{m}| |
|
To ensure maximum aerodynamics, the shape of some racing cars resembles a triangle.
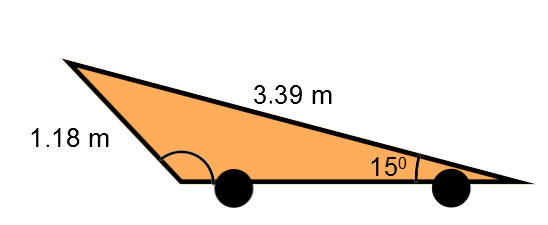
What should the angle closer to the back wheel measure for these proportions to be maintained?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
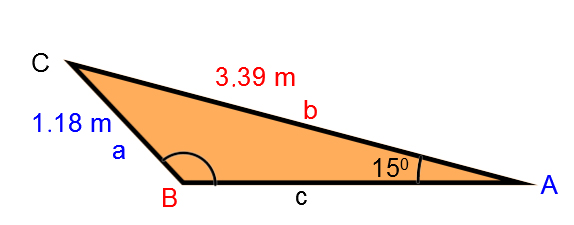 |
Identify the vertices and edges of the triangle. |
|
|\begin{align} \dfrac{\color{blue}{1{.}18}}{\sin \color{blue}{15}} &= \dfrac {\color{red}{3{.}39}}{\sin \color{red}{B}} \\\\ \Rightarrow\ \sin \color{red}{B} &= \dfrac{\color{red}{3{.}39} \sin \color{blue}{15}}{\color{blue}{1{.}18}} \\ \sin \color{red}{B} &\approx 0{.}744 \end{align}| |
Use the law of sines and isolate the sine of the desired angle. |
|
|\begin{align} \sin \color{red}{B} &\approx 0{.}744 \\ \color{red}{ B} &\approx \sin^{-1} (0{.}744) \\ \color{red}{B} &\approx 48{.}1^\circ \end{align}| |
Calculate the value of the variable using |\sin^{-1}.| |
|
|\begin{align} \color{red}{m\angle B} &\approx 180^\circ - 48{.}1^\circ \\ \color{red}{m\angle B} &\approx 131{.}9^\circ \end{align}| |
Find the obtuse angle’s value. |
|
The angle’s measurement is |131{.}9^\circ.| |
|
When identifying triangles, it is always essential to identify
-
|\color{green}{\text{side a opposite to angle A}}|
-
|\color{red}{\text{side b opposite to angle B}}|
-
|\color{blue}{\text{side c opposite to angle C}}|
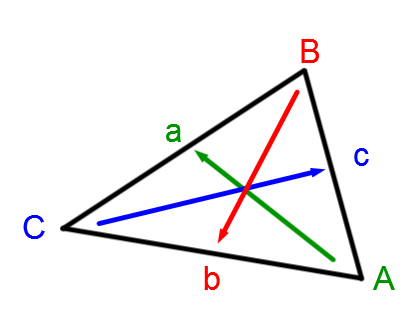
See also
A series of equivalences can be deduced for any given triangle.
|a^2 = \color{blue}{b}^2 + \color{red}{c}^2 - 2 \color{blue}{b} \color{red}{c} \cos A|
|\color{blue}{b}^2 = a^2 + \color{red}{c}^2 - 2 a \color{red}{c} \cos \color{blue}{B}|
|\color{red}{c}^2 = a^2 + \color{blue}{b}^2 - 2 a \color{blue}{b} \cos \color{red}{C}|
A hunter, who uses a bow, settles in the corner of his land to maximize his chances of hunting a moose. The following triangle shows the range of his arrows:
Based on the information provided, what |\color{red}{\text{distance}}| can the moose walk while staying as far as possible from the hunter?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
| |
Identify the vertices and edges of the triangle. |
|
|\begin{align} \color{red}{a}^2 &= \color{blue}{b}^2 + \color{green}{c}^2 - 2\color{blue}{b} \color{green}{c} \cos \color{red}{A} \\ \color{red}{a}^2 &= \color{blue}{92}^2 + \color{green}{125}^2 - 2 \color{blue}{(92)} \color{green}{(125)} \cos \color{red}{81^\circ} \end{align}| |
Apply the appropriate formula so there is only one unknown. |
|
|\begin{align} \color{red}{a}^2 &= \color{blue}{92}^2 + \color{green}{125}^2 - 2 \color{blue}{(92)} \color{green}{(125)} \cos \color{red}{81^\circ} \\ \color{red}{a}^2 &\approx 8\ 464 + 15\ 625 - 3\ 598 \\ \color{red}{a}^2 &\approx 20\ 491 \\ \color{red}{a}\ &\approx 143{.}15 \end{align}| |
Solve the equation by isolating the variable. |
|
The moose can walk a |\color{red}{\text{distance}}| of about |143{.}15\ \text{m}.| |
|
A bank is installing a rotating surveillance camera in its entrance hall to keep its employees safe. In addition, a security guard is in charge of monitoring the same area, defined by the following triangle:
What should the camera's rotation angle measure to ensure that there are no blind spots?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
| |
Identify the vertices and edges of the triangle. |
|
|\begin{align} \color{blue}{a^2} &= \color{red}{b^2} + \color{green}{c^2} - 2 \color{red}{b} \color{green}{c} \cos \color{blue}{A} \\ \color{blue}{22^2} &= \color{red}{24^2} + \color{green}{21^2} - 2 \color{red}{(24)} \color{green}{(21)} \cos \color{blue}{A} \end{align}| |
Substitute in the values of the formula. Here we use |a^2 = b^2 + c^2 - 2bc \cos A| since we are looking for the measurement of angle |A|. |
|
|\begin{align} \color{blue}{22^2} &= \color{red}{24^2} + \color{green}{21^2} - 2 \color{red}{(24)} \color{green}{(21)} \cos \color{blue}{A} \\ \color{blue}{484} &=576+441 - 1 \ 008 \cos \color{blue}{A}\\\\ \Rightarrow\ \dfrac{484 - 576-441}{- 1 \ 008} &= \cos \color{blue}{A} \\ 0{.}529 &\approx \cos \color{blue}{A} \\ 58^\circ &\approx \color{blue}{m\angle A} \end{align}| |
Isolate the variable. |
|
The camera should rotate through an angle of approximately |58^\circ| to ensure there are no blind spots. |
|
See also
To achieve this, add lines (usually a height) with particular properties and unknown measures.
-
Choose the correct vertex from which to draw a height.
-
Use the trigonometric ratios in the right triangle to find the missing measurements.
-
Apply the formula for the area of a triangle with the found measurements.
Find the area of the following triangle:
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
| |
If possible, find all angle measurements (sum of interior angles of a triangle |= 180^\circ|). |
| |
Sketch a height and make it the unknown in the trigonometric ratio used. |
| |
Identify the right triangle formed. |
|
|\begin{align} \sin \color{red}{24^\circ} &= \dfrac{\color{blue}{h}}{42} \\ 17{.}08 &\approx \color{blue}{h} \\\\ \cos \color{red}{24^\circ} &= \dfrac{\color{green}{b}}{42} \\ 38{.}37 &\approx \color{green}{b} \end{align}| |
Find as many measurements as possible using trigonometric ratios. |
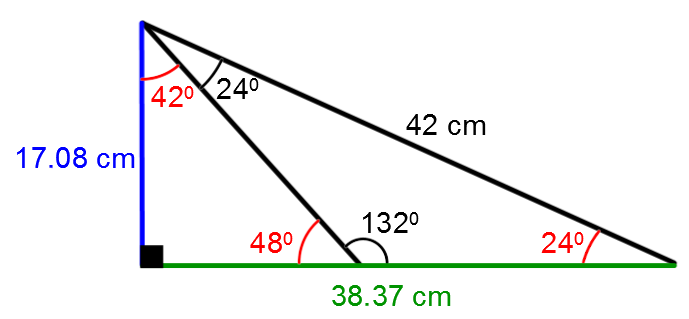 |
Find the missing angle measurements of the smaller left triangle. |
|
|\begin{align} \tan \color{red}{48^\circ} &= \dfrac{\color{blue}{17{.}08}}{\color{green}{2^\text{nd}\text{ leg}}} \\ 15{.}38 &\approx \color{green}{2^\text{nd}\text{ leg}} \end{align}| |
Find the measurement of the 2nd leg of the small triangle. |
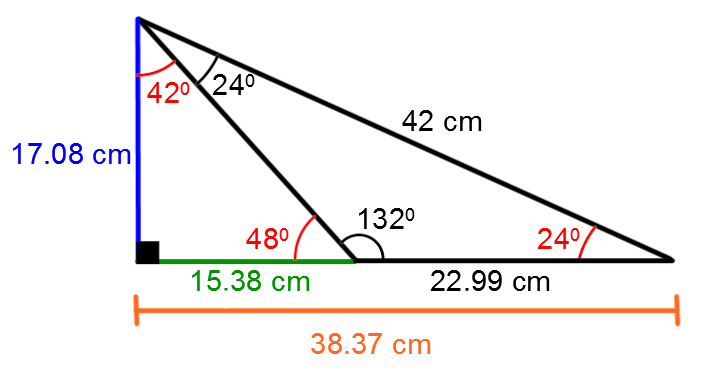 |
Determine the measurement of the base of the initial triangle. |
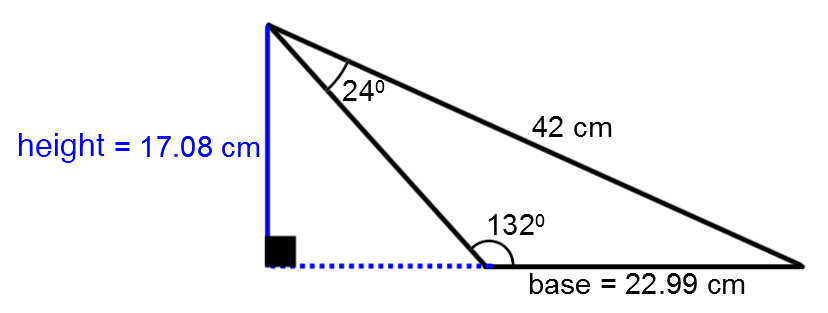 |
Identify the base and height needed to calculate the area of the triangle. |
|
|\begin{align} A &= \dfrac{b \times \color{blue}{h}}{2} \\ A &= \dfrac{22{.}99 \times \color{blue}{17{.}08}}{2} \\ &\approx 196{.}33 \ \text{cm}^2 \end{align}| |
Calculate the area of the triangle according to its formula. |
|
The area of the triangle is approximately |196{.}33 \ \text{cm}^2.| |
|
See also
-
A - S - A: Two triangles are congruent when a pair of corresponding congruent sides is located between two pairs of corresponding congruent angles.
-
S - A - S: Two triangles are congruent when a pair of corresponding congruent angles is located between two pairs of corresponding congruent sides.
-
S - S - S: Two triangles are congruent when all pairs of corresponding sides are congruent.
Due to machinery problems, construction workers need to complete the construction of a house by assembling the triangular-shaped roof trusses themselves. All of the trusses must be identical.
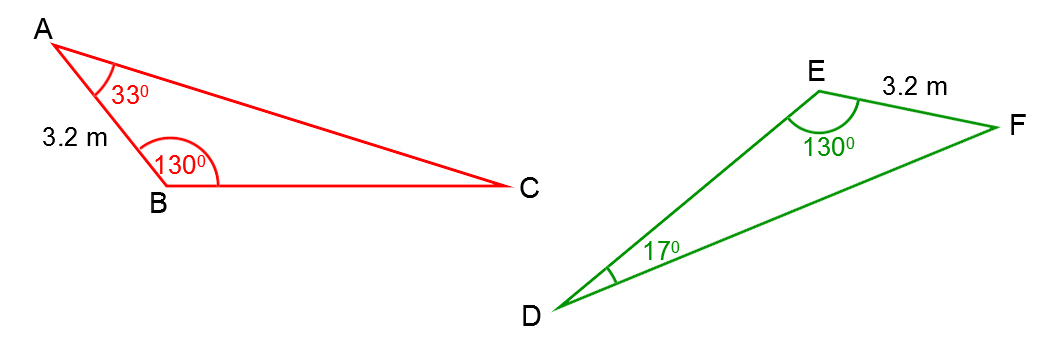
With the information provided above, demonstrate that these two constructions are congruent.
|
CONFIRMATIONS |
RATIONALE |
|---|---|
|
|\angle \color{red}{BAC} \cong \angle \color{green}{EFG}| |
|m \angle \color{green}{EFG} = 180^\circ - 130^\circ - 17^\circ = 33^\circ = m \angle \color{red}{BAC}| |
|
|\color{red}{\overline{AB}} \cong \color{green}{\overline{EF}}| |
By hypothesis |
|
|\angle \color{red}{ABC} \cong \angle \color{green}{DEF}| |
By hypothesis |
|
The |\Delta \color{red}{ABC} \cong \Delta \color{green}{DEF}| by the minimal condition of congruent triangle A-S-A. |
|
See also
-
A - A: Two triangles are similar when two pairs of corresponding angles are congruent.
-
S - A - S: Two triangles are similar when a pair of corresponding congruent angles is located between two pairs of proportional corresponding sides.
-
S - S - S: Two triangles are similar if all three pairs of corresponding sides are proportional.
The city is organizing a family run as part of a fundraiser for a community organization. They want the adults to take a path similar to that of the children.
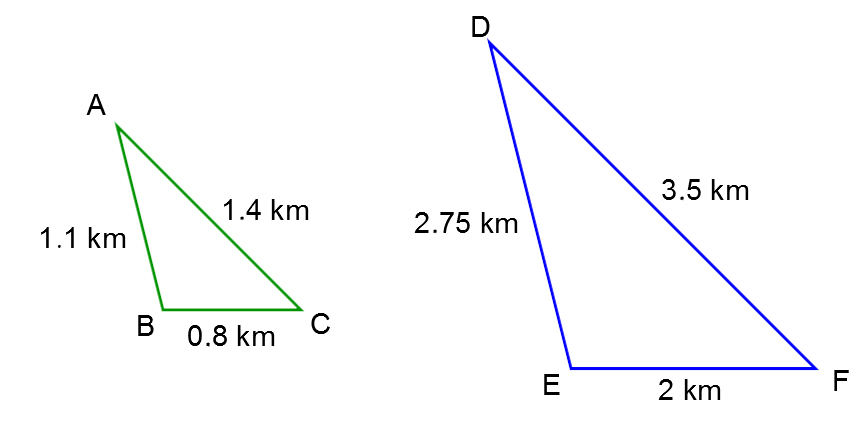
Taking into account the information provided, demonstrate that the two paths are similar.
|
AFFIRMATIONS |
JUSTIFICATIONS |
|---|---|
|
|\dfrac{m\color{green}{\overline{AC}}}{m \color{blue}{\overline {DF}}} = \dfrac{m\color{green}{\overline{AB}}}{m \color{blue}{\overline {DE}}}= \dfrac{m\color{green}{\overline{BC}}}{m \color{blue}{\overline {EF}}}| |
|\begin{align} \dfrac{\color{green}{1{.}4}}{\color{blue}{3{.}5}} &= \dfrac{\color{green}{1{.}1}}{\color{blue}{2{.}75}}= \dfrac{\color{green}{0{.}8}}{\color{blue}{2}} \\ \dfrac{2}{5}\ \ &=\ \ \dfrac{2}{5}\ \ \ =\ \ \dfrac{2}{5}\end{align}| |
|
Therefore, |\Delta \color{green}{ABC} \sim \Delta \color{blue}{DEF}| by the minimal condition of similar triangle S-S-S. |
|
See also
From the following right-angled triangle, we can deduce three theorems from the following right-angled triangle.
-
In a right triangle, the measurement of each side of the right angle is the geometric mean between its projection onto the hypotenuse and the hypotenuse itself.||\begin{align} \dfrac{m}{a} = \dfrac{a}{c}\ &\Leftrightarrow\ a^2 = m c \\\\ \dfrac{n}{b} = \dfrac{b}{c}\ &\Leftrightarrow\ b^2 = n c \end{align}||
-
In a right triangle, the height measurement (altitude) is the geometric mean of the projections of the sides onto the hypotenuse. ||\dfrac{m}{h} = \dfrac{h}{n}\ \Leftrightarrow\ h^2 = m n||
-
In a right triangle, the product of the measurements of the hypotenuse and the height (altitude) equal the product of the measurements of the sides of the right angle. ||c h = a b||
To stand out from other contractors, a construction company suggests houses with roofs of different shapes. Among these choices, we have the following shape:
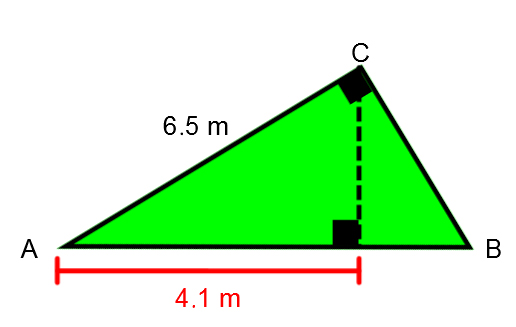
To estimate production costs, the company needs the two missing outer measurements of triangle |(\overline {AB}, \overline {BC}).| Help determine them.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align} &a\; = 6{.}5 \\ &m = 4{.}1 \\ &c \ (m\overline {AB}) =\ ? \\ &b \ (m\overline {BC}) =\ ? \end{align}| |
Associate all known and wanted measurements with one of the measurements in the reference drawing. |
|
|\begin{align} a^2 &= m c \\ 6{.}5^2 &= 4{.}1 c \end{align}| |
Choose the theorem where there will be only one unknown: In a right triangle, the measurement of each side of the right angle is the geometric mean between its projection onto the hypotenuse and the hypotenuse itself. |
|
|\begin{align} 6{.}5^2 &= 4{.}1 c \\ 42{.}25 &= 4{.}1 c \\ \dfrac{42{.}25}{\color{red}{4{.}1}} &= \dfrac{4{.}1c}{\color{red}{4{.}1}} \\ 10{.}3 &\approx c \end{align}| |
Solve the equation. |
|
|\begin{align} a^2 + b^2 &= c^2 \\ 6{.}5^2 + m\overline {BC}^2 &= 10{.}3^2 \\ 42{.}25 + m\overline {BC}^2 &\approx 106{.}09 \\ m\overline {BC}^2 &\approx 63{.}84 \\ m\overline {BC} &\approx 8 \end{align}| |
Apply the Pythagorean theorem on the large green right triangle to find the measurement of the missing leg. |
|
So, |m \overline {AB} \approx 10{.}3 \ \text{m}| and |m \overline {BC} \approx 8 \ \text{m}.| |
|
See also
|\text{Distance} = \sqrt{(y_2 - y_1)^2 + (x_2 - x_1)^2}|
where
|(x_1, y_1):| Coordinates of the starting point of the segment
|(x_2, y_2):| Coordinates of the ending point of the segment
To determine the amount of gasoline an airplane needs to complete a Montreal-Paris flight, the cities are represented on a Cartesian plane (graduated in kilometres).
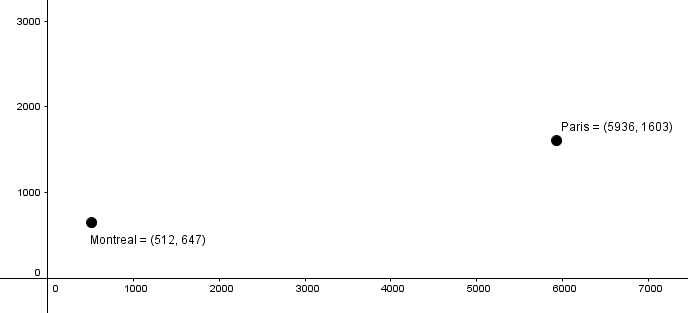
What is the distance between these two cities (in kilometres)?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
Montreal |= (\color{blue}{x_1}, \color{red}{y_1}) = (\color{blue}{512}, \color{red}{647})| |
Identify the points |(x_1, y_1)| and |(x_2, y_2).| |
|
|\begin{align} \text{Distance} &= \sqrt{(y_2 - \color{red}{y_1})^2 + (\color{green}{x_2} - \color{blue}{x_1})^2} \\ \text{Distance} &= \sqrt{(1\ 603 - \color{red}{647})^2 + (\color{green}{5\ 936} - \color{blue}{512})^2} \end{align}| |
Substitute the values into the formula. |
|
|\begin{align} \text{Distance} &= \sqrt{(1\ 603 - \color{red}{647})^2 + (\color{green}{5\ 936} - \color{blue}{512})^2} \\ \text{Distance} &= \sqrt{ 956^2 + 5\ 424^2} \\ \text{Distance} &\approx 5\ 507{.}6 \ \text{km} \end{align}| |
Solve the equation. |
|
The distance between Montreal and Paris is approximately |5\ 507{.}6 \ \text{km}.| |
|
See also
The lines |y_1 = a_1 x + b_1| and |y_2 = a_2 x + b_2| are parallel if and only if |a_1 = a_2.|
What is the equation of the line parallel to that identified in the Cartesian plane below and which passes through point C?
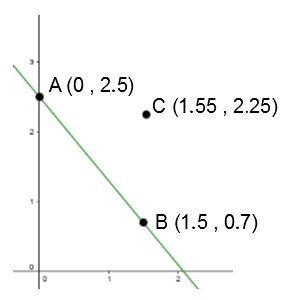
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|a = \dfrac{\Delta y}{\Delta x} = \dfrac{0{.}7 - 2{.}5}{1{.}5 - 0} = -1{.}2| |
Find the slope of |\overline{AB}.| |
|
|\begin{align} y &= -1{.}2x + b \\ 2{.}25 &= -1{.}2 (1{.}55) + b \\ 2{.}25 &= -1{.}86 + b \\ 4{.}11 &= b \end{align}| |
Find the equation of the line passing through |C (1{.}55, 2{.}25)| of the form |y = ax + b.| In this case, the value of |a| of the desired equation is the same as that of |\overline{AB}| since the lines are parallel. |
|
Finally, the equation of the line parallel to |\overline{AB}| and which passes through the point | C (1{.}55, 2{.}25)| is |y = -1{.}2x + 4{.}11.| |
|
The lines |y_1 = a_1 x + b_1| and |y_2 = a_2 x + b_2| are perpendicular if and only if |a_1 \times a_2 = -1.|
Two lines are also perpendicular if the slope of one is the opposite of the reciprocal of the slope of the other: |a_2 = \dfrac{-1}{a_1}.|
What is the equation of the line perpendicular to that in the Cartesian plane below and which passes through point C?
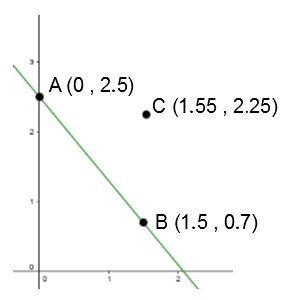
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|a_1 = \dfrac{\Delta y}{\Delta x} = \dfrac{0{.}7 - 2{.}5}{1{.}5 - 0} = -1{.}2| |
Find the slope of |\overline{AB}.| |
|
|\begin{align} a_1 \times a_2 &= -1 \\-1{.}2 \times a_2 &= -1 \\ a_2 &= \dfrac{-1}{-1{.}2} \\ a_2 &= 0{.}8\overline{3} \end{align}| |
Find the value of |a_2| of the line passing through C, using the fact that the product of the slopes of two perpendicular lines is equal to |-1.| |
|
|\begin{align} y &= a_2 x + b \\ y &= 0{.}8\overline{3}x + b \\ 2{.}25 &= 0{.}8\overline{3} (1{.}55) + b \\ 2{.}25 &\approx 1{.}29 + b \\ 0{.}96 &\approx b \end{align}| |
Find the rule of the line passing through |C (1{.}55, 2{.}25)| |
|
Finally, the equation of the line perpendicular to |\overline{AB}| and which passes through point |C(1{.}55, 2{.}25)| is |y=0{.}8\overline{3}x + 0{.}96.| |
|
See also
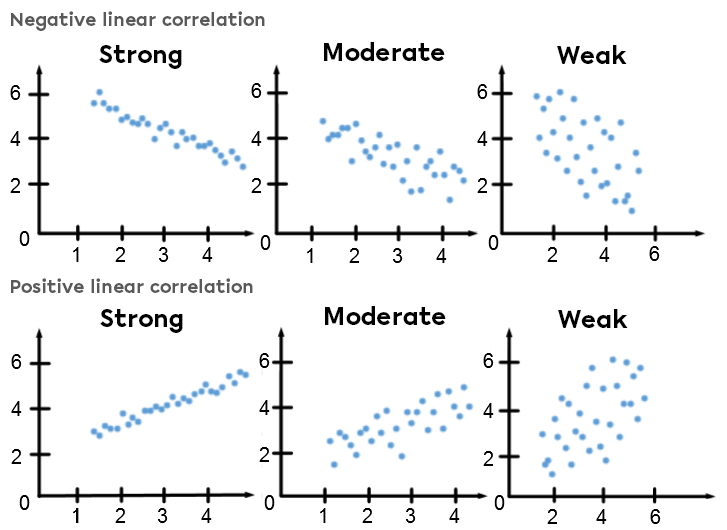
The scatter plot is used to estimate the correlation between two variables. It is necessary to calculate the correlation coefficient to get a more precise idea of the correlation.
A new company has increased its profits for five years and seeks to expand its production centre. However, the owners want to ensure that their company’s economic growth is positive and strong. To break it all down, here's a count of business income for the past 30 weeks.
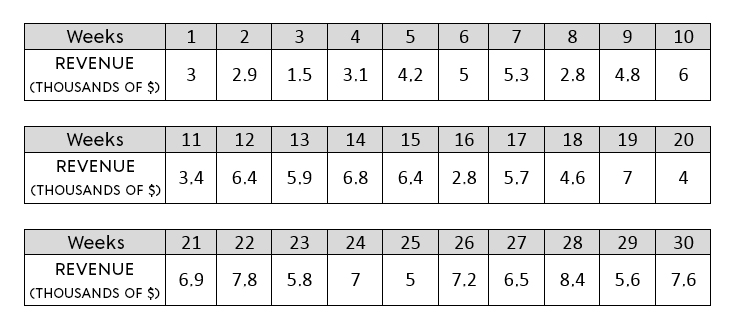
In your opinion, is the economic growth of the company positive and strong?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
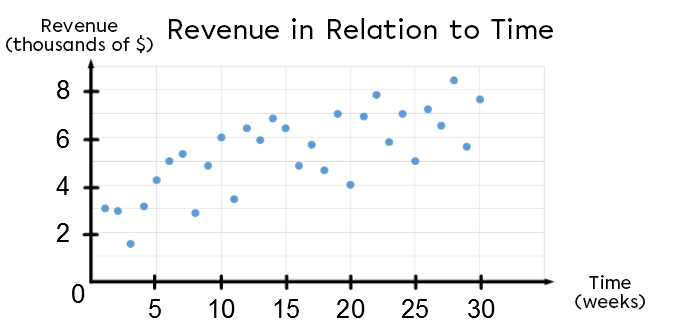 |
Construct the scatter plot. |
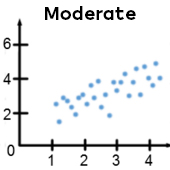 |
Compare the scatter plot to those that serve as a reference. |
|
According to the scatter plot, we can conclude that the correlation is average positive. Since it is not a strong correlation, it would be better to wait before expanding. |
|
See also
After framing the scatter plot and taking the length measurement |(l)| and the width |(w)| of the rectangle:
||r = \pm \left(1 - \dfrac{w}{l}\right)||
The sign will depend on the direction of the scatter plot (increasing or decreasing).
This coefficient can also be used to qualify the correlation:
| Value |r| | Linear correlation strength |
|---|---|
| Near |0| | Zero |
| Near |\pm 0{.}50| | Weak |
| Near |\pm 0{.}75| | Average |
| Near |\pm 0{.}87| | Strong |
| Near |\pm 1| | Very strong |
| |\pm 1| | Perfect |
To understand the success of students who enroll in adult education, an administration team is interested in the correlation between absenteeism (in hours) and final grades (in %). They grouped the data into a scatter plot to properly analyze the situation:
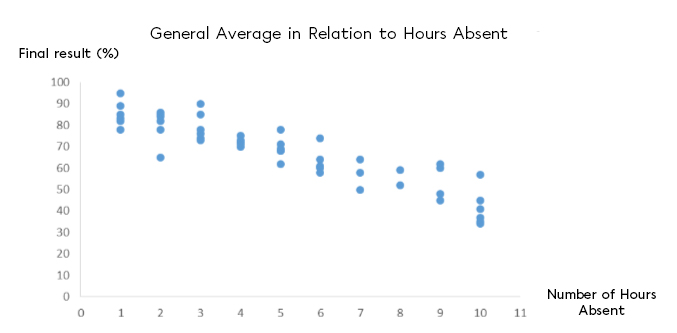
What is the correlation coefficient of this study?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
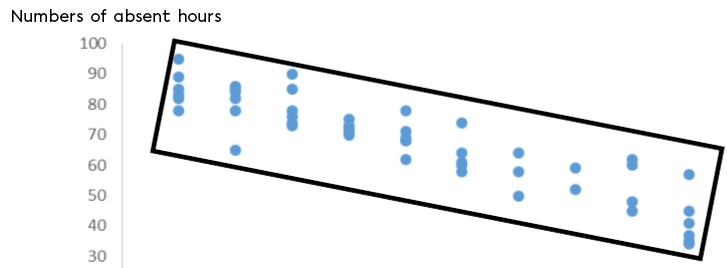 |
Draw a rectangle – as small as possible – to frame the scatter plot. |
| |
Using a ruler, measure the length |(l)| and width |(w)| of the rectangle. In this case, |\color{blue}{l = 13{.}5 \ \text{cm}}| |\color{red}{w = 2{.}8 \ \text{cm}}| |
|
|\begin{align} r &\approx \pm \left(1 - \dfrac{\color{red}{2{.}8}}{\color{blue}{13{.}5}}\right) \\ r &\approx \pm 0{.}79 \end{align}| |
Replace |l| and |w| in the formula |r \approx \pm \left(1 - \frac{\color{red}{w}}{\color{blue}{l}}\right).| |
|
|r \approx - 0{.}79| |
Since the rectangle is oriented downward (decreasing), the correlation coefficient is negative. |
|
The correlation coefficient between the number of absent hours and the final result in percentage is approximately |-0{.}79,| which means it is an average, negative correlation. |
|
See also
Follow these steps to find the equation of the regression line according to the median-median method:
-
Arrange the points in ascending order according to the value of |x.|
-
If possible, divide the pairs into three equal groups.
-
Calculate the median coordinates |(M_1, M_2, M_3)| of each group.
-
Calculate the mean coordinates |(P_1)| of the three midpoints.
-
Calculate the value of the slope |(a)| with |M_1| and |M_3.|
-
Calculate the value of the initial value |(b)| with |P_1.|
-
Write the equation of the regression line in the form |y = ax + b.|
Before a new condo tower is built and the landscaping is done, the height of the neighbouring trees is measured so they do not obscure the view for the next 20 years. The following table of values is used to estimate the height of the trees:
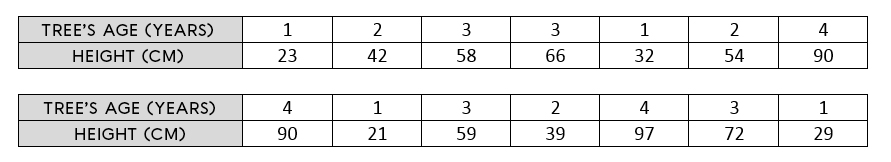
Using this information, determine how high the first balconies need to be for the view to remain unobstructed.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
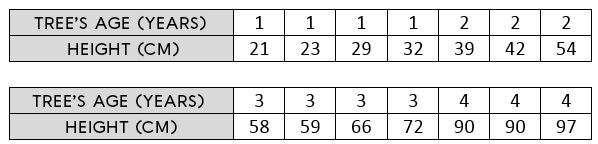 |
Place the pairs in ascending order according to the value of the independent variable |(x)|, taking care not to “undo” the initial ordered pairs. |
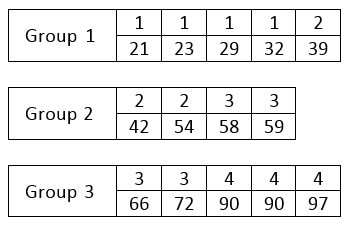 |
Separate the points into three equal groups. If this is not possible, make sure that the first and the last group have the same amount of data. |
|
|M_1 = (1, 29)| |M_2 = (\dfrac{2+3}{2}, \dfrac{54+58}{2}) = (2{.}5 ; 56)| |M_3 = (4, 90)| |
Calculate the median coordinate of each group. |
|
|\begin{align} \color{green}{P} &= \left(\frac{1 + 2{.}5 + 4}{3}, \frac{29 + 56 + 90}{3}\right) \\ &= \color{green}{(2{.}5, 58{.}33)} \end{align}| |
Calculate the mean point by averaging the |x| and |y| of the three midpoints. |
|
|\color{blue}{a} = \dfrac{\Delta y}{\Delta x} = \dfrac{90 - 29}{4 - 1} \approx 20{.}33| |
According to the equation of regression line |y = \color{blue}{a}x + \color{red}{b}|, determine the value of |\color{blue}{a}| from points |M_1| and |M_3.| |
|
|\begin{align} y &= \color{blue}{20{.}33} x + \color{red}{b}\\ \color{green}{58{.}33} &= \color{blue}{20{.}33}(\color{green}{2{.}5}) + \color{red}{b} \\ \color{red}{7{.}503} &= \color{red}{b} \end{align}| Thereby, |y = \color{blue}{20{.}33} x + \color{red}{7{.}503}| |
Find the value of parameter |\color{red}{b}| by substituting |x| and |y| with the coordinates of point |\color{green}{P}.| |
|
|\begin{align} y &= \color{blue}{20{.}33} x + \color{red}{7{.}503} \\ y &= \color{blue}{20{.}33} (20) + \color{red}{7{.}503} \\ y &= 414{.}103 \end{align}| |
Since we want to know the height of the trees after |20| years, we substitute |x| with |20.| |
|
After |20| years, the trees will be about |414{.}103\ \text{cm}.| Thus, the first balconies must have a minimum height of |414{.}103\ \text{cm}.| |
|
Although the situation and data are the same, it is normal that the final answer varies depending on the method used (Median-Median method or Mayer method).
Since these methods are used to estimate and not to predict outcomes with certainty, there may be a difference between the two outcomes.
See also
Follow these steps to find the equation of the regression line according to Mayer’s method:
-
Arrange the pairs in ascending order according to the value in |x.|
-
If possible, divide the pairs into two equal groups.
-
Calculate mean points |(P_1| and |P_2)| of each group.
-
Use these mean points to find the value of slope |(a)| and the initial value |(b).|
-
Write the equation of the regression line in the form |y = ax + b.|
Before a new condo tower is built and the landscaping is done, the height of the neighbouring trees are measured so they do not obscure the view for at least 20 years. The following table of values is used to estimate the height of the trees:
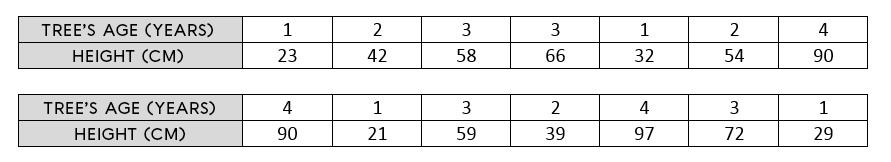
Using this information, determine how high the first balconies need to be for the view to remain unobstructed.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
 |
First, arrange the points in ascending order according to the value of the independent variable without undoing the initial ordered pairs. |
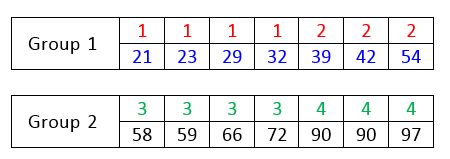 |
If possible, separate the distribution into two equal groups. |
|
|\begin{align} P_1 &= \left(\dfrac{\color{red}{1+1+... +2+2}}{7}, \dfrac{\color{blue}{21+23+...+42+54}}{7}\right) \\ &\approx (\color{red}{1{.}43} ,\color{blue}{34{.}29}) \\\\ P_2 &= \left(\dfrac{\color{green}{3+3...+4+4}}{7}, \dfrac{58+59+...+90+97}{7}\right) \\ &\approx (\color{green}{3{.}43} , 76) \end{align}| |
Calculate the mean points of each group. |
|
|a = \dfrac{\Delta y}{\Delta x} = \dfrac{76 - \color{blue}{34{.}29}}{\color{green}{3{.}43} - \color{red}{1{.}43}} \approx 20{.}86| Thereby, |y = 20{.}86x + b.| Substitute the coordinates of |P_1:| ||\begin{align} y &= 20{.}86x + b \\ 34{.}29 &= 20{.}86 (1{.}43) + b \\ 34{.}29 &= 29{.}83 + b \\ 4{.}46 &= b \end{align}|| Thereby, |y = 20{.}86x + 4{.}46.| |
Find the equation of the regression line in the form |y = ax + b| according to points |P_1| and |P_2.| |
|
|\begin{align} y &= 20{.}86x + 4{.}46 \\ y &= 20{.}86 (20) +4{.}46 \\ y &= 417{.}2 + 4{.}46 \\ y &= 421{.}66 \end{align}| |
Since we want to know the height of the trees after |20| years, we substitute |x| with |20.| |
|
After |20| years, the trees will be about |421{.}66\ \text{cm}.| Thus, the first balconies must be of a minimum height of |421{.}66\ \text{cm}.| |
|
Although the situation and data are the same, it is normal that the final answer varies depending on the approach used (Median-Median method or Mayer method).
Since these methods are used to estimate and not to predict outcomes with certainty, there may be a difference between the two outcomes.