Here is a short study guide containing all the concepts covered in secondary 4 in the TS pathway. For further explanation, each formula will be followed by an example and a link to a concept sheet in our virtual library.
Dividing polynomials is done the same way as dividing two numbers using long division.
Find the result of the following division:
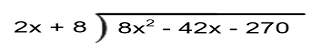
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
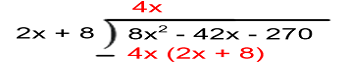 |
Determine the monomial that you must multiply |2x+8| by in order to cancel the |x^2| term of the polynomial |
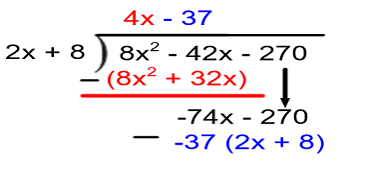 |
Determine the monomial that you must multiply |2x+8| by in order to cancel the |x| of the polynomial. |
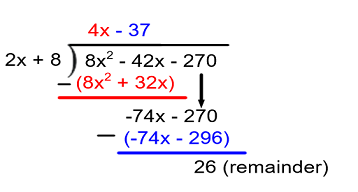 |
Identify the remainder when the degree of the subtraction’s result is smaller than the binomial in the divisor. |
|
The result of the division is |4x-37| remainder |26,| or |4x-37+\dfrac{26}{2x+8}.| |
|
See also
Follow these steps to add or subtract rational expressions:
-
Find a common denominator.
-
Calculate equivalent fractions according to the common denominator.
-
Complete the addition or subtraction of like terms with the numerators.
-
If possible, simplify the final rational expression by factoring the numerator and denominator
Simplify the following algebraic expression: ||\dfrac{x-2}{x+4} - \dfrac{3}{-3x-12}||
-
Find a common denominator and calculate the equivalent fractions according to the denominator. ||\begin{align} &\dfrac{x-2}{x+4} - \dfrac{3}{-3x-12} \\ =\ &\dfrac{\color{red}{-3} (x-2)}{\color{red}{-3} (x+4)} - \dfrac{3}{-3x-12} \\ =\ &\dfrac{-3x+6}{-3x-12} - \dfrac{3}{-3x-12}\end{align}||
-
Complete the operation with the numerators. ||\begin{align} &\dfrac{-3x+6}{-3x-12} - \dfrac{3}{-3x-12} \\ =\ &\dfrac{(-3x+6) - (3)}{-3x-12} \\ =\ &\dfrac{-3x+3}{-3x-12} \end{align}||
-
If possible, simplify the rational expression by factoring. Here, we can factor out a common factor from both the numerator and denominator. ||\begin{align} &\dfrac{-3x+3}{-3x-12} \\ =\ &\dfrac{-3(x-1)}{-3(x + 4)} \\ =\ &\dfrac{x-1}{x+4} \end{align}||
Answer: The result of the difference of these two rational expressions is |\dfrac{x-1}{x+4}.|
See also
It is important to master the distributive concept associated with multiplication:
-
If possible, simplify the expressions in brackets.
-
Distribute each of the terms in the first bracket to all the terms in the second bracket.
-
Simplify by adding and subtracting like terms.
Find the simplified algebraic expression for the following multiplication: ||(7x + 4) (2x^2 -4x +3)||
-
Distribute each of the terms in the first bracket with the terms in the second bracket. ||\begin{align} &(\color{blue}{7x} + \color{red}{4}) (2x^2 - 4x + 3) \\ =\ &\color{blue}{7x} \times 2x^2 + \color{blue}{7x} \times -4x + \color{blue}{7x} \times 3 + \color{red}{4} \times 2x^2 + \color{red}{4} \times -4x + \color{red}{4} \times 3 \\ =\ &14x^3 +-28x^2 + 21x + 8x^2 +-16x +12 \end{align}||
-
Complete addition and subtraction of like terms. ||\begin{align} &14x^3 +\color{blue}{-28x^2} + \color{green}{21x}+\color{blue}{8x^2} + \color{green}{-16x} + 12 \\ =\ &14x^3 + \color{blue}{-20x^2} + \color{green}{5x} + 12 \end{align}||
The simplified algebraic expression is |14x^3 - 20x^2 + 5x +12.|
See also
Sometimes several factorization methods are required to factor the same algebraic expression, and that is why it is important to master them all.
|
EXAMPLE OF THE PRODUCT-SUM METHOD |
|
|---|---|
|
What measurements (in numerical form or as an algebraic expression) are associated with each of the dimensions of a rectangular prism whose volume is |(4x^2 + 8x - 32 )\ \text{cm}^3|? |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} &4x^2 + 8x - 32 \\ =\ &4 (x^2 + 2x - 8) \end{align}| |
If possible, factor out a greatest common factor, making sure that all coefficients remain whole. |
|
|4 (\color{blue}{x}^2 + \color{red}{2x} \color{green}{-8})| The numbers are |4| and |-2.| |
Determine the numbers that correspond to the product and the sum of the polynomial in brackets. |
|
|\begin{align} &4 (x^2 + \color{red}{2x} - 8) \\ =\ &4 (x^2 + \color{red}{4x -2x} - 8) \\ =\ &4(\left[x^2 + 4x\right] +\left[-2x -8\right]) \\ =\ &4 (\color{blue}{x} (\color{green}{x + 4}) \color{blue}{-2} (\color{green}{x + 4})) \\ =\ &4 (\color{green}{x+4}) (\color{blue}{x-2}) \end{align}| |
Separate the |\color{red}{x}| term into two terms using the two found numbers and then factor using the Grouping method. |
|
The three dimensions measure |4,(x+4),| and |(x-2)\ \text{cm}.| |
|
|
EXAMPLE OF A DIFFERENCE OF SQUARES |
|
|---|---|
|
What are the algebraic expressions that represent the measurement of the base and the height of a triangle whose area is |(2x^2-8)\ \text{m}^2|? |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} \dfrac{\color{blue}{b} \times \color{red}{h}}{2} &= 2x^2 - 8 \\ \color{blue}{b} \times \color{red}{h} &= 4x^2 - 16 \end{align}| |
Create the equation that represents the situation. |
|
|\sqrt{4x^2} = 2x| This is a subtraction between two terms. |
Verify that the binomial meets the criteria of a difference of squares. |
|
|\begin{align} \color{blue}{b} \times \color{red}{h} &= 4x^4 - 16 \\ \color{blue}{b} \times \color{red}{h} &= \color{blue}{(2x - 4)} \color{red}{ (2x + 4)} \end{align}| |
Factor according to this model. |
|
Therefore, we can conclude that |\color{blue}{b=(2x-4)}| and |\color{red}{h=(2x+4)}\ \text{m}.| |
|
|
EXAMPLE OF A PERFECT SQUARE TRINOMIAL |
|
|---|---|
|
What is the algebraic expression associated with the measurement of a square’s edge that has an area of |(\color{blue}{9}x^2 - \color{red}{42}x +\color{green}{49})\ \text{m}^2?| |
|
|
CALCULATIONS |
EXPLANATIONS |
|
|\begin{align} \sqrt{\color{blue}{a}} &= \sqrt{\color{blue}{9}} = \color{blue}{3} \\ \sqrt{\color{green}{c}} &= \sqrt{\color{green}{49}} = \color{green}{7} \\\\ \color{red}{b} &\overset{?}{=} 2 \sqrt{\color{blue}{a}} \sqrt{ \color{green}{c}} \\ \color{red}{42} &= 2 \times \color{blue}{3} \times \color{green}{7}=42\end{align}| |
Verify that the trinomial is indeed a perfect square trinomial. |
|
|\color{blue}{9}x^2 - \color{red}{42}x + \color{green}{49}| |
Factor according to the perfect square trinomial model. |
|
Since the area of a square is calculated using the formula |A=s^2,| we can deduce that |A=(\color{blue}{3x}-\color{green}{7})^2.| By association, we obtain |c=(\color{blue}{3x}-\color{green}{7})\ \text{m}.| |
|
See also
Function form (Standard form)
|y = ax + b| where | a = \dfrac{\Delta y}{\Delta x} = \dfrac{y_2 - y_1}{x_2 - x_1}|
General form
|0 = Ax + By + C| where |A, B, C \in \mathbb{Z}|
With the information provided in the graph below, determine the equation of the line in general form.
-
Find the slope given by |\dfrac{\Delta y}{\Delta x}.| ||a = \dfrac{2 - 4{.}4}{-0{.}5 - 0{.}5} = 2{.}4||
-
Find the initial value |(b)| by substituting in one of the points of the graph. ||\begin{align} f(x) &= 2{.}4 x + b\\ 2 &= 2{.}4 (-0{.}5) + b \\ b &= 3{.}2 \end{align}||
-
Transform the value of parameters |a| and |b| in function form to their simplified fractional form. ||\begin{align} y &= 2{,}4x + 3{,}2 \\ y &= \dfrac{24}{10}x + \dfrac{32}{10} \\ y &= \dfrac{12}{5}x + \dfrac{16}{5} \end{align}||
-
Find a common denominator for all the terms in the equation. ||\begin{align} y &= \dfrac{12}{5}x + \dfrac{16}{5} \\ \Rightarrow\ \frac{5y}{5} &= \dfrac{12x}{5} + \dfrac{16}{5} \end{align}||
-
Set the equation equal to |0.| ||\begin{align} \frac{5y}{\cancel{5}} &= \frac{12x}{\cancel{5}} + \frac{16}{\cancel{5}} \\ \Rightarrow 5y &= 12x + 16 \\ \Rightarrow\, \ 0 &= 12x - 5y + 16 \end{align}||
Answer: The equation of the line in general form is |0=12x-5y+16.|
See also
|y = a(bx)^2|
where |b=1| unless working with a specific context.
Determine the parabola’s equation with the information provided in the table below.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align}f(x)&=ax^2\\-6.75&=a(-1.5)^2\\-3&=a\end{align}| |
Substitute the |x| and |y| values with the coordinates of a point on the curve to find the value of |a.| |
|
The parabola’s equation is |f(x)=-3x^2.| |
|
For second-degree polynomial functions: |a \ne \dfrac{\Delta y}{\Delta x}|
See also
|f(x) = a \sqrt{bx}|
where |b=1| if the function is oriented to the right and |b=-1| if the function is oriented to the left.
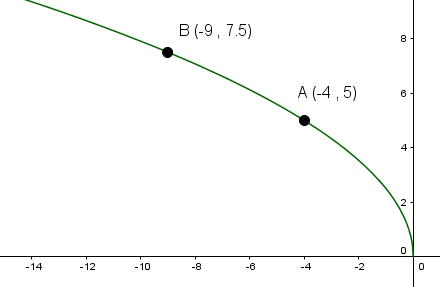
Find the equation of the following square root function:
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|b=-1,| since the function is oriented to the left. |
Determine the value of |b| based on the graph’s orientation. |
|
|\begin{align}f(x)&=a\sqrt{-x}\\5&=a\sqrt{-(-4)}\\5&=a(2)\\\dfrac{5}{2}&=a\end{align}| |
Substitute |(x,y)| in the equation with the coordinates of a point on the graph to find the value of |a.| |
|
Thus, the rule associated with the curve is |f(x)=\dfrac{5}{2}\sqrt{-x}.| |
|
See also
|y = a(c)^{bx}|
where
|a:| Initial value
|c:| Base (multiplying factor)
|b:| Calculation frequency
In 2005, a pond had 500 toads. Its toad population decreases by 5% every three years. If this pace continues, in what year will it have approximately 368 toads?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|x:| Number of years since 2005 |f(x):| Number of toads |
Define the variables. |
|
|\begin{align} f(x) &= a (c)^{bx} \\ f(x) &= 500 (c)^{bx} \end{align}| |
Replace |a| by the initial value, i.e. the number of toads in 2005. |
|
|\begin{align}f(x)&=500(c)^{x}\\f(x)&=500(0.95)^{x}\end{align}| |
Replace |c| with |0.95,| since |c=1-5\ \text{%}=1- 0.05=0.95.| Replace |b| with |\dfrac{1}{3}|since the |5\ \text{%}| decrease occurs every |3| years. |
|
|\begin{align} f(18) &= 500 (0{.}95)^{\frac{18}{3}} \\ f(18) &\approx 368 \end{align}| |
By replacing |x| with different values and using trial-and-error, we deduce that |x=18.| |
|
If |x=18,| this implies that in |18| years there will be |368| toads. Since the study took place in 2005, we can deduce that there will be approximately |368| toads in 2023 |(2005 + 18).| |
|
See also
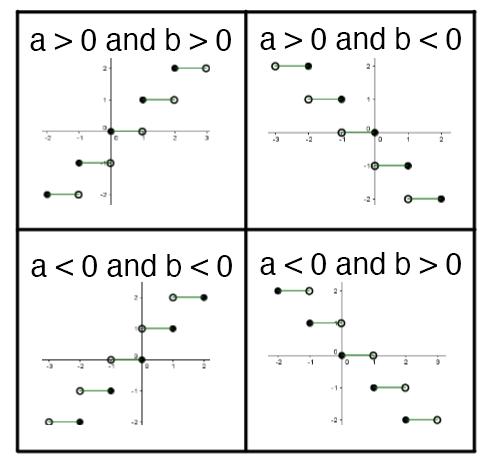
|f(x) = a \left[ b x\right]|
where
|{\mid}a{\mid} = | Vertical distance or height between two steps
|\dfrac{1}{{\mid}b{\mid}} = | Length of a step
To determine the sign of both |a| and |b,| refer to the direction of the open and closed points, and the variation (increasing or decreasing) of the graph:
A grocery store offers a new reward programme with stamps that provide significant discounts on selected items. To determine the number of stamps given to each customer, the grocery store uses the following graph:
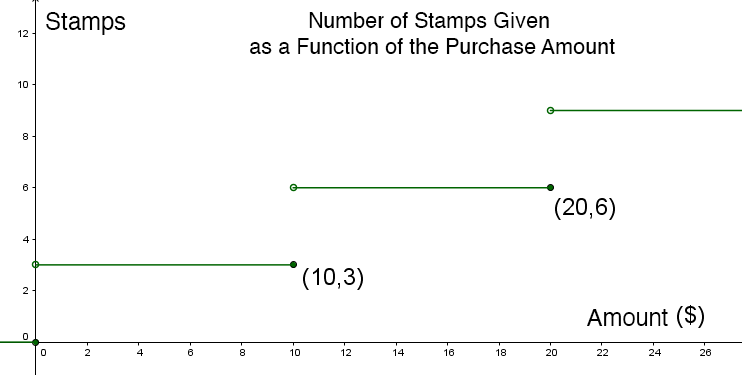
Using this graph, determine the possible purchase amounts if a customer receives |48| stamps.
-
Find the rule of the form |y=\color{red}{a}\left[\color{blue}{b}x\right]| ||\begin{align}\vert\color{red}{a}\vert&=6-3=\color{red}{3}\\ \vert\color{blue}{b}\vert&=\dfrac{1}{\text{Length of the step}}=\dfrac{1}{10}\end{align}||Based on the orientation of the graph and the order of the open and closed points, we deduce that: ||y = \color{red}{-3} \left[ \color{blue}{-\frac{1}{10}}x\right]||
-
Find the value of |x| when |y| is |48| ||\begin{gather}\begin{aligned}y&=\color{red}{-3}\left[\color{blue}{-\dfrac{1}{10}}x\right]\\48&=\color{red}{-3}\left[\color{blue}{-\dfrac{1}{10}}x\right]\\-16&=\left[\color{blue}{-\dfrac{1}{10}}x\right]\end{aligned}\\\\\begin{aligned}-16<\color{blue}{-\dfrac{1}{10}}x\quad&\text{or}\quad-15\geq\color{blue}{-\dfrac{1}{10}}x\\160>x\quad&\text{or}\quad150\leq x\end{aligned}\end{gather}||
-
Determine the |x| interval of the solution||x \in \left[150, 160\right[||
Answer : The purchase amount is at least |$150,| but less than |$160.|
See also
In a periodic function, a cycle is a repeating pattern, while the period is the length of one cycle along the |x|-axis.
Marie-Claude decides to get back in shape after a vacation by cycling with her group of friends. A coach guides them and decides what speed to maintain. To prepare the group for the next session, the coach gives them the following graph:
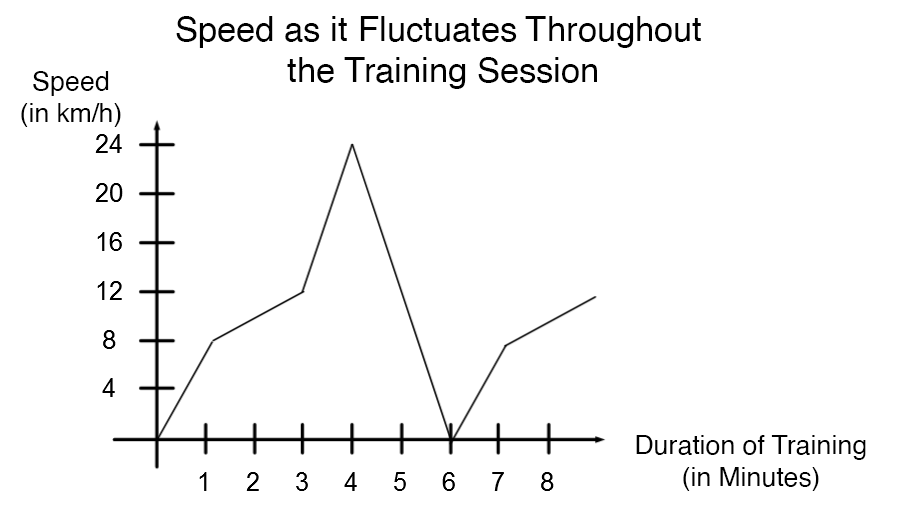
The training consists of repeating the same trip for |45| minutes. How many minutes, in total, will Marie-Claude have pedalled at a minimum speed of |16| km/h?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
| |
Identify the places where the speed is |16| km/h on the first cycle. |
| |
Identify points on each of the lines where the desired minimum speed is located. |
|
|a = \dfrac{\Delta y}{\Delta x} = \dfrac{24-12}{4-3} = 12| Thereby, |y = 12x + b| By the substitution of x and y, Thus, |\color{green}{y=12x - 24}| |
Find the equation of |\color{green}{\text{the green line}}.| To do so, use the points |(3,12)| and |(4,24),| since they are on |\color{green}{\text{the green line}}.| |
|
|\begin{align}16&=12x-24\\40&=12x\\\color{green}{3.33}&\approx x\end{align}| |
Replace |y| with |16| to find the time in minutes of the |\color{green}{\text{green point}}.| |
|
|a = \dfrac{\Delta y}{\Delta x} = \dfrac{24-0}{4-6} = -12| Thereby, |y = -12x + b| By the substitution of x and y, Thus, |\color{blue}{y=-12x + 72}| |
Find the equation of |\color{blue}{\text{the blue line}}.| To do so, use the points |(4,24)| and |(6,0)| since they are on |\color{blue}{\text{the blue line}}.| |
|
|\begin{align}16&=-12x+72\\-56&=-12x\\\color{blue}{4.67}&\approx x\end{align}| |
Replace the |y| by |16| to find the time in minutes of the |\color{blue}{\text{blue line}}.| |
|
|\color{blue}{4.67}-\color{green}{3.33}=\color{red}{1.34\ \text{min}}| |
Determine the duration between |\color{green}{\text{green point}}| and |\color{blue}{\text{blue point}}.| |
|
|6 - 0 = 6 \ \text{min}| |
Determine the period (length) of the repeated cycle. |
|
|\begin{align}\text{No. of cycles}&=\dfrac{\text{Total trip duration}}{\text{Duration of One Cycle}}\\\text{No. de cycles}&=\dfrac{45}{6}\\\text{No. de cycles}&=7.5\end{align}| |
Determine the number of completed cycles in the route. |
|
|7.5| equals |7| complete cycles |(7\times6=42\ \text{min})| and half a cycle.  Thus, the interval of |1.34| minute repeats exactly seven times. |
Analyze the incomplete cycle to determine if the portion meets the requirements of the initial question (minimum speed of |16| km/h). |
|
|\begin{align}\text{Total duration}&=\color{red}{\text{Duration of One Cycle}}\times\text{No. of periods}\\\text{Total duration}&=\color{red}{1.34}\times7\\\text{Total duration}&=9.38\end{align}| |
Determine the total duration of the analyzed interval. |
|
At the end of her training, Marie-Claude will have spent a total of |9.38| minutes pedalling at a speed of at least |16| km/h. |
|
See also
The inverse of a function |f(x),| denoted |f^{-1}(x),| is obtained by inverting the coordinates of the points such that |(x,y)\rightarrow(y,x).|
Sketch the graph of the inverse of the following function:
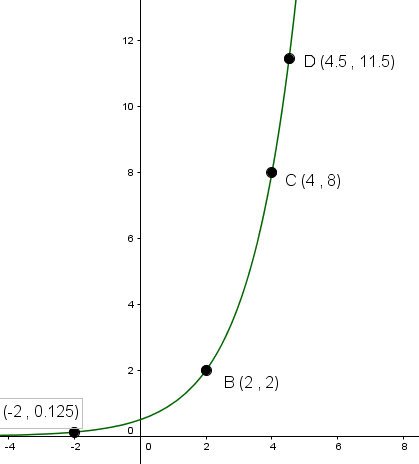
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
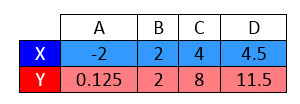 |
Create a table of values based on the given points. |
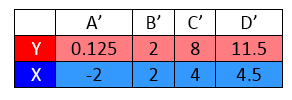 |
Invert the coordinates |(\color{blue}{x}, \color{red}{y}) \rightarrow (\color{red}{y}, \color{blue}{x}).| |
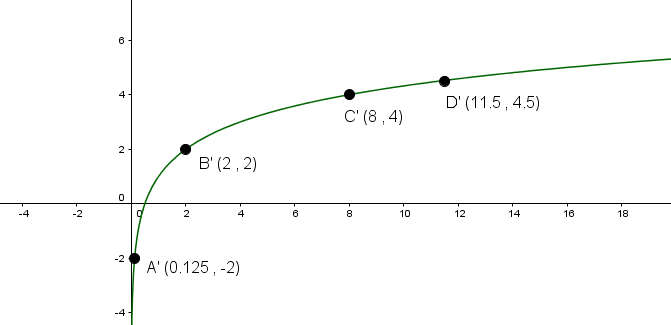 |
Sketch the graph of the inverse using this new table of values. |
The inverse of a function is not always a function itself. In the cases studied here, these functions are inverses of each other:
-
Exponential and logarithmic functions
-
A linear function with respect to itself
-
A second-degree function (with a restricted domain) and a square root function
See also
The same properties must always be analyzed when studying a function:
-
Domain: all the possible values of |x|
-
Range: all the possible values of |y|
-
X-intercept: the value of |x| when |y=0|
-
Y-intercept: the value of |y| when |x=0|
-
Maximum: the greatest value of |y|
-
Minimum: the smallest value of |y|
-
Increasing: when the graph goes "upwards" or is constant
-
Decreasing: when the graph goes "downwards" or is constant
-
Sign:
-
Positive sign: the portion of the graph that is above or equal to the |x|-axis
-
Negative sign: the portion of the graph that is below or equal to the |x|-axis
-
As an accountant of a large company, you must give a detailed account of the trends in profits over the past year. To help, here is the graph of the last 12 months.
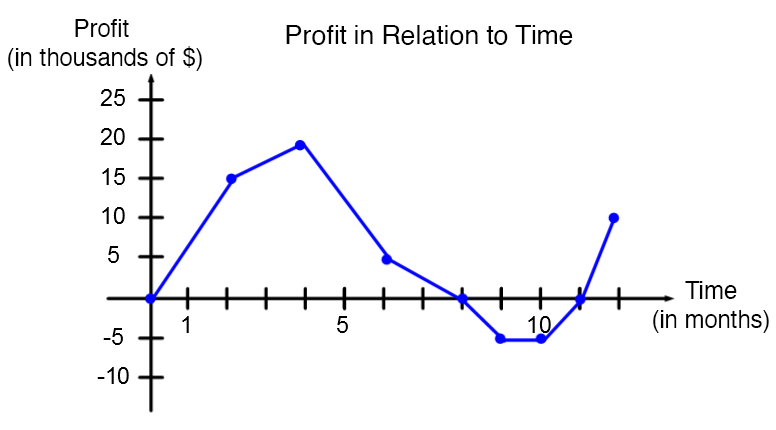
In order to properly support your argument, you must study this graph closely before preparing your presentation speech.
|
PROPERTIES |
EXPLANATIONS |
|---|---|
|
Domain: |[0, 12]| |
The smallest value on the |x|-axis is |0| and the biggest is |12.| |
|
Range: |[-5, 20]| |
The smallest value on the |y|-axis is |-5| and the biggest is |20.| |
|
Increasing: |[0, 4] \cup [9, 12]| |
By analyzing the |x| values, these are the two portions of the graph that go up or are constant. |
|
Decreasing: |[4, 10]| |
By analyzing the |x| values, it is the only portion of the graph that goes down or that is constant. |
|
Maximum: |\left\{20 \right\}| |
This is the largest |y| value reached by the graph. |
|
Minimum: |\left\{-5\right\}| |
This is the smallest |y| value reached by the graph. |
|
X-intercepts (Zeros): |(0,0) , (8,0) , (11,0)| |
These are the coordinates of the points where the graph touches the |x|-axis. |
|
Y-intercept: |(0,0)| |
These are the coordinates of the point where the graph touches the |y|-axis. |
|
Positive: |[0,8]\cup[11,12]| |
Among |x| values, these portions of the graph are above or equal to the |x|-axis. |
| Negative: |\{0\}\cup [8,11]| | Among the |x| values, these portions of the graph are below or equal to the |x|-axis. |
See also
Follow these steps to solve a system of equations using comparison:
-
Identify the variables associated with the unknowns.
-
Create the equations according to the scenario.
-
Isolate the same variable for each of the equations.
-
Compare the two equations to form a new one.
-
Solve this new equation.
-
Substitute the value of the found variable into one of the starting equations to find the value of the other variable.
At a corner store, a group of workers buys 4 coffees and 6 muffins for |$15.06.| The next day, they buy |3| coffees and |5| muffins for a total of |$11.97.| If the following day they want to buy |6| coffees and |4| muffins, how much will it cost?
-
Identify the variables associated with the unknowns
|x=| cost of a coffee (\$)
|y=| cost of a muffin (\$) -
Create the equations according to the scenario||\color{blue}{4x+6y=15.06}\\\color{red}{3x+5y=11.97}||
-
Isolate the same variable for each of the equations ||\begin{align}y&=\color{blue}{2.51-\dfrac{4}{6}x}\\y&=\color{red}{2.394-\dfrac{3}{5}x}\end{align}||
-
Compare the two equations to form a new one ||\color{blue}{2.51-\dfrac{4}{6}x}=\color{red}{2.394 -\dfrac{3}{5}x}||
-
Solve this new equation ||\begin{align}\color{blue}{2.51-\dfrac{4}{6}x}&=\color{red}{2.394-\dfrac{3}{5}x}\\2.51-2.394&=-\dfrac{3}{5}x+\dfrac{4}{6}x\\0.116&=\dfrac{1}{15}x\\1.74&=x\end{align}||
-
Substitute the value of the found variable into one of the starting equations to find the value of the other variable ||\begin{align}4x+6y&=15.06\\4(1.74)+6y&=15.06\\y&=1.35\end{align}||
-
Calculate the cost of |6| coffees and |4| muffins ||\begin{align}6\ \text{coffees}+4\ \text{muffins}&=\ ?\\6x+4y&=\ ?\\6(1.74)+4(1.35)&=\ ?\\15.84&=\ ?\end{align}||
Answer: It will cost |$15.84.|
See also
Follow these steps to solve a system of equations using substitution:
-
Identify the variables associated with the unknowns.
-
Create the equations according to the scenario.
-
Isolate a variable in one of the two equations.
-
Substitute this same variable in the other equation by the algebraic expression associated with it.
-
Solve this new equation.
-
Substitute the value of the found variable into one of the starting equations to find the value of the other variable.
At a corner store, a group of workers buys |4| coffees and |6| muffins for |$15.06.| The next day, they buy |3| coffees and |5| muffins for a total of |$11.97.| If the following day they buy |6| coffees and |4| muffins, how much will it cost?
-
Identify the variables associated with the unknowns
|x=| cost of a coffee (\$)
|y=| cost of a muffin (\$) -
Create the equations according to the scenario||\color{blue}{4x+6y=15.06}\\\color{red}{3x+5y=11.97}||
-
Isolate a variable in one of the two equations ||\begin{align}\color{blue}{4x+6y}&=\color{blue}{15.06}\\\color{blue}{y}&=\color{blue}{2.51-\dfrac{4}{6}x}\end{align}||
-
Substitute this same variable in the other equation by the algebraic expression associated with it ||\begin{align}\color{red}{3x+5}\color{blue}{y}&=\color{red}{11.97}\\\color{red}{3x+5}\color{blue}{\left(2.51-\dfrac{4}{6}x\right)}&=\color{red}{11.97}\end{align}||
-
Solve this new equation ||\begin{align}\color{red}{3x+5}\color{blue}{\left(2.51-\dfrac{4}{6}x\right)}&=\color{red}{11.97}\\3x+12.55-\dfrac{20}{6}x&=11.97\\x&=1.74\end{align}||
-
Substitute the value of the found variable into one of the starting equations to find the value of the other variable
||\begin{align}4x+6y&=15.06\\4(1.74)+6y&=15.06\\ y&=1.35\end{align}|| -
Calculate the cost of |6| coffees and |4| muffins ||\begin{align}6\ \text{coffees}+4\ \text{muffins}&=\ ?\\6x+4y&=\ ?\\6(1.74)+4(1.35)&=\ ?\\15.84&=\ ?\end{align}||
Answer: It will cost |$15.84.|
See also
Follow these steps to solve an equation system using the elimination method:
-
Identify the variables associated with the unknowns.
-
Create the equations according to the scenario.
-
Find equivalent equations to obtain the same coefficient for the same variable.
-
Subtract the two equations.
-
Isolate the remaining variable to find its value.
-
Substitute the value of the found variable in one of the starting equations to find the value of the other variable.
At a corner store, a group of workers buys 4 coffees and 6 muffins for |$15{.}06.| The next day, they buy 3 coffees and 5 muffins for a total of |$11{.}97.| If the following day they buy 6 coffees and 4 muffins, how much will it cost?
-
Identify the variables associated with the unknowns
|x=| cost of a coffee (\$)
|y=| cost of a muffin (\$) -
Create the equations according to the scenario||\color{blue}{4x+6y=15.06}\\\color{red}{3x+5y=11.97}||
-
Find equivalent equations to obtain the same coefficient for the same variable ||\begin{align}3(\color{blue}{4x+6y}=\color{blue}{15.06})&\Rightarrow\color{blue}{12x+18y=45.18}\\4(\color{red}{3x+5y}=\color{red}{11.97})&\Rightarrow\color{red}{12x+20y=47.88}\end{align}||
-
Subtract the two equations ||\begin{align}\color{blue}{12x+18y}&=\color{blue}{45.18}\\-(\color{red}{12x+20y}&=\color{blue}{47.88}\big)\\\hline-2y&=-2.70\end{align}||
-
Isolate the remaining variable to find its value ||\begin{align}-2y&=-2.70\\ y&=1.35\end{align}||
-
Substitute the value of the found variable into one of the starting equations to find the value of the other variable ||\begin{align}4x+6y&=15.06\\4x+6(1.35)&=15.06\\ x&=1.74\end{align}||
-
Calculate the cost of |6| coffees and |4| muffins ||\begin{align}6\ \text{coffees}+4\ \text{muffins}&=\ ?\\6x+4y&=\ ?\\6(1.74)+4(1.35)&=\ ?\\15.84&=\ ?\end{align}||
Answer: It will cost |$15.84.|
See also
In general, the law of multiplying radicals is used to carry out the factorization |(\sqrt{ab}=\sqrt{a}\sqrt{b}).| To do so:
-
Decompose the radicand into a product of factors, where one factor is a square number.
-
Transform the root of a product into a product of roots |(\sqrt{ab}=\sqrt{a}\sqrt{b}).|
-
Calculate the root of the square number.
What is the simplified value of the following radical: ||\sqrt{45}||
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\sqrt{45} = \sqrt{\color{blue}{9} \times 5}| |
Factor the radicand into a product of factors where one factor is a square number. |
|
|\begin{align} &\sqrt{\color{blue}{9} \times 5} \\=\ &\sqrt{\color{blue}{9}} \sqrt {5} \end{align}| |
Use the law of the product of radicals: |\sqrt{ab}=\sqrt{a}\sqrt{b}.| |
|
|\begin{align} \sqrt{\color{blue}{9}} \sqrt {5} \\ =\ \color{blue}{3} \sqrt {5} \end{align}| |
Calculate the square root of the square number. |
|
Therefore, |\sqrt{45} = 3 \sqrt{5}.| |
|
See also
We can deduce three theorems from the following right triangle.
-
In a right triangle, the measurement of each side of the right angle is the geometric mean between its projection onto the hypotenuse and the hypotenuse itself.||\begin{align} \dfrac{m}{a} = \dfrac{a}{c}\ &\Leftrightarrow\ a^2 = m c \\\\ \dfrac{n}{b} = \dfrac{b}{c}\ &\Leftrightarrow\ b^2 = n c \end{align}||
-
In a right triangle, the height measurement (altitude) is the geometric mean of the projections of the sides onto the hypotenuse. ||\dfrac{m}{h} = \dfrac{h}{n}\ \Leftrightarrow\ h^2 = m n||
-
In a right triangle, the product of the measures of the hypotenuse and the height (altitude) equals the product of the measurements of the sides of the right angle. ||c h = a b||
To stand out from other contractors, a construction company suggests houses with roofs of different shapes. Among these choices, we have the following form:
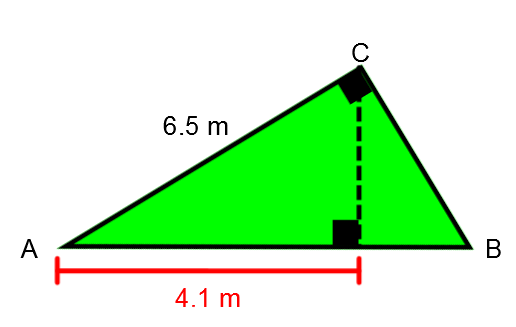
The company manager needs the two missing outer measurements of triangle |(\overline{AB},\overline{BC})| to estimate the production costs. Help him find them.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align}a&=6.5\\ m&=4.1\\ c\ (m\overline{AB})&=\ ?\\ b\ (m\overline{BC})&=\ ?\end{align}| |
Associate all known and desired measurements with one of the measurements in the reference drawing. |
|
|\begin{align}a^2&=mc\\6.5^2&=4.1c\end{align}| |
Choose the theorem where there will be only one unknown: In a right triangle, the measurement of either side of the right angle is the geometric mean between its projection on the hypotenuse and the hypotenuse itself. |
|
|\begin{align}6.5^2&=4.1c\\42.25&=4.1c\\\dfrac{42.25}{\color{red}{4.1}}&=\dfrac{4.1c}{\color{red}{4.1}}\\10.3&\approx c\end{align}| |
Solve the equation. |
|
|\begin{align}a^2+b^2&=c^2\\6.5^2+m\overline{BC}^2&=10.3^2\\42.25+m\overline{BC}^2&\approx106.09\\ m\overline{BC}^2&\approx63{.}84\\ m\overline{BC}&\approx8\end{align}| |
Apply the Pythagorean theorem on the large green right triangle to find the measurement of the missing leg. |
|
Thus, |m\overline{AB}\approx10.3\ \text{m}| and |m\overline{BC}\approx8\ \text{m}.| |
|
See also
Considering the angle |\theta| as a reference, we have:
|\sin \theta = \dfrac{\text{Measurement of side opposite to}\ \theta}{\text{Measurement of the hypotenuse}}|
|\cos \theta = \dfrac{\text{Measurement of the side adjacent to}\ \theta}{\text{Measurement of the hypotenuse}}|
|\tan \theta = \dfrac{\text{Measurement of the side opposite to} \ \theta}{\text{Measurement of side adjacent to} \ \theta}|
The elevation angle of a house's roof trusses must be a minimum of |25^\circ| for building standards to be met. To ensure that this constraint is respected, a manufacturer decides to establish this angle at |35^\circ.| If the length of the roof truss is |13| metres, what will be the measurements of the other two sides?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\sin \color{red}{35^\circ} = \dfrac{\color{red}{c}}{\color{green}{13}}| |
Identify the correct trigonometric ratio: |\sin \theta = \dfrac{\text{Opposite}}{\text{Hypotenuse}}| |
|
|\begin{align} \sin \color{red}{35^\circ} &= \dfrac{\color{red}{c}}{\color{green}{13}} \\ \color{green}{13}\sin \color{red}{35^\circ} &= \color{red}{c} \\ 7{.}46 &\approx \color{red}{c} \end{align}| |
Solve the equation. |
|
|\cos \color{red}{35^\circ} = \dfrac{\color{blue}{b}}{\color{green}{13}}| |
Identify the correct trigonometric ratio: |\cos\theta=\dfrac{\text{Adjacent}}{\text{Hypotenuse}}| |
|
|\begin{align} \cos \color{red}{35^\circ} &= \dfrac{\color{blue}{b}}{\color{green}{13}} \\ \color{green}{13} \cos \color{red}{35^\circ} &= \color{blue}{b} \\ 10{.}65 &\approx \color{blue}{b} \end{align}| |
Solve the equation. |
|
Thus, |\color{blue}{m\overline{AC}}\approx10.65\ \text{m}| and |\color{red}{m\overline{AB}}\approx7.46\ \text{m}.| |
|
To determine the route a helicopter should take to pick up people in the woods who need help, a map of the region has been triangulated with the helicopter’s current location, the hospital and the people who are in distress.
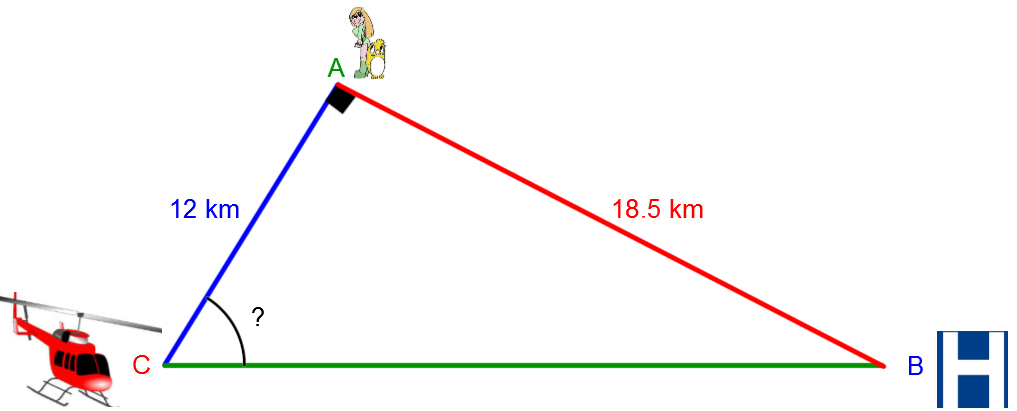
According to this drawing, what angle of orientation should the helicopter take to reach the people as quickly as possible?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\tan\ ?=\dfrac{\color{red}{18.5}}{\color{blue}{12}}| |
Identify the correct trigonometric ratio: |\tan\theta=\dfrac{\text{Opposite}}{\text{Adjacent}}| |
|
|\begin{align}\tan\ ?&=\dfrac{\color{red}{18.5}}{\color{blue}{12}}\\ \tan\ ?&\approx1.54\\ ?&=\tan^{-1}(1.54)\\ ?&\approx57^\circ\end{align}| |
Solve the equation. |
|
The helicopter's angle of orientation should be |57^\circ.| |
|
See also
To find this, you have to add lines (usually a height) with particular properties and unknown measures.
-
Choose the correct vertex from which to draw a height.
-
Use the trigonometric ratios in the right triangle to find the missing measurements.
-
Apply the formula for the area of a triangle with the found measurements.
Find the area of the following triangle:
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
| |
Find all angle measurements, if possible (sum of interior angles of a triangle |=180^\circ|). |
| |
Sketch a height to make it the unknown in the trigonometric ratio used. |
| |
Identify the right triangle formed. |
|
|\begin{align}\sin\color{red}{24^\circ}&=\dfrac{\color{blue}{h}}{42}\\ 17.08&\approx\color{blue}{h}\\\\ \cos\color{red}{24^\circ}&=\dfrac{\color{green}{b}}{42}\\ 38.37&\approx\color{green}{b}\end{align}| |
Find as many measurements as possible using trigonometric ratios. |
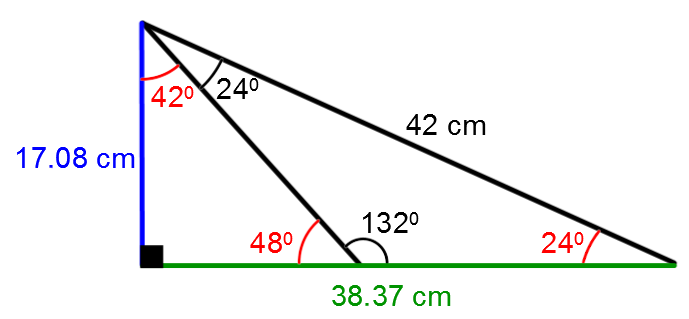 |
Find the missing angle measurements of the small triangle on the left. |
|
|\begin{align}\tan\color{red}{48^\circ}&=\dfrac{\color{blue}{17.08}}{\color{green}{2^\text{nd}\text{ leg}}}\\ 15.38&\approx\color{green}{2^\text{nd}\text{ leg}}\end{align}| |
Find the measurement of the 2nd leg of the small triangle. |
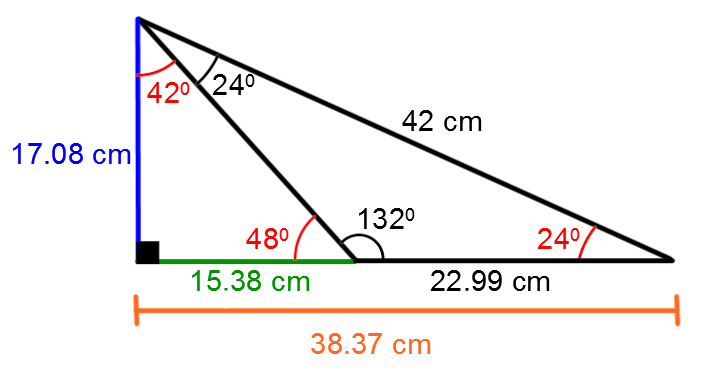 |
Find the measurement of the base of the initial triangle. |
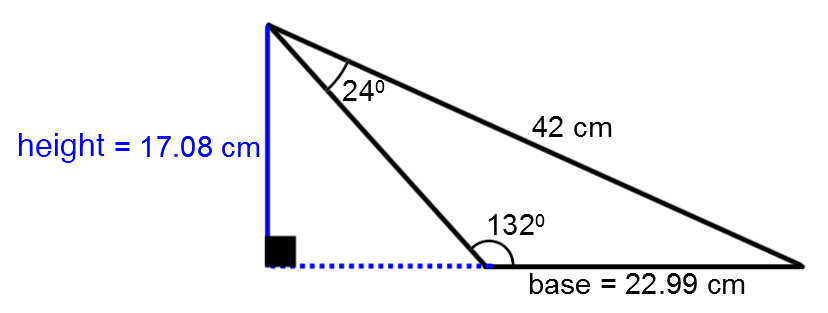 |
Identify the base and height and use them to calculate the area of the triangle. |
|
|\begin{align}A&=\dfrac{b\times\color{blue}{h}}{2}\\ A&=\dfrac{22.99\times\color{blue}{17.08}}{2}\\ &\approx196.33\ \text{cm}^2\end{align}| |
Calculate the area of the triangle according to the formula. |
|
The area of the triangle is approximately |196{.}33 \ \text{cm}^2.| |
|
See also
-
A - S - A: Two triangles are congruent (isometric) when a pair of corresponding congruent sides is located between two pairs of corresponding congruent angles.
-
S - A - S: Two triangles are congruent (isometric) when a pair of corresponding congruent angles is located between two pairs of corresponding congruent sides.
-
S - S - S: Two triangles are congruent (isometric) when all of the pairs of corresponding sides are congruent.
Due to machinery problems at a construction company, employees have to assemble the triangular-shaped roof trusses themselves to complete the construction on a house. All of the roof trusses must be identical.
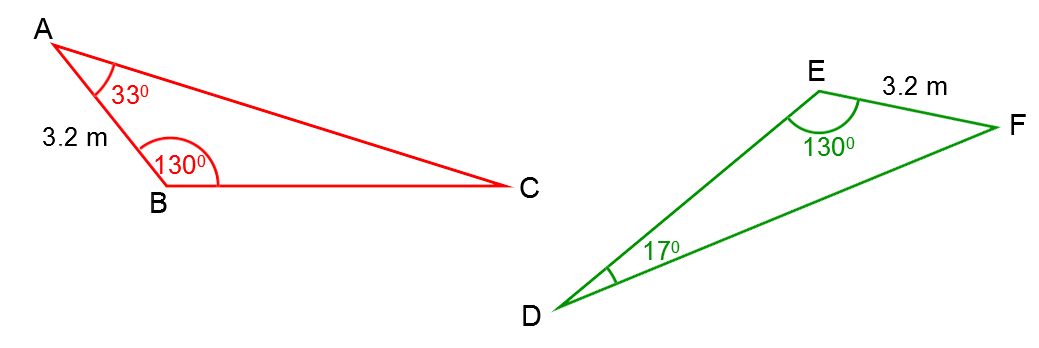
Using the information provided above, demonstrate that these two constructions are congruent (isometric).
|
CONFIRMATIONS |
EXPLANATIONS |
|---|---|
|
|\angle \color{red}{BAC} \cong \angle \color{green}{EFG}| |
|m \angle \color{green}{EFG} = 180^\circ - 130^\circ - 17^\circ = 33^\circ = m \angle \color{red}{BAC}| |
|
|\color{red}{\overline{AB}} \cong \color{green}{\overline{EF}}| |
By hypothesis |
|
|\angle \color{red}{ABC} \cong \angle \color{green}{DEF}| |
By hypothesis |
|
The |\Delta\color{red}{ABC}\cong\Delta\color{green}{DEF}| by A-S-A Theorem for Congruence. |
|
See also
-
A - A: Two triangles are similar when two pairs of corresponding angles are congruent.
-
S - A - S: Two triangles are similar when a pair of congruent corresponding angles is located between two pairs of proportional corresponding sides.
-
S - S - S: Two triangles are similar if all three pairs of corresponding sides are proportional.
The city is organizing a family run for a community fundraiser. They want the path taken by adults to be similar to that taken by the children.
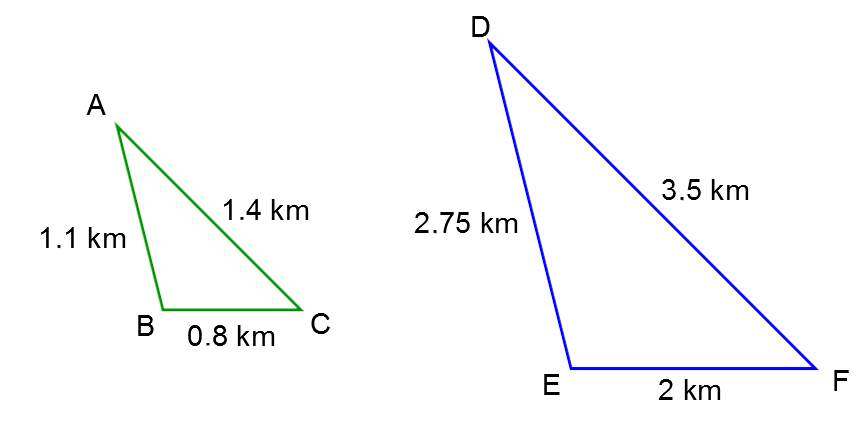
Considering the information given above, demonstrate that the two paths are similar.
|
CONFIRMATIONS |
EXPLANATIONS |
|---|---|
|
|\dfrac{m\color{green}{\overline{AC}}}{m \color{blue}{\overline {DF}}} = \dfrac{m\color{green}{\overline{AB}}}{m \color{blue}{\overline {DE}}}= \dfrac{m\color{green}{\overline{BC}}}{m \color{blue}{\overline {EF}}}| |
|\begin{gather}\dfrac{\color{green}{1.4}}{\color{blue}{3.5}}=\dfrac{\color{green}{1.1}}{\color{blue}{2.75}}=\dfrac{\color{green}{0.8}}{\color{blue}{2}}\\ \dfrac{2}{5}=\dfrac{2}{5}=\dfrac{2}{5}\end{gather}| |
|
Thus, |\Delta\color{green}{ABC}\sim\Delta\color{blue}{DEF}| by the S-S-S Theorem of Similarity. |
|
See also
|\text{Distance} = \sqrt{(y_2 - y_1)^2 + (x_2 - x_1)^2}|
where
|(x_1, y_1):| Coordinates of the starting point of the segment
|(x_2, y_2):| Coordinates of the ending point of the segment
To determine how much gasoline an airplane must have to complete a Montreal-Paris flight, both cities are represented on a Cartesian plane graduated in kilometres.
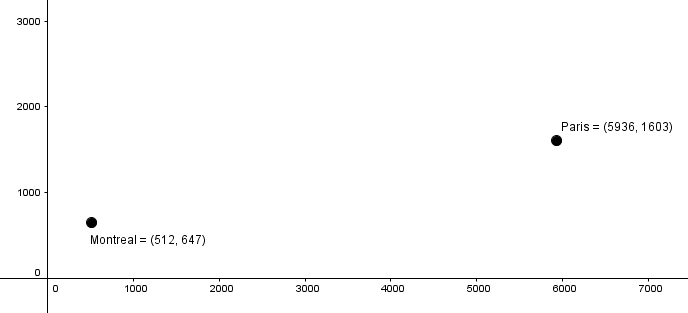
What is the distance in kilometres between these two cities?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align}\text{Montreal}&=(\color{blue}{x_1},\color{red}{y_1})=(\color{blue}{512},\color{red}{647})\\ \text{Paris}&=(\color{green}{x_2},y_2)=(\color{green}{5936},1603)\end{align}| |
Identify the points |(x_1,y_1)| and |(x_2,y_2).| |
|
|\begin{align}\text{Distance}&=\sqrt{(y_2-\color{red}{y_1})^2+(\color{green}{x_2}-\color{blue}{x_1})^2}\\ \text{Distance}&=\sqrt{(1\ 603-\color{red}{647})^2+(\color{green}{5\ 936}-\color{blue}{512})^2}\end{align}| |
Substitute the values into the formula. |
|
|\begin{align}\text{Distance}&=\sqrt{(1\ 603-\color{red}{647})^2+(\color{green}{5\ 936}-\color{blue}{512})^2}\\ \text{Distance}&=\sqrt{956^2+5\ 424^2}\\ \text{Distance}&\approx5\ 507.6\ \text{km}\end{align}| |
Solve the equation. |
|
The distance between Montreal and Paris is approximately |5\ 507.6 \ \text{km}.| |
|
See also
Consider |(x,y),| the coordinates of the desired division point. ||x=x_1+ \dfrac{a}{b} (x_2-x_1)|| ||y=y_1+ \dfrac{a}{b} (y_2-y_1)||
where
|(x_1,y_1):| Starting point of the segment
|(x_2,y_2):| Endpoint of the segment
|\dfrac{a}{b}:| Fraction that defines the division of the segment (part to whole)
Every morning, you wait at the bus stop for the bus to take you to school. You noticed that for the stop to be on-route for the other students in the area, it divides the segment of the street from your house to the school into a ratio of |1:4.|
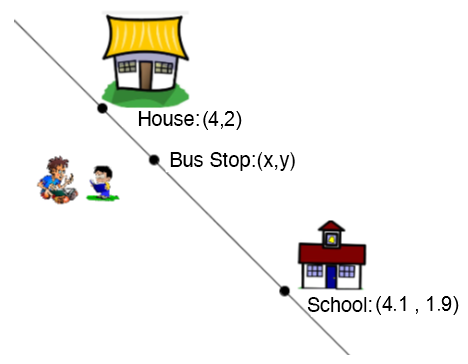
Using the information provided, determine the coordinates of your bus stop’s location.
-
Identify the starting point and the ending point. ||\begin{align} \text{House} &=(\color{blue}{x_1},\color{red}{y_1})= (\color{blue}{4},\color{red}{2}) \\ \text{School} &=(\color{green}{x_2},y_2)= (\color{green}{4{.}1},1{.}9) \end{align}||
-
Find the fraction |\dfrac{a}{b}| associated with the ratio. ||1:4=\dfrac{1}{1+4}=\dfrac{1}{5}||
-
Substitute the values into the formula and solve the equation to find the |x|-coordinate of the division point. ||\begin{align}x&=\color{blue}{x_1}+\dfrac{a}{b}(\color{green}{x_2}-\color{blue}{x_1})\\ x&=\color{blue}{4}+\dfrac{1}{5}(\color{green}{4.1}-\color{blue}{4})\\ x&=4.02\end{align}||
-
Substitute the values in the formula and solve the equation to find the |y|- coordinate of the division point. ||\begin{align}y&=\color{red}{y_1}+\dfrac{a}{b}(y_2-\color{red}{y_1})\\ y&=\color{red}{2}+\dfrac{1}{5}(1.9-\color{red}{2})\\ y&=1.98\end{align}||
Answer: The coordinates of the division point |(x,y)| are |(4.02,1.98).|
It is important to differentiate between the two types of notations used to illustrate the portion associated with a division point. This is done in order to then use the appropriate notation for the formula: ||\begin{matrix}\text{Ratio}\\ a:b\end{matrix}\Leftrightarrow\begin{matrix}\text{Fraction}\\ \dfrac{a}{a+b}\end{matrix}||
See also
The lines |y_1=a_1x+b_1| and |y_2=a_2x+b_2| are parallel if and only if |a_1=a_2.|
What is the equation of the line that is parallel to the one identified in the Cartesian plane below and passes through point C?
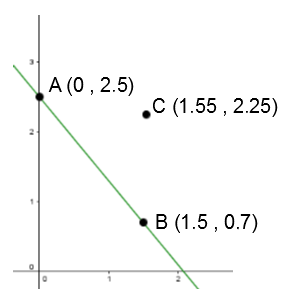
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|a=\dfrac{\Delta y}{\Delta x}=\dfrac{0.7-2.5}{1.5-0}=-1.2| |
Find the slope of |\overline{AB}.| |
|
|\begin{align}y&=-1.2x+b\\ 2.25&=-1.2(1.55)+b\\ 2.25&=-1.86+b\\ 4.11&=b\end{align}| |
Find the equation of the line passing through |C(1.55,2.25)| in function form |y=ax+b.| In this case, the |a| value of the desired equation is the same as that of |\overline{AB},| since the lines are parallel. |
|
Finally, the equation of the line which is parallel to |\overline{AB}| and passes through point |C(1.55,2.25)| is |y=-1.2x+4.11.| |
|
The lines |y_1=a_1x+b_1| and |y_2=a_2x+b_2| are perpendicular if and only if |a_1\times a_2=-1.|
In other words, two lines are perpendicular if the slope of one is the opposite of the reciprocal of the slope of the other: |a_2=\dfrac{-1}{a_1}.|
What is the equation of the line that is perpendicular to the one identified in the Cartesian plane below and passes through point |C|?
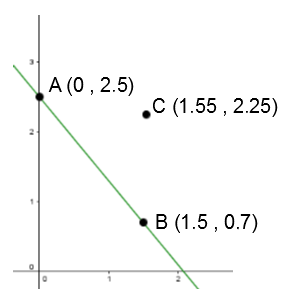
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|a_1=\dfrac{\Delta y}{\Delta x}=\dfrac{0.7-2.5}{1.5-0}=-1.2| |
Find the slope of |\overline{AB}.| |
|
|\begin{align}a_1\times a_2&=-1\\ -1.2\times a_2&=-1\\ a_2&=\dfrac{-1}{-1.2}\\ a_2&=0.8\overline{3}\end{align}| |
Find the value of |a_2| of the line passing through C using the fact that the product of the slopes of two perpendicular lines is equal to |-1.| |
|
|\begin{align}y&=a_2x+b\\ y&=0.8\overline{3}x+b\\ 2.25&=0.8\overline{3}(1.55)+b\\ 2.25&\approx1.29+b\\ 0.96&\approx b\end{align}| |
Find the rule of the line passing through |C(1.55,2.25)| in function form |y=a_2x+b.| |
|
Finally, the equation of the line that is perpendicular to |\overline{AB}| and passes through point |C(1.55,2.25)| is |y=0.8\overline{3}x+0.96.| |
|
See also
|
TYPES OF EVENTS |
DEFINITION |
EXAMPLE |
|---|---|---|
|
Mutually Exclusive |
When the events can't happen at the same time. |
Roll a six-sided die and get a result that is a multiple of both 3 and 4. |
|
Not Mutually Exclusive |
When the events can occur at the same time. |
Draw a random card from a deck of 52 cards and get one that is both red and an ace. |
|
Dependent |
When the occurrence of one affects the occurrence of the other. |
Draw two cards successively, without replacement, from a deck that contains 52 cards at the start. |
|
Independent |
When the occurrence of one does not affect the occurrence of the other. |
Choose a card from a deck that contains 52 cards and roll a six-sided die. |
Do not forget the types of events that have been seen in previous years (certain, probable, impossible, elementary, complementary, compatible, and incompatible).
See also
Consider |a,| the odds for and |b,| the odds against. Therefore:
A ratio of odds for |= a : b \Rightarrow \dfrac {a}{a+b}|
RA ratio of odds against |= b : a \Rightarrow \dfrac {b}{b+a}|
Thus, the net gain is obtained according to the following proportion: ||\dfrac{\text{Bet Amount}}{\text{Net Gain}}=\dfrac{\text{No. of chances bet on}}{\text{No. of total chances}}||
During the days of the Quebec racetrack, it was possible to bet on the victories of racehorses. Each horse had a rating which quantified its chances of winning. For the last race, a fan bet |$20| on the victory of a horse whose odds for winning were |1:14.| What was the potential payout (net gain) of his bet?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align}\dfrac{20}{\text{Net gain}}&=\dfrac{\color{blue}{1}}{\color{blue}{1}+\color{red}{14}}\\\\ \dfrac{20}{\text{Net gain}}&=\dfrac{\color{blue}{1}}{15}\end{align}| |
Apply the proportion. |
|
|\begin{align}\dfrac{20}{\text{Net gain}}&=\dfrac{\color{blue}{1}}{15}\\\\ \text{Net gain}&=\dfrac{20\times15}{\color{blue}{1}}\\ \text{Net gain}&=300\end{align}| |
Solve using cross multiplication. |
|
If his horse finished in first place in the race, the fan would win |$300.| |
|
For some boxing matches, the outcome of a match can be bet upon. Each fighter has a rating which quantifies their chances of winning. The champion has |44:1| chances of winning the next fight. If a fan bet |$10| against the champion's victory, what would be their net gain?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align}\text{Ratio of chances for}&=\color{blue}{44}:\color{red}{1}\\\\ \Rightarrow\text{Ratio of chances against}&=\color{red}{1}:\color{blue}{44}\end{align}| |
Identify the odds against ratio. |
|
|\begin{align}\dfrac{10}{\text{Net gain}}&=\dfrac{\color{red}{1}}{\color{red}{1}+\color{blue}{44}}\\\\ \dfrac{10}{\text{Net gain}}&=\dfrac{\color{red}{1}}{45}\end{align}| |
Apply the proportion. |
|
|\begin{align}\dfrac{10}{\text{Net gain}}&=\dfrac{\color{red}{1}}{45}\\\\ \text{Net gain}&=\dfrac{10\times45}{\color{red}{1}}\\ \text{Net gain}&=450\end{align}| |
Solve using cross multiplication. |
|
If the champion does not manage to keep his belt, the boxing fan will win |$450.| |
|
See also
|\mathbb{E} = (p_1 x_1 + p_2 x_2 + \dots + p_i x_i) - M|
where
|p_i =| Probability of occurrence of the event |i|
|x_i =| Amount associated with the event |i|
|M =| Initial bet amount
If |\mathbb{E}=0,| then the game is fair.
If |\mathbb{E}<0,| then the game is unfavourable to the player.
If |\mathbb{E}>0,| then the game is favourable to the player.
To finance the school's freestyle ski team, organizers set up a fundraising activity for which it is possible to win the following prizes:
-
A weekend family ski pass (value of |$800|)
-
Two alpine ski season tickets (value of |$500| each)
-
Four pairs of skis (value of |$300| each)
-
Eight lift tickets, valid for one day (value of |$45| each)
There are |336| tickets to sell. What should the selling price of a ticket be to ensure the raffle is fair?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align} \mathbb{E} &= (\color{blue}{p_1 x_1} + \color{red}{p_2 x_2} + \color{green}{p_3 x_3} + \color{black}{p_4 x_4}) - M \\\\ \mathbb{E} &= \left(\color{blue}{\dfrac{1}{336}\times 800 } + \color{red}{\dfrac{2}{336} \times 500} + \color{green}{\dfrac{4}{336} \times 300} + \color{black}{\dfrac{8}{336} \times 45}\right) - M \end{align}| |
Apply the mathematical expectation formula. |
|
|\begin{align} \mathbb{E} &= \left(\color{blue}{\dfrac{1}{336}\times 800 } + \color{red}{\dfrac{2}{336} \times 500} + \color{green}{\dfrac{4}{336} \times 300} + \color{black}{\dfrac{8}{336} \times 45}\right) - M \\\\ 0 &= \left(\color{blue}{\dfrac{1}{336}\times 800 } + \color{red}{\dfrac{2}{336} \times 500} + \color{green}{\dfrac{4}{336} \times 300} + \color{black}{\dfrac{8}{336} \times 45}\right) - M \end{align}| |
Replace the value of |\mathbb{E}| by |0,| since the game is fair. |
|
|\begin{align} 0 &= \left(\color{blue}{\dfrac{1}{336}\times 800 } + \color{red}{\dfrac{2}{336} \times 500} + \color{green}{\dfrac{4}{336} \times 300} + \color{black}{\dfrac{8}{336} \times 45}\right) - M \\ 0 &= \left(\color{blue}{\dfrac{800}{336}} + \color{red}{\dfrac{1000}{336}} + \color{green}{\dfrac{1200}{336}} + \color{black}{\dfrac{360}{336}}\right) - M \\ 0 &= \dfrac{3360}{336} - M \\ M &= \dfrac{3360}{336} \\ M &= 10\ $ \end{align}| |
Isolate |M| to find the value of the initial bet. |
|
For the raffle to be fair, tickets must be sold for |$10.| |
|
See also
|P(A \mid B) = \dfrac{P (A \cap B)}{P (B)}|
where |P(A) >0|
During the previous month, listeners of a Quebec radio channel had the chance to win a trip to Walt Disney World. Before randomly drawing for the winner, the broadcaster provided an overview of the participants:
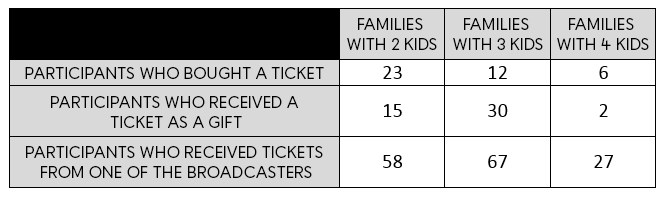
With the knowledge that he was given the raffle ticket as a gift, what is the probability that the winner will be the father of a family of three children?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|P(\color{red}{B}) = \dfrac{\color{red}{15 + 30 + 2}}{23 + 12 + \dots + 67 + 27} = \dfrac{\color{red}{47}}{240}| |
Identify the boxes referring to those who received a ticket as a gift. |
|
|P(\color{blue}{A \cap B}) = \dfrac{\color{blue}{30}}{240}| |
Of the people identified above, identify those with a family of three children. |
|
|\begin{align} P(\color{blue}{A} \mid \color{red}{B}) &= \dfrac {P( \color{blue}{A \cap B})}{P(\color{red}{B})} \\ P(\color{blue}{A} \mid \color{red}{B}) &= \dfrac{\frac{\color{blue}{30}}{240}}{\frac{\color{red}{47}}{240}} \\ P(\color{blue}{A} \mid \color{red}{B}) &= \dfrac{\color{blue}{30}}{\color{red}{47}} \end{align}| |
Apply the formula. |
|
Thus, the probability that the winner is a father of a family of three children, knowing that he was given the ticket as a gift, is |\dfrac{30}{47}.| |
|
See also
|EM = \dfrac{\sum \mid x_i - \overline {x} \mid}{n}|
where
|x_i| each data point
|\sum| the sum
|n| the total number of data
Over the last month, 11 houses in the same neighbourhood were sold for the following prices:
|\color{blue}{$\ 156\ 700},\color{red}{$\ 158\ 900},$\ 159\ 000,$\ 162\ 500,$\ 164\ 100,$\ 167\ 400,$\ 172\ 000,$\ 175\ 000,$\ 178\ 100,$\ 179\ 000,$\ 183\ 000.|
For statistical purposes, calculate the mean deviation of this distribution.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\dfrac{156\ 700+158\ 900+\dots+179\ 000+183\ 000}{11}=$\color{green}{168\ 700}| |
Calculate the mean given by n|\dfrac{\sum x_i}{n}.| |
|
|\begin{align} \mid \color{blue}{156\ 700} - \color{green}{168\ 700}{\mid} &= 12\ 000 \\ \mid \color{red}{158\ 900} - \color{green}{168\ 700}{\mid} &= 9\ 800 \\ \mid 159\ 000 - \color{green}{168\ 700}{\mid} &= 9\ 700 \\ \mid 162\ 500 - \color{green}{168\ 700}{\mid} &= 6\ 200 \\ \mid 164\ 100 - \color{green}{168\ 700}{\mid} &= 4\ 600 \\ \mid 167\ 400 - \color{green}{168\ 700}{\mid} &= 1\ 300 \\ \mid 172\ 000 - \color{green}{168\ 700}{\mid} &= 3\ 300 \\ \mid 175\ 000 - \color{green}{168\ 700}{\mid} &= 6\ 300 \\ \mid 178\ 100 - \color{green}{168\ 700}{\mid} &= 9\ 400 \\ \mid 179\ 000 - \color{green}{168\ 700}{\mid} &= 10\ 300 \\ \mid 183\ 000 - \color{green}{168\ 700}{\mid} &= 14\ 300 \end{align}| |
Calculate the deviations from the mean of each of the data. |
|
|EM=\dfrac{12\ 000+9\ 800+\dots+10\ 300+14\ 300}{11}\approx$\ 7\ 927.27| |
Calculate the average of the deviations from the mean (mean deviation). |
See also
For a sample: |s= \sqrt{\dfrac{\sum(x_{i}-\overline{x})^{2}}{n-1}}|
For a population: |\sigma = \sqrt{\dfrac{\sum(x_i-\mu)^2}{N}}|
where
|\sum| a sum of several elements.
|x_i| the |i^\text{th}| distribution value.
|\overline{x}| the sample mean and |\mu,| the average of the population.
|n| the sample size and |N,| the size of the population.
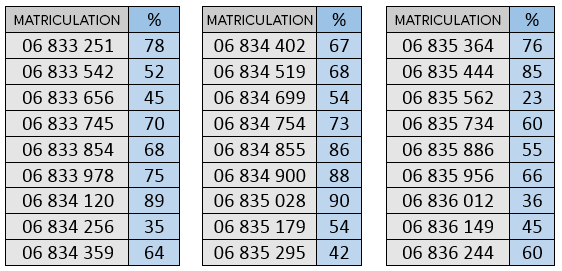
In some university courses, professors assign marks based on exam scores and the standard deviation of the distribution. What is the standard deviation of the following distribution?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
|
|\begin{align}\mu&=\dfrac{78+52+45+\dots+45+60}{27}\\ \mu&\approx63.11\end{align}| |
Determine the mean |(\mu)| of the results according to the population represented by the class. |
|
|\begin{align}(78-63.11)^2&\approx221.71\\ (52-63.11)^2&\approx123.43\\ \vdots\\ (45-63.11)^2&\approx327.97\\ (60-63.11)^2&\approx9.67\end{align}| |
Calculate the square of the all of the data’s deviations from the mean. |
|
|\sqrt{\dfrac{221.71+123.43+\dots+327.97+9.67}{27}}\approx17.61| |
Calculate the square root of the mean of the previous results. |
|
Thus, the standard deviation of the marks of the pupils in this class is approximately |17.61.| |
|
See also
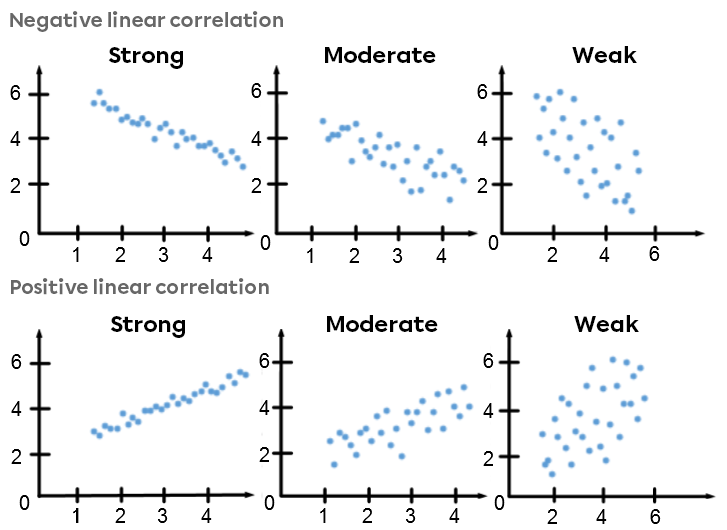
The scatter plot is used to estimate the correlation that exists between two variables. To get a more precise idea of the correlation, you must calculate the correlation coefficient.
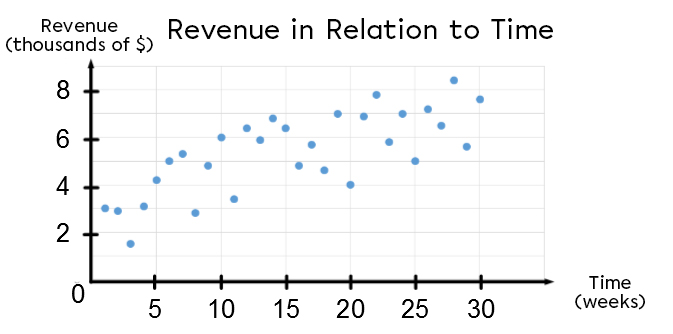
A new company has increased its profits in the last five years and wishes to expand its production centre. However, the owners want to ensure that the economic growth of their company is positive and strongly correlated. To break it all down, here's a count of business income for the past 30 weeks.
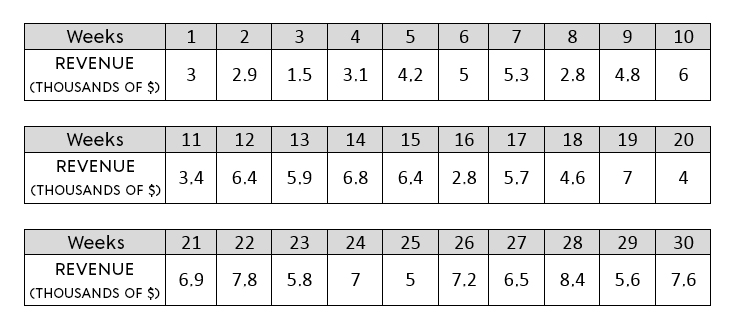
In your opinion, is the economic growth of the company positive and strongly correlated?
-
Plot the scatter plot
-
Compare the scatter plot to those that serve as a reference
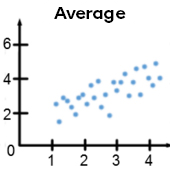
Answer: According to the scatter plot, it can be concluded that the incomes are positively and moderately correlated. Since they are not strongly correlated, it would be best to wait before expanding.
See also
After framing the scatter plot and measuring the length |(L)| and width |(l)| of the rectangle, we have:||r = \pm \left(1 - \dfrac{l}{L}\right)||As for the sign, it depends on the direction of the scatter plots (increasing or decreasing).
This coefficient can also be used to qualify the correlation:
|
Value |r| |
Linear correlation strength |
|---|---|
|
Near |0| |
Zero |
|
Near |\pm0.50| |
Weak |
|
Near |\pm0.75| |
Moderate |
|
Near |\pm0.87| |
Strong |
|
Near |\pm1| |
Very strong |
|
|\pm 1| |
Perfect |
To take stock of the success of students who enroll in adult education, the administration team is interested in the correlation between absenteeism (in hours) and the students’ final grades (in %). To analyze everything properly, they grouped the data into a scatter plot:
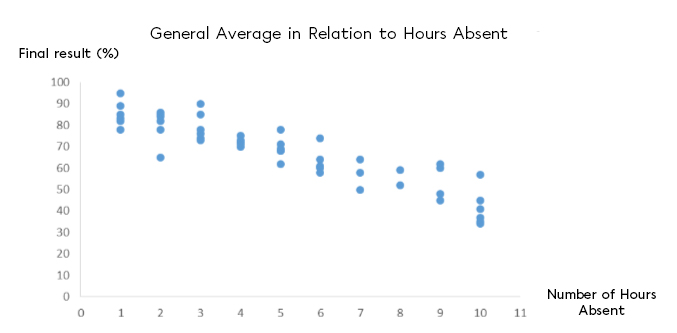
What is the correlation coefficient of this study?
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
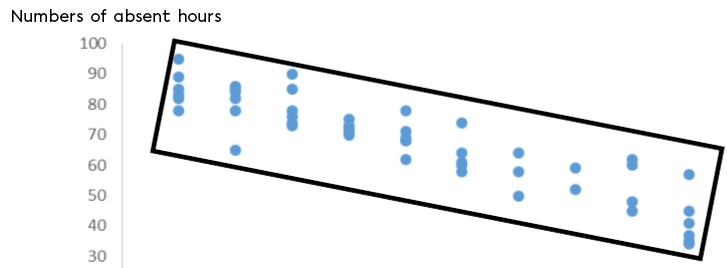 |
Draw a rectangle, as small as possible, to frame the scatter plot. |
| |
Using a ruler, measure the length |(L)| and width |(l)| of the rectangle. In this case, |\color{blue}{L = 13{.}5 \ \text{cm}}| |\color{red}{l = 2{.}8 \ \text{cm}}| |
|
|\begin{align} r &\approx \pm \left(1 - \dfrac{\color{red}{2{.}8}}{\color{blue}{13{.}5}}\right) \\ r &\approx \pm 0{.}79 \end{align}| |
To replace |L| and |l| in the formula |r \approx \pm \left(1 - \dfrac{\color{red}{l}}{\color{blue}{L}}\right).| |
|
|r \approx - 0{.}79| |
Since the rectangle is oriented downward (decreasing), the correlation coefficient is negative. |
|
The correlation coefficient between the number of hours absent and the final result in percentage is approximately |-0.79,| which means it is a moderate negative correlation. |
|
See also
Follow these steps to find the equation of the regression line according to the median-median method:
-
Rearrange in ascending order according to the value of |x.|
-
If possible, divide the points into three equal groups.
-
Calculate the median coordinate |(M_1,M_2,M_3)| of each group.
-
Calculate the mean coordinates of point |(P_1)| of the three midpoints.
-
Calculate the value of slope |(a)| with |M_1| and |M_3.|
-
Calculate the value of the initial value |(b)| with |P_1.|
-
Write the equation of the regression line in the form |y=ax+b.|
Before a new condo tower is built and the landscaping is done, the heights of the surrounding trees are measured to ensure they do not obscure the view for at least 20 years. The following table of values is used to estimate the height of the view:
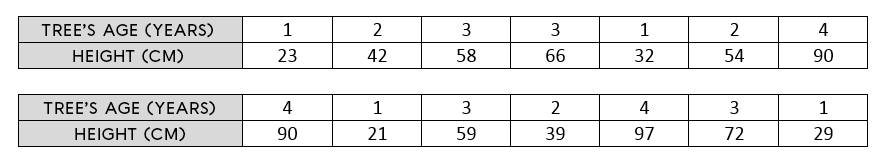
Using this information, determine how high the first balconies should be for the view to remain unobstructed by trees.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
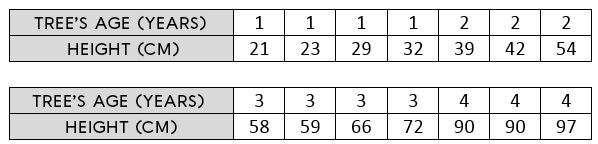 |
Rearrange the points in ascending order according to the value of the independent variable |(x),| taking care not to “undo” the initial ordered pairs. |
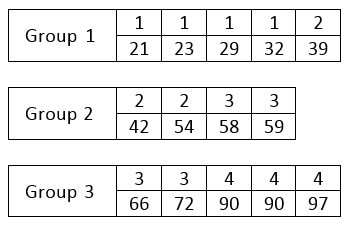 |
Separate the points into three equal groups. If this is not possible, then make sure that the first and the last group have the same amount of data. |
|
|M_1 = (1 . 29)| |M_2 = \left(\dfrac{2+3}{2}, \dfrac{54+58}{2}\right) = (2{.}5 ; 56)| |M_3 = (4, 90)| |
Calculate the median coordinate of each group. |
|
|\begin{align}\color{green}{P}&=\left(\dfrac{1+2.5+4}{3},\dfrac{29+56+90}{3}\right)\\ &=\color{green}{(2.5,58.33)}\end{align}| |
Calculate the mean point by taking the average of the |x|s and |y|s of the three midpoints. |
|
|\color{blue}{a}=\dfrac{\Delta y}{\Delta x}=\dfrac{90-29}{4-1}\approx20.33| |
According to the equation of the regression line |y=\color{blue}{a}x+\color{red}{b},| determine the value of |\color{blue}{a}| by using the points |M_1| and |M_3.| |
|
|\begin{align}y&=\color{blue}{20.33}x+\color{red}{b}\\ \color{green}{58.33}&=\color{blue}{20.33}(\color{green}{2.5})+\color{red}{b}\\ \color{red}{7.503}&=\color{red}{b}\end{align}| Thus, |y=\color{blue}{20.33}x+\color{red}{7.503}| |
Find the value of parameter |\color{red}{b}| by substituting |x| and |y| with the coordinates of point |\color{green}{P}.| |
|
|\begin{align}y&=\color{blue}{20.33}x+\color{red}{7.503}\\ y&=\color{blue}{20.33}(20)+\color{red}{7.503}\\ y&=414.103\end{align}| |
Since we want to know the height of the trees after |20| years, substitute |x| with |20.| |
|
After |20| years, the trees will be about |414.103\ \text{cm}.| Thus, the first balconies must be at a minimum height of |414.103\ \text{cm}.| |
|
Although the situation and data are the same, it is normal that the final answer varies depending on the method used (Median-Median method or Mayer method).
Since these methods are used to estimate rather than to predict outcomes with certainty, there may be a difference between the two outcomes.
See also
To find the equation of the regression line according to Mayer's method, follow these steps:
-
Rearrange the points in ascending order according to the value of |x.|
-
If possible, divide the points into two equal groups.
-
Calculate the mean points |(P_1\text{ and }P_2)| of each group.
-
Use these mean points to find the value of slope |(a)| and the initial value |(b).|
-
Write the equation of the regression line in the form |y=ax+b.|
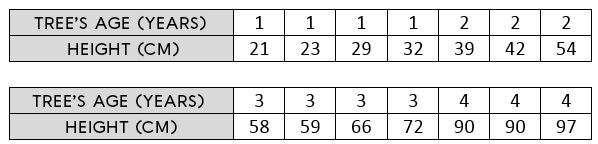
Before a new condo tower is built and the landscaping is done, the height of the surrounding trees are measured to ensure they do not obscure the view for at least 20 years. The following table of values is used to estimate the height of the view:
Using this information, determine how high the first balconies should be for the view to remain unobstructed by trees.
|
CALCULATIONS |
EXPLANATIONS |
|---|---|
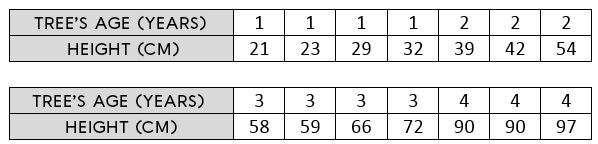 |
First, it is necessary to place the points in ascending order according to the value of the independent variable, x, without undoing the initial ordered pairs. |
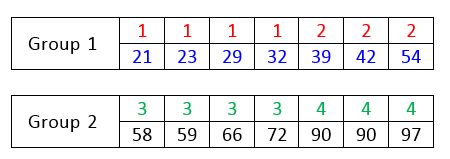 |
If possible, separate the distribution into two equal groups. |
|
|\begin{align}P_1&=\left(\dfrac{\color{red}{1+1+\ldots+2+2}}{7},\dfrac{\color{blue}{21+23+\ldots+42+54}}{7}\right)\\ &\approx(\color{red}{1.43},\color{blue}{34.29})\\\\ P_2&=\left(\dfrac{\color{green}{3+3+\ldots+4+4}}{7},\dfrac{58+59+\ldots+90+97}{7}\right)\\ &\approx(\color{green}{3.43},76)\end{align}| |
Calculate the mean points of each group. |
|
|a=\dfrac{\Delta y}{\Delta x}=\dfrac{76-\color{blue}{34.29}}{\color{green}{3.43}-\color{red}{1.43}}\approx20.86| Therefore, |y=20.86x+b.| By substituting the coordinates of |P_1:|| ||\begin{align}y&=20.86x+b\\ 34.29&=20.86(1.43)+b\\ 34.29&=29.83+b\\ 4.46&=b\end{align}|| Therefore, |y=20.86x+4.46.| |
Find the equation of the regression line of the form |y=ax+b| using points |P_1| and |P_2.| |
|
||\begin{align}y&=20.86x+4.46\\ y&=20.86(20)+4.46\\ y&=417.2+4.46\\ y&=421.66\end{align}|| |
Since we want to know the height of the trees after |20| years, substitute |x| with |20.| |
|
After |20| years, the trees will be about |421.66\ \text{cm}.| Thus, the first balconies must be a minimum height of |421.66\ \text{cm}.| |
|
Although the situation and data are the same, it is normal that the final answer varies depending on the method used (Median-Median method or Mayer method).
Since these methods are used to estimate rather than to predict outcomes with certainty, there may be a difference between the two outcomes.